filmov
tv
Functions of operators in quantum mechanics

Показать описание
📚 Many important phenomena in quantum mechanics are described by functions of operators. For example, spatial translations are given by an exponential function of the momentum operator, and time evolution is given by an exponential function of the Hamiltonian. In this video we explain how to construct the function of an operator, and explore what their properties are.
⏮️ BACKGROUND
⏭️ WHAT NEXT?
📖 READ MORE
If you are interested in the mathematics behind the Baker-Campbell-Hausdorff formula, further details can be found in the following links:
~
Director and writer: BM
Producer and designer: MC
Functions of operators in quantum mechanics
Position and Momentum Operators in Quantum Mechanics
Quantum Operators
Ever heard of Quantum Operators and Commutators? (Explained for Beginners)!
Quantum Chemistry 3.2 - Operators
Before You Start On Quantum Mechanics, Learn This
Quantum Wavefunction | Quantum physics | Physics | Khan Academy
Wavefunction Properties, Normalization, and Expectation Values
Reverse Physics (Live): planning Quantum Mechanics
The Quantum Wavefunction Explained
Quantum Chemistry 3.3 - Eigenvalues and Eigenfunctions
What is a Hamiltonian? Quantum Jargon Explained
The SECOND Most Important Equation in Quantum Mechanics: Eigenvalue Equation Explained for BEGINNERS
Ch 14: Where does the momentum operator come from? | Maths of Quantum Mechanics
Quantum Chemistry -1 | Operators in quantum mechanics
The translation operator in quantum mechanics
Eigenfunctions and Eigenvalues
Understanding Quantum Mechanics #4: It's not so difficult!
Hermitian Operator in Quantum Mechanics | Explained with solved example | Quantum Chemistry
Quantum Wave Function Visualization
Wave Functions in Quantum Mechanics: The SIMPLE Explanation | Quantum Mechanics... But Quickly
Why Momentum in Quantum Physics is Complex
Eigenvalues and eigenstates in quantum mechanics
The parity operator in quantum mechanics
Комментарии
 0:19:28
0:19:28
 0:26:05
0:26:05
 0:21:46
0:21:46
 0:13:47
0:13:47
 0:04:55
0:04:55
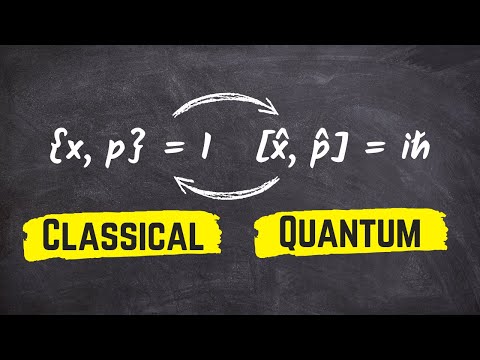 0:11:05
0:11:05
 0:10:11
0:10:11
 0:23:16
0:23:16
 1:38:46
1:38:46
 0:05:40
0:05:40
 0:05:09
0:05:09
 0:00:54
0:00:54
 0:11:25
0:11:25
 0:17:43
0:17:43
 0:21:36
0:21:36
 0:09:47
0:09:47
 0:02:09
0:02:09
 0:08:05
0:08:05
 0:09:27
0:09:27
 0:11:23
0:11:23
 0:09:29
0:09:29
 0:09:26
0:09:26
 0:17:51
0:17:51
 0:19:27
0:19:27