Why Momentum in Quantum Physics is Complex

In classical physics, we are used to calculating an object's momentum by multiplying its mass by its velocity. But how do we deal with momentum in Quantum Mechanics, where we commonly deal with wave functions?

A wave function is a mathematical function that contains all the information we can know about any system we're studying. For example, if our system is a single electron, the wave function can be used to calculate the probability of finding the electron at different positions in space, or with different values of momentum.

It's only when we make a measurement on the particle, that we cause a collapse in the wave function, and thus know a certain value for the particle's momentum. So a question we can ask is, how do we mathematically deal with the idea of making a measurement in quantum mechanics?

Since the system is described by the wave function, we apply a "measurement operator" to the wave function, which is the real-life equivalent of making a measurement. Mathematically, this is like applying a matrix to a vector (where the matrix is the operator, and the vector is the wave function). On the right hand side of the equation, we get the "eigenvalue", which is the actual measured value that we find as a result of our experiment. This equation is described as the eigenvalue equation.

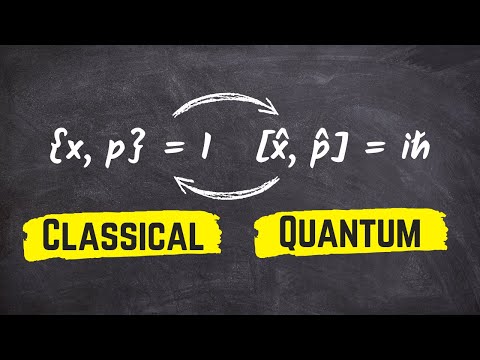
But what does the momentum operator, that we use in the eigenvalue equation, actually look like? Does it look similar to mass x velocity, which is what momentum looks like in classical physics? The answer is no - the momentum operator is more complex, with the imaginary number, reduced Planck constant, and a partial derivative with respect to x as part of the expression.

The reason for this, is that the operator is derived by considering a "building block" wave function (which is sinusoidal). This can be written in terms of the exponential function, and the position and momentum of the particle. This building block function can also be used to break down almost any other function we deal with. When we differentiate this building block wave function, we find that the result is equal to the momentum, p, of the particle, multiplied by the original wave function and some other factors. Thus if we rearrange this, we find the momentum operator expression.

It's worth noting though that the logic behind momentum is still the same - the conservation of momentum still applies, and the idea is still that it is the mass of the object multiplied by its velocity.

Many of you have asked about the stuff I use to make my videos, so I'm posting some affiliate links here! I make a small commission if you make a purchase through these links.

Thanks so much for watching - please do check out my socials here:
Instagram - @parthvlogs
Music Chanel - Parth G's Shenanigans

Timestamps:
0:00 - Classical Momentum (p = mv) and Wave Functions
2:36 - Mathematically Encoding Momentum in Quantum Mechanics
3:44 - What Does the Momentum Measurement Operator Look Like, and Where Does it Come From?
5:33 - Breaking Down Complex Wave Functions into Simple Building Blocks
6:17 - Deriving the Momentum Operator by Differentiating the Wave Function Building Block

[ParthGChannel]

Also, as always, let me know what other physics topics you'd like me to cover in future videos :)

[AndrewDotsonvideos]

Maybe this is what you were saving for a future video, but the operator being complex (specifically Hermitian) is required so that its eigenvalues are guaranteed to be real. So even though the operator is complex, what's measured in experiment is always real:) Complex "observables" confused me so much in undergrad until that connection was made lol. Nice job as always!

[qubex]

I found your overview to be thorough and it was very thoughtfully presented. I do appreciate when explainers take time to “unpack the math° and strip away the sometimes abstruse notations to show “what’s really going on” at the level that one could then sit down and actually perform the calculation with Calculus II level knowledge. Thank-you.

[abdulllllahhh]

You are a talented teacher, you speak so elegantly and manage to make this stuff sound so simple. You just earned yourself a subscriber and I will definitely be recommending you to my friends!

[theverynoob]

I've watched a lot of quantum physics related content. I've been engaged in quantum physics courses. I had never seen an explanation that was so easy to follow but still bringing all the info I needed to not feel like something was missing.

[mann]

also at 8:20 there is a small mistake, you have written p = -ihd/dt, the derivative is supposed to be wrt x!

[carlthorellstein]

Still can't believe his channel isn't called Parth to Knowledge...

[johncgibson]

I was a EE student 20 years ago. The imaginary part i is most certainly the phase parameter of the particle or device. Capacitors and inductors also have the i component as their parameter when you want to calculate the circuit's behavior in a phasor diagram.

[account]

When you apply an operator to an eigenstate, you end up multiplying the eigenstate by the eigenvalue, this is not the same as what happens when you make a measurement: when you make a measurement the general state collapses randomly to an eigenstate with a probability given by |c|^2 where c is the coefficient of that eigenstate in the original state - applying an operator and making a measurement are not the same thing :)

Operators give us a spectrum of possible eigenvalues for any given observable, and they also provide us with a means of calculating an expectation value for any given observable

[ugoamaldi]

Sir, this is a first-class educational video and you explain with straight and precise language extremely difficult concepts. Thank you!

[Waterfront]

You can say I suppose that In QM momentum is a term in the exponent, to get the momentum out of the exponent you have to take the differential with respect to distance and multiply with i*hbar to remove unwanted factors. The wave function will not change as it is an exponential, therefore the wave function is on both sides of the equation.

[zray]

In the Heisenberg picture, the momentum is the mass times the velocity operator (time derivative of the position operator).

[MasterHigure]

2:00 It is, as I remember it, theoretically impossible to have totally precise measurements of observables that have a continuum of possible outcomes, like position usually does. So even after measuring the particle, we only get a somewhat certain result back, and the wave function collapses to a more localized, but not single-positioned wave function.

[Lucky]

6:17
This is giving me flashbacks to Fourier series problems in my signals and systems class last year. Fourier series (which are how we write complicated wave functions as sums of simpler wave functions) are awesome as a concept and super useful, but computing them by hand is a *ginormous* pain in the butt. Thanks heavens for Matlab and wolfram alpha.

[georgerevell]

wow thats great thanks! Its amazing how easy QM can be sometimes, abstract and complex but not necessarily too complicated.

[mauroviscardi]

Great lesson, Parth G. Just a comment: last formula is really correct (derivative over X, not over time)? Thank you for all you excellent support.

[palacinka]

So good! I wish you would be giving lectures at my Uni

[rsbenari]

Very nicely done. Thanks. It would be helpful to get your brief take on why and how the plane wave works -- aside from the mathematical convenience of its partials fitting the needs of the Schrödinger Equation (as you hint at here). Thanks again. As in your other vids, your explanation here is crystal.

[skg]

Thank you! I'm studying at the university now and finaly got it! 🙌

[mistersilly]

this is the clear explanation i've been waiting for. well, one of them. i've seen lots of descriptions of squaring the wave function to get a probability, but not a concrete connection between the probability of a thing and the wave function of a thing. i'm still digesting this, but when you introduced the system & wavefunction, i'd have liked to see a few examples of different systems before you focused on a particle's momentum, so i had a better picture of psi's general role in describing a system's evolution ... psi describes this variable in this system when these variables are held constant. very very good though

i always wondered why they switched notation for partial derivatives. if you have extra independant variables, dy/dx could only make sense if you treat the other variables as constant ... you're specifically talking about sliding infinitessimally in the x direction only, whether you have one or many iv's