Solving Math Equations but they keep getting HARDER | jensenmath.ca

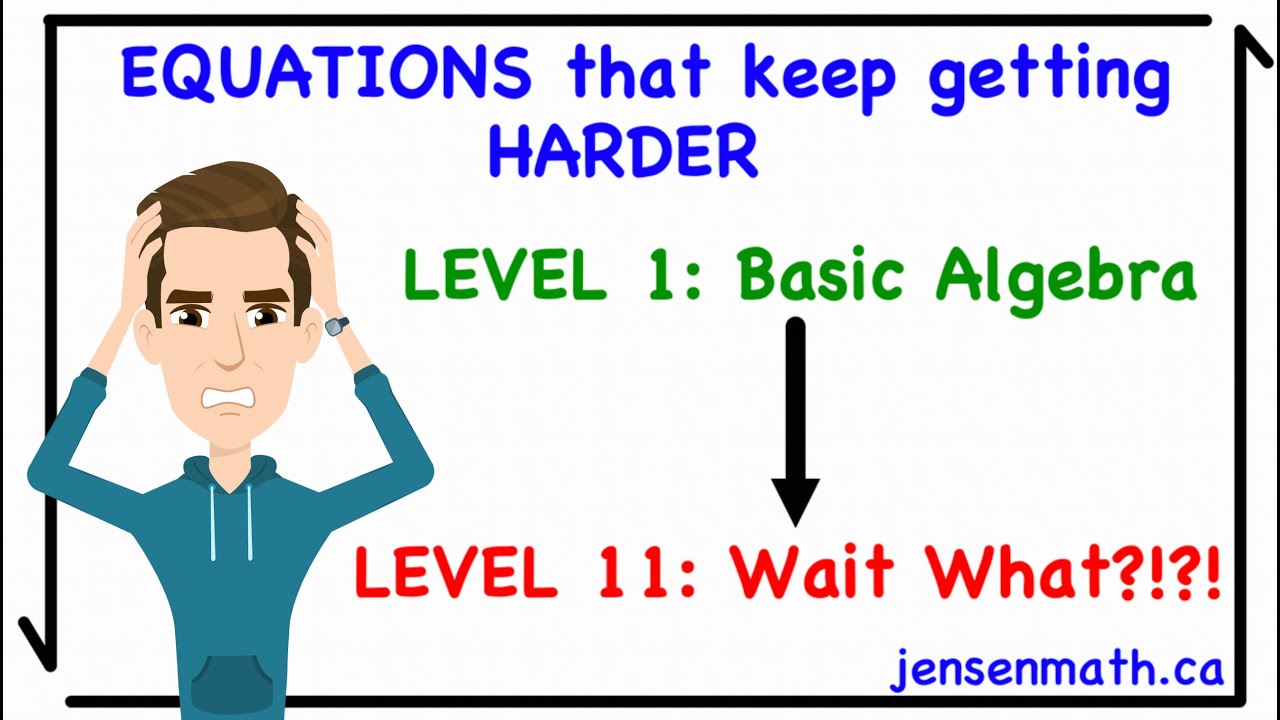
Welcome to JensenMath! In this video, we'll dive into 11 high school math equations, starting from fundamental algebraic expressions and progressing to more advanced topics like quadratic equations, exponentials, logarithms, and trigonometry. Whether you're a student looking to reinforce your understanding or a curious mind eager to explore the beauty of mathematics, this video is for you!

Throughout the video, we'll provide step-by-step explanations and examples to help you grasp each concept. Remember, math is not just about memorizing formulas; it's about understanding the underlying principles and applying them to solve problems.

0:00 - level 1 equation
1:06 - level 2 equation
2:22 - level 3 equation
3:33 - level 4 equation
5:14 - level 5 equation
6:08 - level 6 equation
8:33 - level 7 equation
10:55 - level 8 equation
13:57 - level 9 equation
16:43 - level 10 equation
20:55 - level 11 equation

[Taric]

I thought you were going to end with complex analysis. These were all pre-calculus.

[azuarc]

I must not be the target audience for this video because I thought these were all really basic. My pre-calc students should be able to solve all of these. I was waiting to be actually challenged.

[janvasicek]

I was waiting for differential equations. But this video was awesome to watch, you have a nice voice😊.

[Booskop.]

I've been out of math class for 15 years and I managed to solve the first 3 equations. Consider me a happy man.
Edit: I was glad when you noticed the missing bracket and corrected it.

[jakejake]

I actually really enjoyed this type of video. I am currently in Grade 12 (finished advanced functions) and I was able to get everything correct except for #10 because I forgot the identities. Great vid I really enjoyed this!

[OculusVeri]

Nice
So I paused the video and just ended level 1 after 2 hours of calculating
Looking forward for level 2
So far so good

[riccardofroz]

Level 8 I would have done it without "test":
x^4-x^3-11x^2+9x+18=0
Split -11x^2 in -2x^2 and -9x^2 and manipulate the signs to have the following
(x^4-x^3-2x^2) - (9x^2-9x-18)=0
Factor x^2 on the left parenthesis group and 9 on the right:

(x^2-x-2)x^2-9(x^2-x-2)=0
(x^2-9)(x^2-x-2)=0
x=+-3
x=(1+-sqrt(9))/2=-1, 2

[charlescrawford]

Saving this for my 10 year old. He'd love this.

[King-sdvg]

Really cool video, this is great for someone like me who missed out early into math, but I'm really fascinated by it now and trying to learn! I've noticed a lot of the time people do anything in their power to not use the quadratic formula I wonder why? For me it's been 10x easier every time I see a quadratic just throw everything into the formula and most of the time it works out nicely.

[mrgamepigeon]

I struggled massively with the level 6 equation. I did it but I went throught like 20 steps. All that for you to show me that I could've taken the log of both sides.

[papapapalima_scotland]

Great practice. Got all but 9 and 10 right. 9 I made a silly mistake, but I own it. 10 I was completely out of my depth, would need a refresher in trig. 1 to 8 and 10 were enjoyable but nothing too difficult.

[inconspicuous]

As a 9th grader (currently halfway through algebra 2) I was pleasantly surprised that I could do the first 6 with ease

[douglasstrother]

Log rolling! Channel your inner Lumberjack! These are all pretty fun.

[bjornfeuerbacher]

Another way to do equation 6: Write it as 4^(3x) times 4^(-1) = 5^x times 5^2, bring every factor with x to the left and every factor with x to the right, so 4^(3x)/5^x = 5^2 times 4, which easily simplifies to (64/5)^x = 100. If you have a modern calculator which can do logs to every basis, now you just have to take the log to the basis 64/5 and you are done; otherwise use log to the basis 10 and use log(64/5)^x = x times log(64/15).

[rubennavarrobonanad]

Thought this video would have dif. Eqs. It didn't, but still great vid!

[CaulolProductions]

me at the first 4 questions: why is bro taking the hard routes?

[Silver_crap]

Basically you solved different kind of quadratic equations...nice👌👌.

[JeftherGabriel]

Wow, I didn't know it was possible to factor quadratic expressions in this way. In school, I only learned to use the resolution formula. At least I can handle exponentials and logarithms; I'm not sure about trigonometrics yet.

[yourcolotisdifferent]

i found a better last problem prove or disprove the Riemann hypothesis

[ElectricGamer_YT]

The advanced trig way to write equation #7 is:
x_1=7π6+2πn | X_2=11π6+2πn
Assuming _ means "subscript" and n is an integer.
It expresses all of the infinite solutions.
Obviously there isn't infinite solutions in-between 0 and 2π, so in a word problem for less advanced trig, then that makes more sense
Also, I was about to comment that x=2-√14 undefined, but nevermind