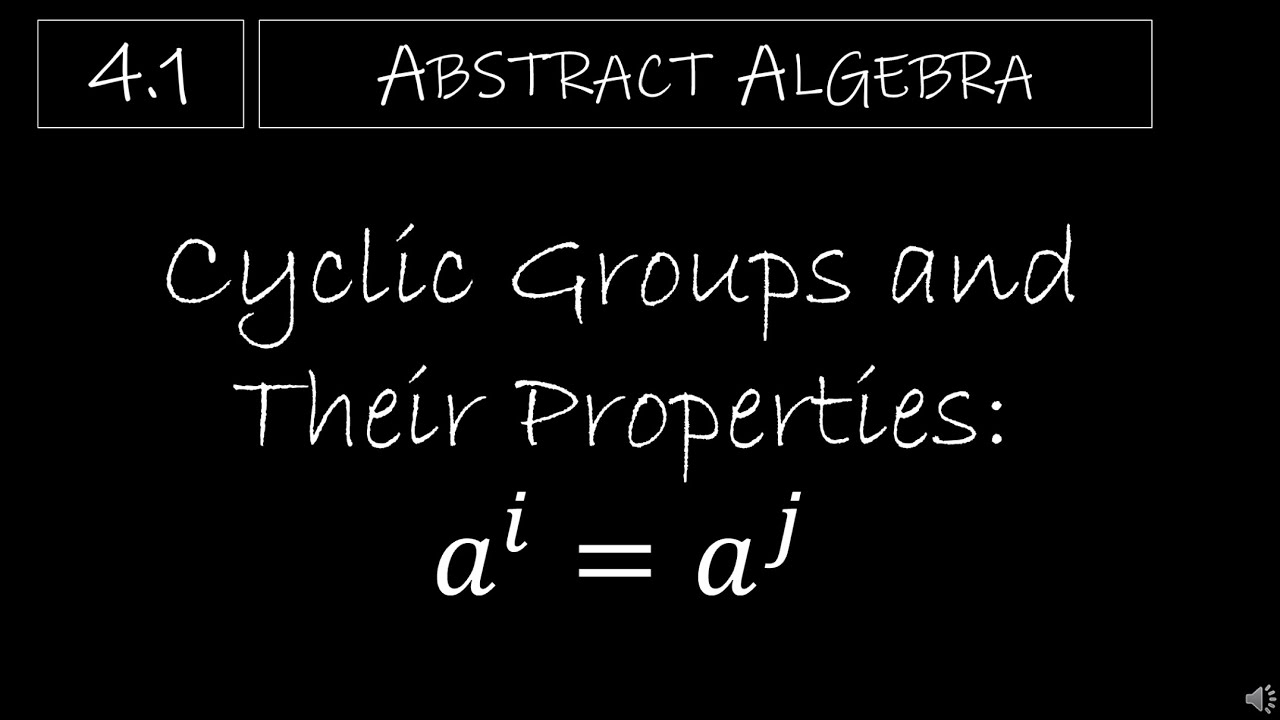
Abstract Algebra - 4.1 Cyclic Groups and Their Properties (𝑎^𝑖=𝑎^𝑗)

We took a brief look at cyclic subgroups in our last chapter. In this chapter, we will review the definition of a cyclic group and look at a few examples to be sure we understand the concept. We then want to take a look at one of the two theorems we have related to cyclic groups and their several corollaries. I've done my best to provide an example of when each corollary is used. If you have others, please feel free to drop them in the comments.

Video Chapters:
Intro 0:00
What is a Cyclic Group? 0:27
The Property a^i=a^j 5:40
Consequences of the Property 10:47
Applications of the Property 13:18
Up Next 16:35

This playlist follows Gallian text, Contemporary Abstract Algebra, 9e.

[shiva_kondapalli]

In the a^i = a^j property, around the 10 minutes 14 seconds timestamp, should it not be 5^2 = 5^6. 6-2=4 right? A quick computation shows 10 mod 20 = 30 mod 20 = 10. Or am I missing something here?

[SummiyaMumtaz]

In case we have t=2+3w as w^2=1 then what the value is t^2 ? It has mod 67 at t^66=(1, 0). Waiting for your response

[CateJones-efox]

Shouldn't Z_20 have a 0 as an element? (8:36)