Permutation Groups and Symmetric Groups | Abstract Algebra

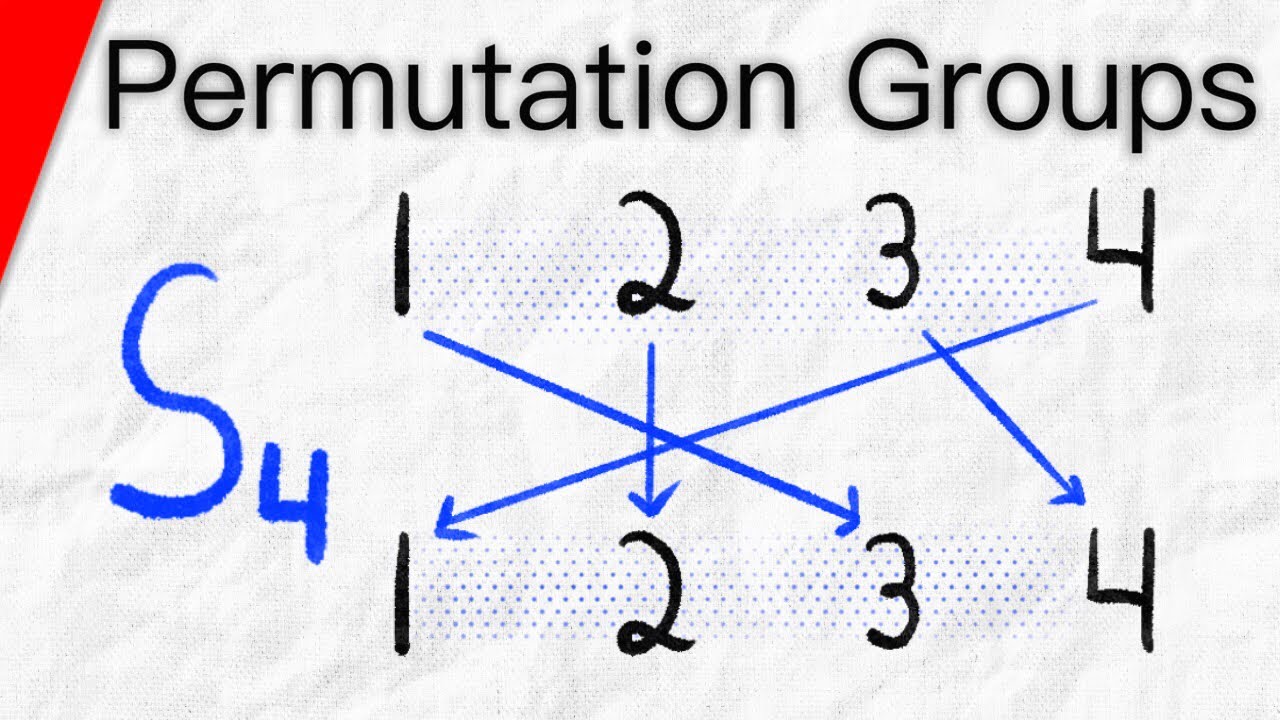
We introduce permutation groups and symmetric groups. We cover some permutation notation, composition of permutations, composition of functions in general, and prove that the permutations of a set make a group (with certain details omitted). #abstractalgebra #grouptheory

We will see the Cayley table for the symmetric group S3, and look at some inverse permutations. We also cover the definition of a permutation, which is a bijection from a set to itself.

A proof that compositions of bijections are bijective in two parts:

★DONATE★

Follow Wrath of Math on...

[WrathofMath]

Support this course by joining Wrath of Math to access exclusive and early videos, original music, plus lecture notes at the premium tier!

[Adecto]

In the first 3 minutes I understood everything better than after 4 hours of lectures and hours of watching other YouTube videos. Thank you so much!!!

[areaxi]

These are some of the best math videos on YT. Other math YT-ers should take note. Aside from good content: 1. high resolution, 2. good mic/clear audio, 3. clear annotations. Not sure why others can't just buy a good microphone and put it by their mouth rather than putting it across the room, in their waving hand, in the kitchen sink, not plugging it in, handing it to their crying baby....

[justinpalmer]

God send, my prof did the composition of groups without explaining what he was doing at all and I was so lost, video helped alot :)

[namelessflower]

Thank you! You made everything so easy to understand.

[misterentername]

These are amazing explanations, clear and mentions everything! Thank you, this deserves more views.

[turefu]

Thanks a lot for this explanation. It helps me with a problem troubling for two days.

[benallen]

alpha is its own inverse because it is a transposition. i.e., any 2 cycle is its own inverse. The cycle decomposition of alpha is (1 2)(3) = (1 2). Hence, (1 2) is its own inverse as is any other transposition. Hope this helps anyone else studying for their abstract algebra final!

[letsimage]

thank you a lot for this explanation, now this topic is much clear for me!

[homqua]

Thanks so much, your explanation is clear 💛

[estherlevenson]

Thank you so much. I'd like to know more about the relationship between symmetry groups and permutation groups.

[shwetabhsingh]

Lovely video, great conceptual clarity, this seems to be missing from a lot of physics lectures which involve group theory. Thanks.

[FMTanmayChopra]

Wonderful man, really clear explanations.

[prianborah]

Hi, this is a fantastic video. Could you make a video on A4 discrete symmetry group and its character table and what its irreducible representations like 1, 1', 1'', and 3 means, and also its multiplication table??

[tirtahadith]

Done (but i think i need to rewatch it again multiple times

[mzarinchang]

Thats so cool....I thought that if a=a^(-1) then a must be the this example showed that its not neccessarily

[jaydevdeshmukh]

In your table at the end of the video, Why does Beta and Beta lead to delta? Should it also not have identity there? Same goes with Delta and Delta which is giving Beta.

[xibbit]

my teachers making us do fuunction composition from left to right because he prefers it but no one uses that... its so annoying and confusing :(

[ismailadekunle]

Can you do a full tutorial on Groups 😢❤

[ma.lourdezs]

Hi! Can you explain the general associative law?