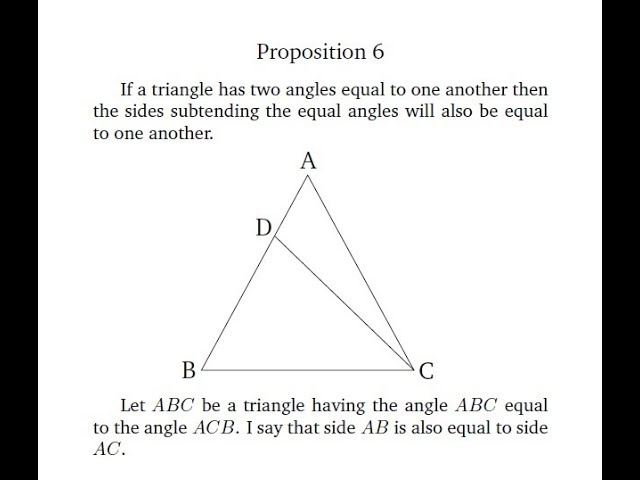
Euclid's first proof by reductio ad absurdum Book I Proposition 6

The video explains the first so called "proof by contradiction" even though the proof is actually based on the absurdity that arises from the initial proposition.

To "assume" means to "suppose to be the case without proof". It does not mean we "believe" it to be true or false as for this matter. The statements which follow "pretend" the initial statement is true.

The IF-THEN deductive logic in mathematics has NOTHING to do with the bullshit of propositional logic which relies on the nonsensical ZFC axioms. For example,

"If the sky is pink then the sun is green" is a true statement in propositional logic. However, in deductive logic in mathematics, the statement IF X THEN Y requires that for Y to be TRUE, X must be TRUE. There is a relation between X and Y.

There are no axioms or postulates in mathematics. Beliefs, decrees and rules have no place in rational thought, only hard, cold (deductive) logic and facts.
Link to applet used in this video:

Link to my free eBook: