filmov
tv
Related Rates in Calculus
Показать описание
Now that we understand differentiation, it's time to learn about all the amazing things we can do with it! First up is related rates. Sometimes the rates at which two parameters change are related to one another by some equation. With our newfound understanding of implicit differentiation, it's not too hard to find this precise relationship so that we can do important scientific calculations. Check it out!
Check out "Is This Wi-Fi Organic?", my book on disarming pseudoscience!
Check out "Is This Wi-Fi Organic?", my book on disarming pseudoscience!
Related Rates in Calculus
Introduction to Related Rates
Related Rates - The Shadow Problem
Calculus AB/BC – 4.5 Solving Related Rates Problems
Calculus 1: Related Rates (Video #17) | Math with Professor V
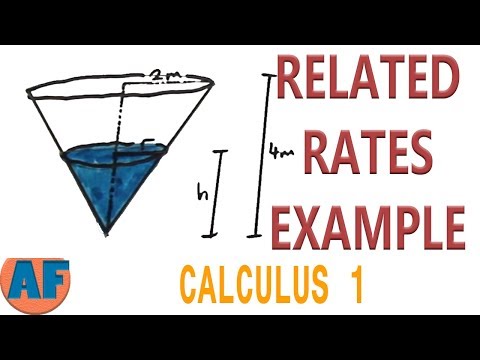
Related rates: water pouring into a cone | AP Calculus AB | Khan Academy
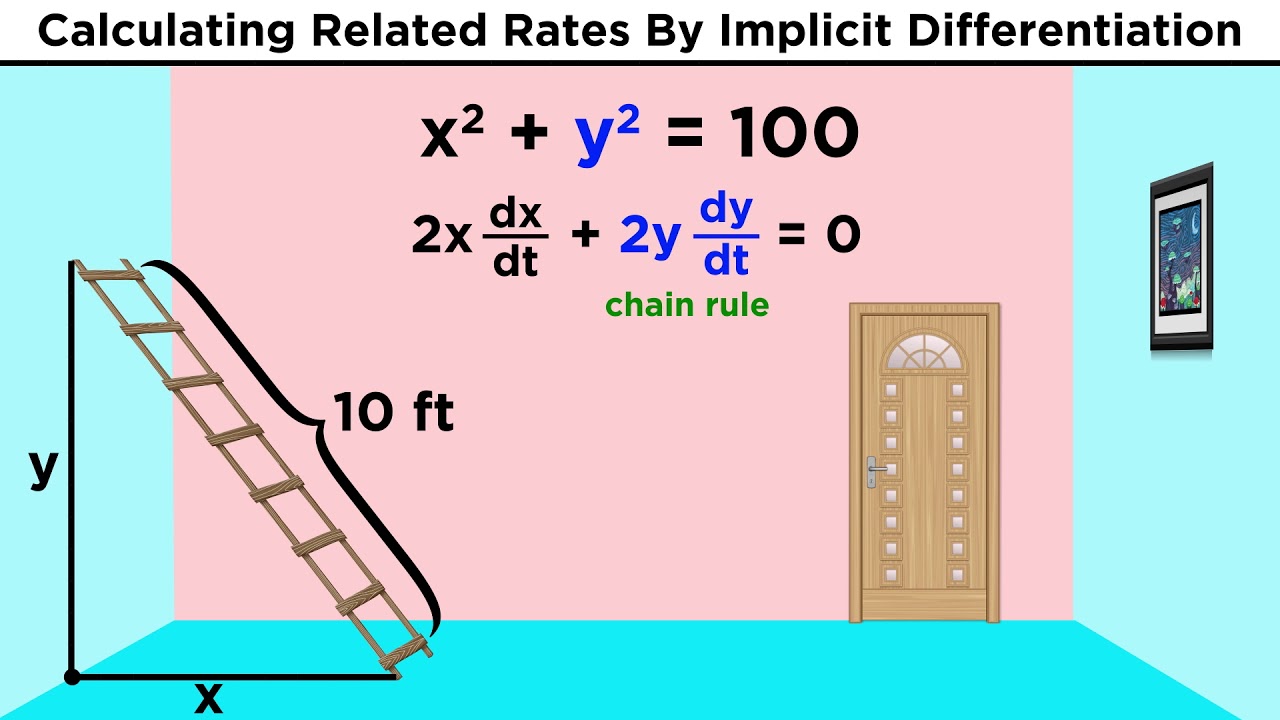
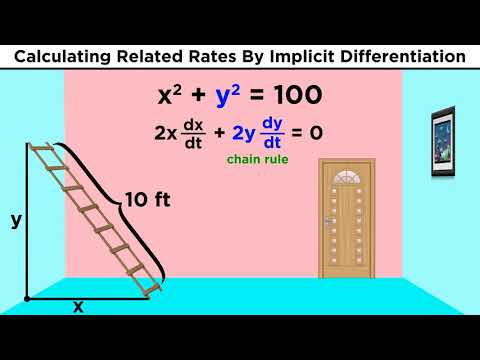
Related Rates - The Ladder Problem
related rates: the streetlight and shadow problem
Related rates intro | Applications of derivatives | AP Calculus AB | Khan Academy
Calculus AB/BC – 4.4 Introduction to Related Rates
Related Rates | Calculus 1 | Math with Professor V
Related Rates of Change: Overall Strategy
Related Rates - Inflated Balloon & Melting Snowball Problem - Surface Area & Volume
Introduction to Related Rates
Calculus 3.9 Related Rates
Related Rates: A Calculus Problem
Related rates: Falling ladder | Applications of derivatives | AP Calculus AB | Khan Academy
Related Rates - Simplified
Related rates: Approaching cars | Applications of derivatives | AP Calculus AB | Khan Academy
Hard Calculus Problems Made EASY!! (Related Rates)
Business Calculus: Related Rates example 1
Related Rates - Area of a Triangle
Step by Step Method of Solving Related Rates Problems - Conical Example
Related Rates Example | Calculus 1 | Math with Professor V
Комментарии
 0:08:53
0:08:53
 0:10:32
0:10:32
 0:10:52
0:10:52
 0:12:17
0:12:17
 0:29:04
0:29:04
 0:11:32
0:11:32
 0:13:52
0:13:52
 0:04:59
0:04:59
 0:07:43
0:07:43
 0:09:14
0:09:14
 0:13:51
0:13:51
 0:07:37
0:07:37
 0:10:53
0:10:53
 0:04:23
0:04:23
 0:25:19
0:25:19
 0:03:51
0:03:51
 0:05:49
0:05:49
 0:09:21
0:09:21
 0:06:53
0:06:53
 0:01:00
0:01:00
 0:05:27
0:05:27
 0:10:11
0:10:11
 0:09:42
0:09:42
 0:07:36
0:07:36