filmov
tv
Discrete Math 2 - Tutorial 16 - Generating Functions

Показать описание
First look at Generating Functions; another way of counting objects.
Please comment rate and subscribe.
for latest updates :
Please comment rate and subscribe.
for latest updates :
Discrete Math 2 - Tutorial 1 - Rule of Sum and Product
5 Tips to Crush Discrete Math (From a TA)
Discrete Math 2 - Tutorial 2 - Permutation Part 1
Discrete Mathematics for Computer Science
Truth Table Tutorial - Discrete Mathematics Logic
Discrete Math 2-Tutorial 15- Generalize Inclusion/Exlusion
INTRODUCTION to SET THEORY - DISCRETE MATHEMATICS
Discrete Math 2 - Tutorial 17 - More Techniques
Discrete Mathematics Chapter -01 [ Mathematical Reasoning ] Tutorial - 02
Discrete Math Proofs in 22 Minutes (5 Types, 9 Examples)
10 Math Concepts for Programmers
Discrete Math 2 - Tutorial 16 - Generating Functions
FUNCTIONS - DISCRETE MATHEMATICS
Discrete Math 2 - Tutorial 18 - Finding Coefficients
Discrete Math 2 - Tutorial 24 - Linear Recurrence Relations
WGU Discrete Math 2 Tips and Tricks - C960 Passed in 3 Weeks!
Proof by Mathematical Induction - How to do a Mathematical Induction Proof ( Example 1 )
INJECTIVE, SURJECTIVE, and BIJECTIVE FUNCTIONS - DISCRETE MATHEMATICS
RECURRENCE RELATIONS - DISCRETE MATHEMATICS
WGU Discrete Math 2 Tips and Tricks | Passed on 1st Attempt!
INTRODUCTION to PROPOSITIONAL LOGIC - DISCRETE MATHEMATICS
Discrete Mathematics (Full Course)
Discrete Math 2 - Tutorial 23 - Partition of Integers Ex.
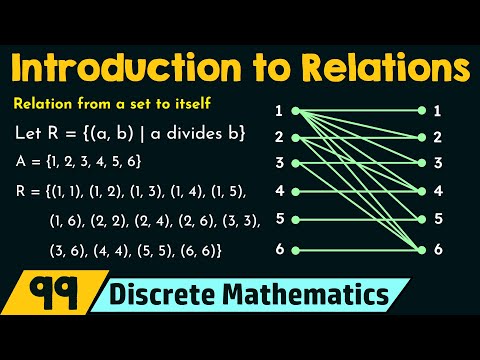
Introduction to Relations
Комментарии
 0:06:01
0:06:01
 0:11:57
0:11:57
 0:08:46
0:08:46
 0:03:15
0:03:15
 0:07:51
0:07:51
 0:09:57
0:09:57
 0:16:38
0:16:38
 0:11:04
0:11:04
 0:13:00
0:13:00
 0:22:09
0:22:09
 0:09:32
0:09:32
 0:10:17
0:10:17
 0:14:40
0:14:40
 0:06:50
0:06:50
 0:10:36
0:10:36
 0:10:08
0:10:08
 0:07:32
0:07:32
 0:17:15
0:17:15
 0:15:25
0:15:25
 0:09:58
0:09:58
 0:11:02
0:11:02
 6:08:01
6:08:01
 0:05:40
0:05:40
 0:07:39
0:07:39