filmov
tv
Linear Independence of Functions & The Wronskian

Показать описание
What is the analog of Linear Independence for functions? If this was just vectors linear independence would mean the only way you can take a linear combination that adds up to zero is the trivial linear combination with all the coefficients being zero. Same for functions. However, we do have one extra tool called the Wronskian, which is a nifty little determinant that we can use to create a quick test for linear dependence of a set of functions. The connection to differential equations will be that we will be looking for a fundamental set of solutions which will have to be linearly independent, more on that in the next video.
0:00 2D case
1:22 Geometric Picture
2:27 Linear Independence
5:54 The Wronskian
8:37 Example
OTHER COURSE PLAYLISTS:
OTHER PLAYLISTS:
► Learning Math Series
►Cool Math Series:
BECOME A MEMBER:
MATH BOOKS & MERCH I LOVE:
SOCIALS:
Linear Independence of Functions & The Wronskian
Determine if the Functions are Linearly Independent or Linearly Dependent
Linear Independence
Linear Dependent Functions
Check for Linear Independence (3 functions, using definition)
Check for Linear Dependence (of 2 functions)
Check for Linear Independence (2 functions)
Introduction to linear independence | Vectors and spaces | Linear Algebra | Khan Academy
Open session - Week 11 and 12
Prove that the Functions sin(x), cos(x), and x are Linearly Independent over the set of Real Numbers
Linearly Independent Functions Examples (Wronskian)
linear Independence of functions
(2.3.104) Determine if 4 Functions Are Linearly Independent or Linearly Dependent
Check for Linear Independence (3 functions, using Wronskian)
Linear Algebra 04: Linearly independent functions (Ch2 Pr2)
How to Determine if Functions are Linearly Independent or Dependent using the Definition
Determining if Functions are Linearly Independent or Dependent using the Definition
The Vector Space of Polynomials: Span, Linear Independence, and Basis
Determine if the Functions are Linearly Independent or Linearly Dependent using the Definition
Linear Dependence Vs Linear Independence For Two Functions Visually Illustrated
Linear Independent Functions - The Wronskian
Sec 4.3 Linear independent functions
Linear Dependence/Independence and Wronskian
How to show that 4 trig functions are Linearly Independent by using the definition of LD
Комментарии
 0:09:59
0:09:59
 0:04:29
0:04:29
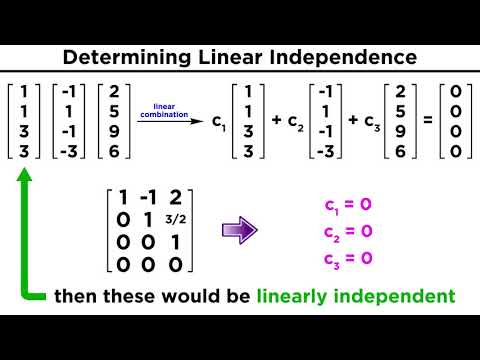 0:12:56
0:12:56
 0:08:38
0:08:38
 0:06:50
0:06:50
 0:07:09
0:07:09
 0:04:07
0:04:07
 0:15:46
0:15:46
 2:11:17
2:11:17
 0:05:42
0:05:42
 0:35:05
0:35:05
 0:03:40
0:03:40
 0:03:43
0:03:43
 0:08:16
0:08:16
 0:04:51
0:04:51
 0:07:07
0:07:07
 0:03:21
0:03:21
 0:12:50
0:12:50
 0:06:12
0:06:12
 0:05:05
0:05:05
 0:12:11
0:12:11
 0:06:21
0:06:21
 0:09:33
0:09:33
 0:06:00
0:06:00