filmov
tv
Solving A Nice log Equation

Показать описание
🤩 Hello everyone, I'm very excited to bring you SyberMath Shorts!
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #counting #geometry #numbertheory #calculus
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #counting #geometry #numbertheory #calculus
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Solving Logarithmic Equations
Solving Log Equation
Solving Exponential and Logarithmic Equations
Solving A Nice Logarithmic Equation
solving a logarithmic equation with different bases
Solving An Interesting Log Equation | Math Olympiads
Solving An Exponential Log Equation | Math Olympiads
Solving logarithmic equations | Exponential and logarithmic functions | Algebra II | Khan Academy
Can YOU Solve This MIND-BLOWING Logarithmic Equation?
Solving A Quick and Easy Logarithmic Equation
Solving Logarithmic Equations... How? (NancyPi)
Solving a Log Equation with Different Bases
Solving a Nice Ln Equation in Two Ways
❖ Solving Logarithmic Equations - Example 1 ❖
How to Solve Advanced Logarithmic Equations: Step-by-Step Tutorial
Let’s solve the LOGARITHMIC Equation - step-by-step….
Solving (Challenging) Log Equations Different Bases
Solving logarithmic equations
SOLVING LOGARITHMIC EQUATIONS || FINDING THE VALUE OF X
Logarithmic Equations - How to Solve
Ex: Solve Logarithmic Equations Containing Only Logarithms
Solving logarithmic equations by factoring
Logarithm Change of Base Formula & Solving Log Equations - Part 1 - [7]
04 - Solving Logarithmic Equations - Part 1 - Equations with Log(x)
Комментарии
 0:25:27
0:25:27
 0:00:55
0:00:55
 0:07:08
0:07:08
 0:09:46
0:09:46
 0:06:02
0:06:02
 0:09:47
0:09:47
 0:09:39
0:09:39
 0:04:13
0:04:13
 0:16:14
0:16:14
 0:09:26
0:09:26
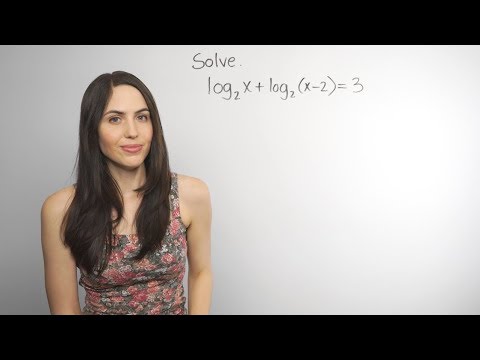 0:15:05
0:15:05
 0:08:47
0:08:47
 0:06:07
0:06:07
 0:03:08
0:03:08
 0:06:05
0:06:05
 0:11:46
0:11:46
 0:02:50
0:02:50
 0:00:57
0:00:57
 0:14:27
0:14:27
 0:03:51
0:03:51
 0:05:18
0:05:18
 0:07:00
0:07:00
 0:23:32
0:23:32
 0:27:26
0:27:26