Trigonometric Equations with Complex Numbers | Complex Analysis #6

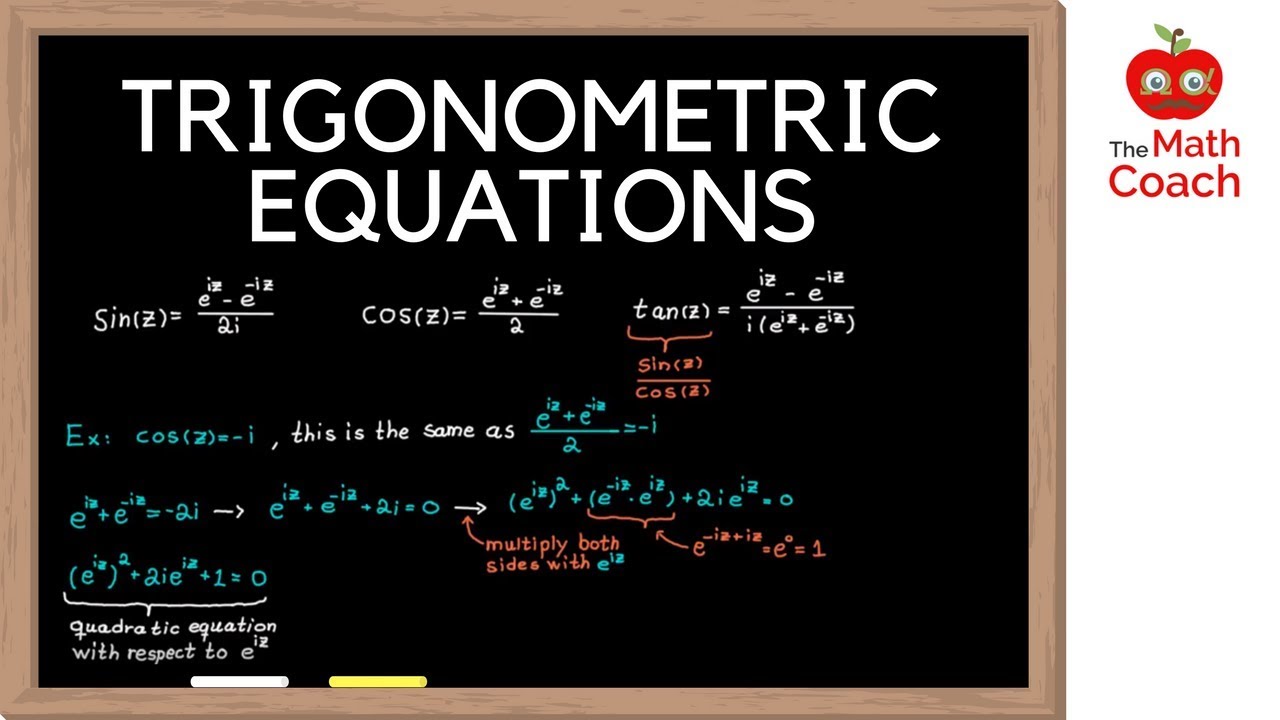
How to solve trigonometric equations with complex numbers. The video includes two different examples (cos(z) = -i and 3sin(z) + icos(z) = e^(iz)) and the method used is thoroughly explained and can also be used for equations with hyperbolic functions.

LINK TO COMPLEX ANALYSIS PLAYLIST

SUPPORT
Consider subscribing and liking if you enjoyed this video or if it helped you understand the subject. It really helps me a lot.

THE METHOD EXPLAINED IN THE VIDEO:
1) Start by rewriting the equation with the definitions of the trigonometric functions.
2) Multiply both sides with e^(iz) and solve for e^(iz). The equation is quadratic, but you might need to simplify it a bit for it to be obvious.
3) Use the complex logarithm to solve for z (determine the principal argument and the length (absolute value)).

IMPORTANT LINKS

CONCEPTS FROM THE VIDEO
► Trigonometric functions for complex numbers
The trigonometric functions can be expanded to the complex numbers by using Euler's formula.

►The Complex Logarithm function: ln(z) = ln|z| + i*arg(z) = ln|z| + i*(Arg(z) + n*2π) n = 0, +-1, +-2, ...
Is also called the multivalued logarithm ln(z) and is the "inverse" (but not really) of the complex exponential function. For a function to have an inverse, it must map distinct values to distinct values, i.e., be injective. But the complex exponential function is not injective since there exists an infinite number of different angles for the same complex number (e^(z+2πi) = e^z for any z).

► Principal Value of the Logarithm: Ln(z) = ln|z| + i*Arg(z)
Is a consequence of when the n in the complex logarithm function is equal to 0. The principal value Ln(z) is when the angle for the complex number lies in the interval (−π,π].

► Argument of a complex number: arg(z)
Is the angle from the positive real axis to the line joining the point to the origin. One complex number can have an infinite number of angles.

► Principal Argument of a complex number: Arg(z)
Is the angle from the positive real axis to the line joining the point to the origin, but the angle most lie in the interval (−π,π].

EDITS
Clockwise and Anti-Clockwise are hard okay :)

TIMESTAMPS
Definition of the trigonometric functions: 00:00 - 00:50
Example cos(z) = -i : 00:50 - 06:35
Example 3sin(z) + icos(z) = e^(iz) : 06:35 - 13:04
Recap: 13:04 - 14:00

SOCIAL

HASHTAGS
#TheMathCoach #ComplexAnalysis

[MajdAlmuntaser-bx]

You are one of the best who explains mathmatics out there. Thank you so much

[domero]

The examples are very useful, and doing them helps with remembering the formulas.

[nitunsingh]

i was looking for this topic for sometime..but i didn't get a better content.. then suddenly i saw your lectures ..these are awesome..thanks alot..sir

[PunmasterSTP]

This was incredible; keep up the phenomenal work!

[gmanxx]

The second method's answer at 13:00 is equivalent to the pair of solutions in the first method because when n is even you get the first of the pair, and when n is odd you get the second of the pair.

[kylefeist]

These videos are exceptional! Thanks so much!

[edinsonvera]

Amazing channel, I´m so glad I found your videos for learn

[letele.]

6:44 Some of us did the examples. Unlike most of you. 😌😅... Your playlist on Complex analysis is golden 💯💯

[liki]

Can someone please explain how we got the length of the complex number in the first example?

[elitheiceman]

can we solve normal equations with complex numbers

[Saucepv]

At 7:20, why after you distrbuted, the e^iz are negative ?

[darkseid]

Is there a way to solve infinite products of trigonometry using complex numbers ?
Like for example, we can solve infinite sum of trigonometric functions using C+iS method is there any method for infinite product ?

[quran_pakshorts]

Plz prove 1+tan^2z=sec^2z I really need plz solve