optimization problems ultimate study guide (area & volume)

You will learn how to solve optimization problems involving areas and volumes for your Calculus 1 class.

Thanks to @itsbishop2285 for the timestamps
0:00 Calculus 1 optimization problems
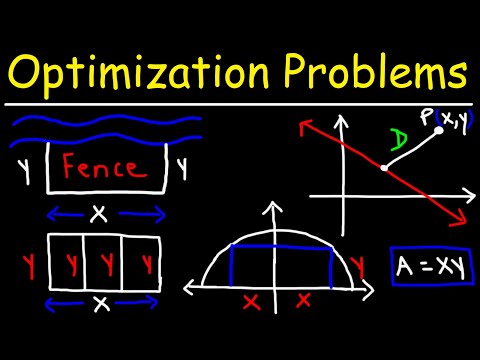
(Q1.) 0:35 Find the dimensions of a rectangle with an area of 1000 m2. whose perimeter is as small as possible.

(Q2.) 8:35 A farmer has 2400 ft of fencing and wants to fence off a rectangular field that boards a straight river. He needs no fence along the river. What are the dimensions of the field that has the largest area?

(Q3.) 14:30 The top and bottom margins of a poster are each 6 cm and the side margins are each 4 cm. If the area of printed material on the poster is fixed at 384 cm2, find the dimensions of the poster with the smallest area.

(Q4.) 23:04 Find the dimension of the rectangle of the largest area that has its base on the x-axis and its other two vertices above the x-axis and lying on the parabola y=12-x^2

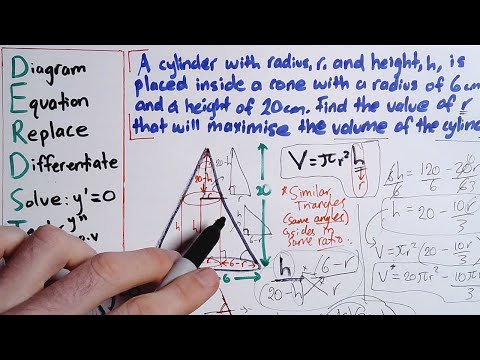
(Q5.) 28:38 A right circular cylinder is inscribed in a sphere of radius 4. Find the largest possible volume of such a cylinder.

(Q6.) 42:22 A rectangular package to be sent by a postal service can have a maximum combined length and girth (perimeter of a cross-section) of 90 inches (see figure). Find the dimensions of the package of the maximum volume that can be sent.

(Q7.) 47:07 A box with an open top is to be constructed from a square piece of cardboard, 6 ft wide, by cutting out a square from each of the four corners and bending up the sides. Find the largest volume that such a box can have.
** 53:13 The unit should be ft^3 **

(Q8.) 53:21 A box with a square base and open top must have a volume of 32,000 cm3. Find the dimensions of the box that minimize the amount of material used.

-----------------------------
-----------------------------

[bawankhalid]

amazing video and logic you are using. If i get a A in calculus 1, i give all the credit to you amazing teacher!!!!

[John.._]

ive watched so many optimization videos and this is the firs tto help. Thanks so much

[peterwoytowich]

very helpful, best optimization video out there

[whyyat]

Q3 is a good problem on one level, but a real gotcha on another. I wonder how many students forget to add in the margin measurements to get the total dimensions after going thru all the computing, especially if this question is given on a major exam....

[iurilourenco]

Great video! The cylinder one is so much easy if you draw the cylinder with it's height on the x axis

[ghAmputeeAtheist]

For the cylinder in the sphere: would have been much easier to maximize the volume as a function of y instead of x. The first and second derivatives are simple (no derivatives of square roots)

[broytingaravsol]

or these would be my latest materials for more practices

[Khalidmarwat]

Where can I get the pdf list of these questions.

[nifunakupunzilachabe]

On Q6 shouldn't the constraint eqaution be 2x+2y=90 which simplifies to x+y=45

[桜木秋水]

Q5. x^y^2=16, V=2πx^2y
x^2= 16-y^2, so, V(y)=2π(16-y^2)y
It's easier to do it this way.