filmov
tv
Describing rotation in 3d with a vector

Показать описание
Learn how a three-dimensional vector can be used to describe three-dimensional rotation. This is important for understanding three-dimensional curl.
Describing rotation in 3d with a vector
Quaternions and 3d rotation, explained interactively
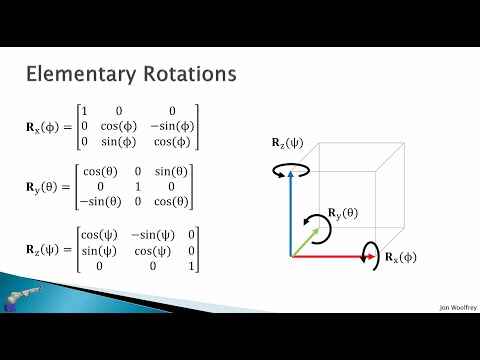
2.3 Rotations in 3D
Math Multivariable calculus - Describing rotation in 3d with a vector
How quaternions produce 3D rotation
What is Euler rotation?
3D rotation | Computer graphics | Lec-27 | Bhanu Priya
3D Rotation Non-Commutativity Example with Rubik's Cube
Week 13 Video 1: Rotations in 3D - Rotation Matrices, Euler Angles and Quaternions
3D rotation about an Arbitrary axis
3 Parameterizations of Rotation: Euler Angles, Axis-angle, and Roll Pitch Yaw
Rotation in Three Dimension
Three-dimensional linear transformations | Chapter 5, Essence of linear algebra
Rotations (Easier Method) 90 180 270
Understanding the Rotation Matrix
3D Rotation
Earth's Rotation Animation
Rotation Matrix and Reference Frames | Numerical Methods with Python 4
Rotation Matrix
3D Rotations in General: Rodrigues Rotation Formula and Quaternion Exponentials
Moon Rotation Around Earth Animation | Earth Rotation Around Sun For Kids
Rotation matrix derivation (step-by-step prove)
Angular Motion and Torque
The Four Transformations In Maths
Комментарии
 0:06:06
0:06:06
 0:05:59
0:05:59
 0:11:14
0:11:14
 0:06:06
0:06:06
 0:11:35
0:11:35
 0:01:00
0:01:00
 0:09:34
0:09:34
 0:00:15
0:00:15
 0:16:55
0:16:55
 0:00:36
0:00:36
 0:04:27
0:04:27
 0:18:36
0:18:36
 0:04:46
0:04:46
 0:01:33
0:01:33
 0:11:22
0:11:22
 0:03:01
0:03:01
 0:00:12
0:00:12
 0:05:07
0:05:07
 0:10:40
0:10:40
 0:33:09
0:33:09
 0:00:14
0:00:14
 0:05:05
0:05:05
 0:07:39
0:07:39
 0:04:13
0:04:13