filmov
tv
Geometric Algebra - 3D Rotations and Rotors
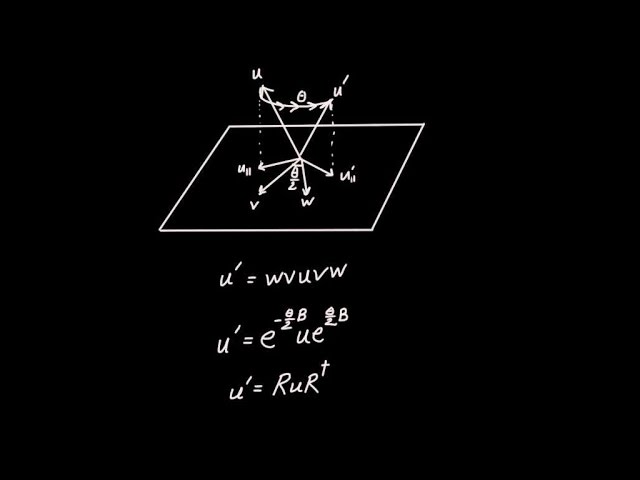
Показать описание
In this video, we employ the fact that two reflections is a rotation to perform rotations in 3D. We also will derive the general rotation formula, show how to compose rotations, and introduce the rotor version of this formula and discover the rotor's sign flip under a 2pi rotation.
References / Further Reading:
1. Lasenby and Doran's "Geometric Algebra for Physicists".
Note: This video builds off prior videos in this series, particularly:
Erratum:
33:50 - the sine function is odd, not even.
References / Further Reading:
1. Lasenby and Doran's "Geometric Algebra for Physicists".
Note: This video builds off prior videos in this series, particularly:
Erratum:
33:50 - the sine function is odd, not even.
Geometric Algebra - 3D Rotations and Rotors
Let's remove Quaternions from every 3D Engine: Intro to Rotors from Geometric Algebra
A Swift Introduction to Geometric Algebra
Quaternions Are Not Four-Dimensional Objects
Basic Intro to Quaternions for 3D Rotations
A Swift Introduction to Projective Geometric Algebra
The Fascinating perspective of Geometric Algebra #SoMEpi
Connecting GA Rotation Formulas
Basics of Rotation | Intro to Geometric Algebra
Three-dimensional linear transformations | Chapter 5, Essence of linear algebra
Geometric Algebra - Rotors and Quaternions
Geometric Algebra vs. Clifford Algebra
Geometric Algebra, First Course, Episode 12: Rotations
The Square Root of a Rotor
Are Bivectors Always Plane Segments?
2.7 Rotations | Geometric Algebra for Physicists
Quick Understanding of Homogeneous Coordinates for Computer Graphics
Decomposition of a 3D PGA Bivector
SR in 3D Geometric Algebra: Frames and Rotors
Addendum to A Swift Introduction to Geometric Algebra
Applications Of Conformal Geometric Algebra To Transmission Line Theory
3D Rotations in General: Rodrigues Rotation Formula and Quaternion Exponentials
Counterexamples in Geometric Algebra
Geometric Algebra 19 3D Geometric Algebra
Комментарии
 0:48:59
0:48:59
 0:16:48
0:16:48
 0:44:23
0:44:23
 0:00:41
0:00:41
 0:05:49
0:05:49
 0:54:38
0:54:38
 0:14:44
0:14:44
 0:00:51
0:00:51
 0:02:31
0:02:31
 0:04:46
0:04:46
 0:36:26
0:36:26
 0:00:54
0:00:54
 0:15:34
0:15:34
 0:00:42
0:00:42
 0:00:53
0:00:53
 0:06:21
0:06:21
 0:06:53
0:06:53
 0:00:52
0:00:52
 0:24:55
0:24:55
 0:23:40
0:23:40
 0:32:50
0:32:50
 0:33:09
0:33:09
 0:00:57
0:00:57
 1:00:35
1:00:35