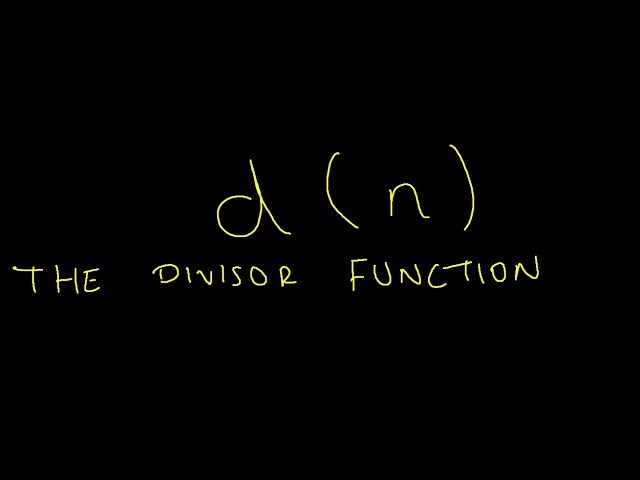
The Distribution of Primes #3 - The Divisor Function, d(n)

In this video, we introduce the divisor function, d(n). This is an arithmetic (multiplicative) function which counts the number of positive divisors of a natural number n. For example, d(4) = 3, because the positive divisors of 4 are 1, 2 and 4.

[crnobijeli]

Thank you so much! I was looking for a simple explanation of the divisor function and this helped me tremendously.

[jhawk]

You can also do this by taking the prime factorization, increasing the exponents by 1, and multiplying them. So 4 would be 2^2, 2 + 1 is 3 and that's it. 480 would be 10 * 48 = 2 * 5 * 2^4 * 3 = 2^5 * 3 * 5. Increasing the exponents by 1 and multiplying we get 6 * 2 * 2 which is 24 factors.