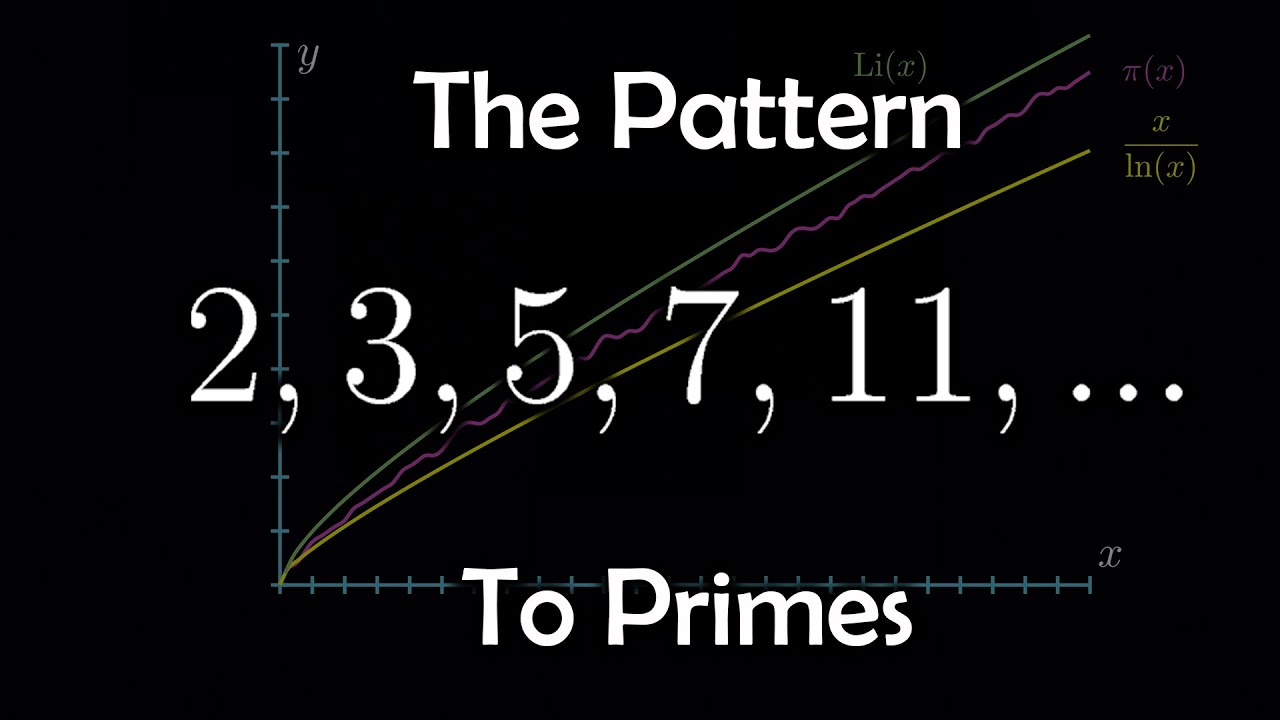
The Pattern to Prime Numbers?

Показать описание

In this video, we explore the "pattern" to prime numbers. I go over the Euler product formula, the prime number theorem and the connection between the Riemann zeta function and primes.

There are a few mistakes in this video, so I clarified them in a pinned comment. Sorry about that!

Follow Me!

Music by ChillHop

#primes #zeta #math

Рекомендации по теме

Комментарии

What's a vcubingx video without errors?
At 1:54 it should be "Q - P = 1" instead of "P - Q = 1"
At 3:04 it should be "Converges" instead of "Coverges"

vcubingx

You’re almost like a spiritual successor to 3Blue1Brown. Keep going, your videos are beautiful.

TheCarlagas

As a statistician, I twitched when I heard ‘when the p-value is greater than 1’.

ffs

A very good teacher who is spreading knowledge for free --- a noble deed!

RohanDasariMinho

It is intuitive to feel that primes have structure. Using Euler and Euclid, Reimann subjected this intuition to rigorous analysis.. He got further than anyone else and left a great legacy. This is a fantastic video, unless you are a prime number, hiding out there in integer space somewhere. In which case you should be worried, because soon your number will be up!

petergregory

I'm only 5 minutes in but already have to comment! I love your explanation of the Euler Product formula, it seems like it would be intimidating to derive given its connection to the Zeta function but you did it beautifully

PenguinMaths

I dont know, but your style is like 3B1B's

oli

i think euclid's theorem works like this (noting this corrects the slight mistake in the video where it suggests that P - Q = 1 at 2:02):

- assume there is a finite number of primes
- then there exists a number P which is the product of this finite set of primes
- consider a number Q = P + 1
- by definition, Q is either prime or non-prime
- CASE 1: if Q is prime, then P is NOT the product of all primes (because Q = P + 1 implies that Q > P and no number greater than P can be a factor of P)
- hence, Q being prime leads to a contradiction
- CASE 2: if Q is NOT prime, then we should be able to factor Q as a product of primes (in the manner demonstrated for 30 earlier in the video)
- let one of Q's prime factors be the prime number p
- recalling that P is the product of ALL primes, p must also be a prime factor of P
- therefore p divides both P and Q
- i THINK there's a theorem which says that it follows that p must also divide Q - P (e.g. think of 3 as a prime factor of both 9 and 15 which leads us to know that 3 is also a prime factor of 15 - 9 = 6).
- by rearranging the original equation, we find that Q - P = 1. hence p should divide 1 by this logic.
- as the video-maker then explains, no number divides 1, so p cannot divide 1 either
- hence, assuming Q being non-prime led to a contradiction
- therefore, the original assumption that there is a finite set of primes must be false
- therefore, the set of primes is infinite

dylanparker

Your channel is hidden goldmine. Underrated!!!

randomdude

Incredibly high quality video. In those 16 minutes you went on such a structured clear and deep route into a topic in a way that most other popular mathematics channels never will.

adenpower

This the best Riemann hypothesis video till date...it take from first basic prime theorem to non-trivial zeroes of zeta function, and this video is not to complicated, I loved it.

jayvaghela

The pattern to prime numbers is that they are prime

SSJAydan

Your video editing skills are really good!

pizzafood

best video that explains the background but also covers different aspects of Riemann function and primes. but have you or anyone found a pattern yet ?

goldenera

I appreciate this video, I’ve always been confused as to how the zeta function relates to primes but you laid it out pretty solidly. I feel like that section would benefit from more clearly explained math but I understand it.

spearmintlatios