filmov
tv
Master Evaluating Logarithmic Expressions using the Change of Base Formula

Показать описание
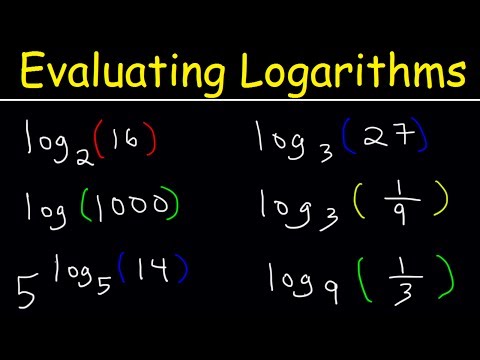
So what I want to do is at least present the formula for you. And then I was also showing you, because if you have a calculator where you cannot determine the base, you have to use log base 10. So I'll kind of give you a couple different ways we can do this.
Now for the first two problems though, you should at least know what the answers are. But I want to use the change of base formula so therefore we can see why it works and how it works. So basically the change of base formula is if you have a logarithm base b of a, then we can take the logarithm of any base y of a and divide it by a log of that same base of b. Or we could do the natural log, which would obviously therefore then if you did the natural log, then y would equal e, right? So it would be the natural log of a over the natural log of b.
Or you could also just look at it as log a over log b in your calculator, because the majority of calculators-- for instance, like a scientific like this-- only has log and base 10. Whereas some TI calculators, you can actually change your base.
So if we were going to look at this, we would know this is going to be base 10, right? So what I want to do is let's go ahead and use this as log base 10. Let's do our change of base formula for this.
So therefore I can rewrite this as log base 10 of 100 divided by log base 10 of 10. Excuse me. So if I change this into log base 10 of 10 divided by log base 10 of 10. So that was my a, and this is my b.
Now again, we already know what log base 10 of 10 is, right? 10 raised to what power gives you 100? We should know the answer is 2. Well 2 over 1, which just equals 2.
You know what, I don't want to do this again. Let's change this. Let's do this to-- let's do 4 over 8. That will work. OK, so now if I was going to a problem like this, log base 4 of 8.
Now I can rewrite this to any logarithm, right? And the easiest probably would be, if you were going to use your calculator, would just be log of 8 divided by log of 4. And you can just plug it in your calculator and I'll show you what to do for those in just a second.
However, I wanted to prove to you that this works. So if you were to look at this, instead of using log base 10, let's use log base 2. So therefore to rewrite this, I'm going to have my y is going to be 2. So my a is 8. So it's log base 2 of 8 over log base 2 of 4.
Now remember, again, you can choose whatever base you want to. So your a and your b have to remain there, but you can choose whatever base you want to. For your calculator, you're going to have to use, more than likely, you're going to use log base 10, because you just type in log and it automatically will be base 10. Or you can use natural log.
However, without a calculator, 2 raised to the eighth-- 2 raised to what power gives you 8-- 3. 2 raised to what power gives you 4-- 2. So the answer is 3/2.
And if you were to verify that in your calculator, you could do log of 8. Actually, that's just do that. Log of 8 divided by log of 4. And what do you get? I get 1.5, which is 3/2, decimal version.
All right, so over here, again as I was mentioning, I'm going to work on this a couple different ways. So if I was going to use my calculator-- now in this case, I don't have the bases are the same. I can't rewrite them like I did over here and pick a base. So therefore I'm simply, for my calculator, I'm going to do log of 8 over the log of 3.
So simply in my calculator, I'm just going to find my log. And I'm going to do log of 8. I'm going to close my parentheses, divided by the log of 3, close parentheses. And I get 1.892789. So therefore I'm approximating. So they can say it's going to be approximately one point-- I'm going around to the 100th-- 89. All right.
And the next example over here. Again, you can use log base 10, but all calculators that are going to have logarithm are also going to have your natural logarithm. So since the base y doesn't matter, I could also do ln of 4 divided by ln of 7. So basically you're taking the logarithm of your value, and then the logarithm of your base of your logarithm usi
Комментарии
 0:10:20
0:10:20
 0:14:46
0:14:46
 0:02:16
0:02:16
 0:07:29
0:07:29
 0:06:38
0:06:38
 0:06:15
0:06:15
 0:20:27
0:20:27
 0:02:42
0:02:42
 0:10:23
0:10:23
 0:25:27
0:25:27
 0:04:20
0:04:20
 0:08:58
0:08:58
 0:03:27
0:03:27
 0:01:28
0:01:28
 0:01:15
0:01:15
 0:37:57
0:37:57
 0:02:30
0:02:30
 0:51:02
0:51:02
 0:01:41
0:01:41
 0:03:45
0:03:45
 0:05:40
0:05:40
 0:00:30
0:00:30
 0:13:09
0:13:09
 0:00:19
0:00:19