Master Evaluating a Logarithmic Expressions using the one to one property

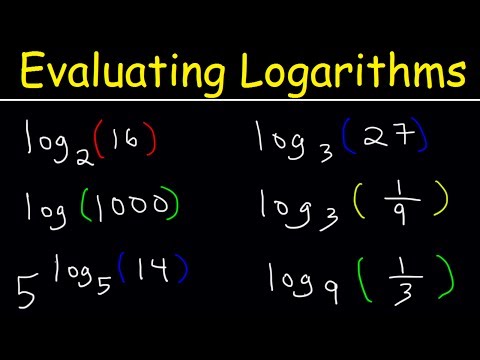
So this one-to-one property basically is, if x raised to the a is equal to x raised to b, then a equals b. Now, that might make sense, might not make sense, so I gave you an example. An example is, if I have 3 raised to some number x and that's equal to 3 squared, well, it would kind of makes sense that if 3 to the x is equal to 3 squared, then that x has to equal 2.

So remember when we're looking at a logarithm, at lease for all these, we're basically asking us 27 raised to what power is going to equal 3? So I think it's kind of beneficial when doing these problems to kind of rewrite it in that exponential format. So I can say 27 raised to what number is going to equal to 3?

Now, I don't know what number 27 raised up to 3. It's not as simple as like the last video I did where three raised to what number gives you 9? It's 2. But in this, it's not very transparent. So what I can do though is can use the one-to-one property. Basically, if I can get this to be an expression or an equation where I have the base that's exactly the same, then I know my powers are going to be equal to each other.

Well, 3 and 27 are related to each other because 3 to the first power is 3, 3 squared is 9, and 3 cubed is going to equal 27. So therefore, I can rewrite 3 cubed raised to the x equals 3. Now, I can use my power rule and I'm not going to do this for all of them, but I've kind of run out of space here. Maybe I'll do it for the last ones.

Anyway, so therefore now, my bases are exactly the same, so therefore, I have-- now this, you could say, well, what is this raise to? Well, you could just say that's raised to the first power. So now you could say 3x is equal to 1 whereas, x is equal to the 1/3 power. Kind of running out of space here, so that's why I kind of went over from there.

So if we do the next example, again, I would do the same thing. I could say 16 raised to what value is going to equal 2?

Now, hopefully, we understand that 2, we have 2 to the first part equals 2. 2 to the second power equals 2. 2 to the third power is 8. 2 to the fourth power is 16. So I could rewrite this as 2 to the fourth raised to the x equals 2.

Now, basically what I didn't show over here is, you should use the power rule at least to show that that's going to be 2 to the 4x equals 2, and then you can raise that to the first power. Now you can divide them out and we could say x equals to the 1/4. Kind of running out of space on those, aren't I?

For the next one, again, we can just do it one last time, so I could do 16 raised to what value is going to leave me with 8? So therefore, x equals 1/4. I should write these in there. It's not really mathematically the way I want to do it. So log base 27 of 3 is 1/3. Log base 16 of 2 is 1/4.

So log base 16 of 8, I can rewrite 16 and 8. Now, you can see that these-- I can't write 16 to a power that's going to give me 8. So I got to look back into my values and think, well, what base thing can I write?

Could I do 4 because I know 4 squared is 16, but I can't make 4 and raise it to a power to give me 8. I can always go back to my 2's though. Because 2 to the fourth power is 16, and 2 to the third power is 8.

So in this case, I'm going to do both of them. So I'm going to do 2 to the fourth power, x equals 2 cubed. Now, using my one-to-one property, I'm left with 4x is equal to 3. Divide by 4, divide by 4, and I get x equals 3/4. So I have x equals 3/4 in that example.

Now, for the next one, I'm going to use green. Actually, I'm going to go over here and use my blue just so you guys can see the difference and I'm not getting everything crowded here.

All right. So now I'm going to do 9 over to the 27th. So you have 9 to the x equals 27. Well, again, 9 squared is 81, so obviously, it's not go work, however, I can rewrite 3 squared is 9 and 3 cubed is 27. So I could do 3 squared raised to the x equals 3 cubed.

.