filmov
tv
Conic Sections: Ellipses: Example 2: Vertical Ellipse

Показать описание
In this video I go over another example on conic sections and ellipses, and this time look at determining the equation of an ellipse when we are given the foci (plural for focus) and the vertices. In this particular example, since the foci (0 , +/- 2) and vertices (0 , +/- 3) are on the y-axis, we obtain a vertical ellipse. Thus we use the formula for an ellipse that involves the a^2 term below the y^2 term: x^2/b^2 + y^2/a^2 = 1. Then all we need to use the definition of b^2 = a^2 – c^2 from my derivation video for ellipses, to get b^2 = 5. Note that b corresponds to the end points on the minor axis of the ellipses, whereas the vertices correspond to the end points on the major axis. From here we get the ellipse x^2/5 + y^2/9 = 1 or 9x^2 + 5y^2 = 45. This is a simple but very detailed look at determining the equation of a vertical or inverted ellipse so make sure to watch this video!
Related Videos:
------------------------------------------------------
Related Videos:
------------------------------------------------------
Conic Sections: Ellipses: Example 2: Vertical Ellipse
Graphing Conic Sections Part 2: Ellipses
Writing Equations of Ellipses In Standard Form and Graphing Ellipses - Conic Sections
Conic Sections: Ellipse Example 1
AQA A-Level Further Maths D15-07 Conic Sections: Ellipse Example 2
Conic Sections: Graphing Ellipses Part 2
Ellipse Graph
Conic Sections - Ellipses (Part 2 of 3)
Conic Sections: Ellipses: Example 1
conic section parabola hyperbola circle ellipse ||,#shorts,👆🔥😂💯
What are Conic Sections?
Conic sections satisfying animation #short
Conics in Polar Coordinates: Example 2: Ellipse
Ellipses | Conic Sections | 4 Examples
9.4: Conic Sections: Ellipses (Algebra II)
Conic Sections; Parabola, Ellipse, and Hyperbola - Calculus II
❖ Conic Sections , Ellipse : Find the Foci of an Ellipse ❖
Conic Sections: Hyperbolas, Ellipses, Parabolas, Circles (How to Graph)
01 - Conic Sections: Ellipses - Graphing, Equation of an Ellipse, Focus - Part 1
Conic Sections Part 2 - Ellipse and Hyperbola
Conic Sections Quiz - Parabolas, Hyperbolas, Ellipses, & Circles
Algebra 2 Conic Section Ellipse 1
How to find the center, foci and vertices of an ellipse
What is Ellipse?Its eq with related terms#Shorts Class_11 Conic Sections
Комментарии
 0:08:10
0:08:10
 0:06:33
0:06:33
 0:31:39
0:31:39
 0:05:23
0:05:23
 0:03:16
0:03:16
 0:13:37
0:13:37
 0:01:01
0:01:01
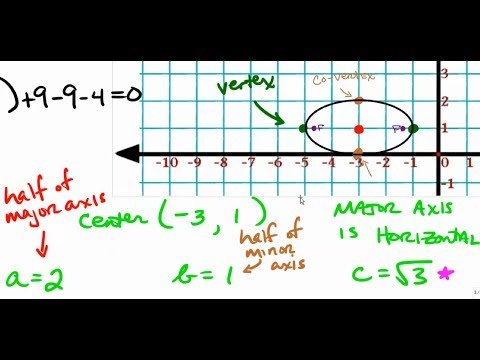 0:28:24
0:28:24
 0:11:20
0:11:20
 0:00:16
0:00:16
 0:00:44
0:00:44
 0:00:29
0:00:29
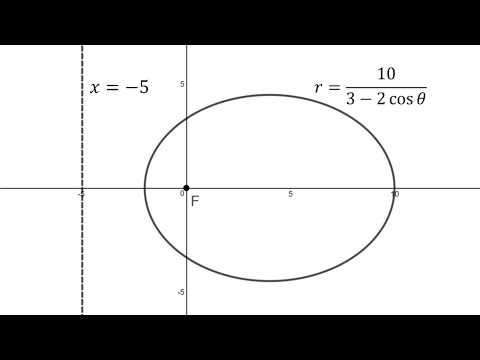 0:16:21
0:16:21
 0:29:24
0:29:24
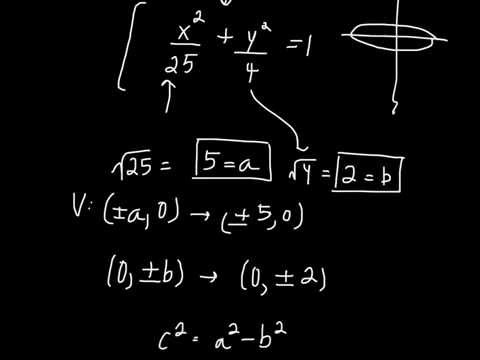 0:05:39
0:05:39
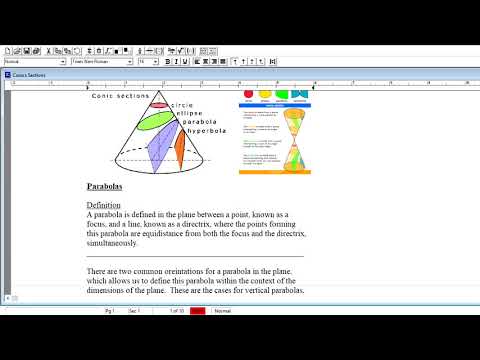 1:04:41
1:04:41
 0:02:58
0:02:58
 0:29:17
0:29:17
 0:33:42
0:33:42
 0:11:18
0:11:18
 0:29:31
0:29:31
 0:05:49
0:05:49
 0:13:29
0:13:29
 0:00:16
0:00:16