filmov
tv
Derivative of |x| (absolute value x)

Показать описание
In this short video I show how to find the derivative of |x|, the absolute value of x. In one minute, I cover the square root definition of absolute value x, the Chain Rule and the Power Rule. This formula is hard to remember in calculus, so I am teaching how to derive the formula so that you will never forget the derivative of absolute value x.
Derivative of absolute value function
Derivative of Absolute Value of x from First Principles
Why isn't abs(x) differentiable at x=0? (definition of derivative)
Derivative of |x| (absolute value x)
Derivative of The Absolute Value of x | JK Math
Integral of absolute value of x or abs(x)
Calculus calc 7 Derivative of absolute value functions mathgotserved how to fast easy trick
Derivative of x+abs(x)
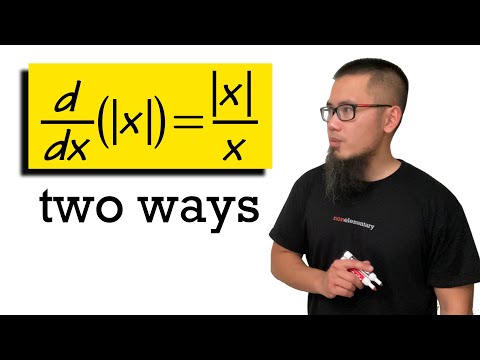
Derivative of abs(x), two ways
Derivative of Absolute Value of x at x = 0
Derivative of abs(x^3 - 1)
Symmetric derivative of abs(x) at 0
How to determine if the derivative exist from the left and right of a absolute value
The Derivative of The ABSOLUTE VALUE of x / How to find
Prove Derivative of Absolute X is Quotient of X and Absolute X MCV4U Calculus
The Integral and Derivative of an Absolute Value
Derivative of Absolute Value Function using Limits (example problem)
The Derivative of The Absolute Value of x
How To Differentiate Modulus Function | Derivative Calculus
5 simple unsolvable equations
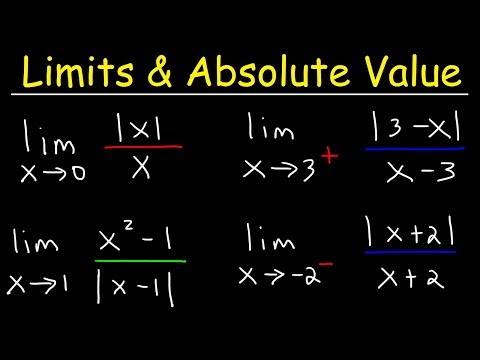
Limits and Absolute Value
Derivative of Absolute Value Functions
Why absolute value is necessary in derivative of inverse secant
Derivative of absolute value functions - - derivative of a function - part 4
Комментарии
 0:08:04
0:08:04
 0:04:30
0:04:30
 0:09:02
0:09:02
 0:01:00
0:01:00
 0:06:12
0:06:12
 0:01:59
0:01:59
 0:07:35
0:07:35
 0:04:09
0:04:09
 0:01:28
0:01:28
 0:03:43
0:03:43
 0:00:46
0:00:46
 0:01:45
0:01:45
 0:04:12
0:04:12
 0:03:49
0:03:49
 0:02:22
0:02:22
 0:06:26
0:06:26
 0:05:50
0:05:50
 0:03:05
0:03:05
 0:00:44
0:00:44
 0:00:50
0:00:50
 0:17:44
0:17:44
 0:05:37
0:05:37
 0:02:54
0:02:54
 0:04:26
0:04:26