filmov
tv
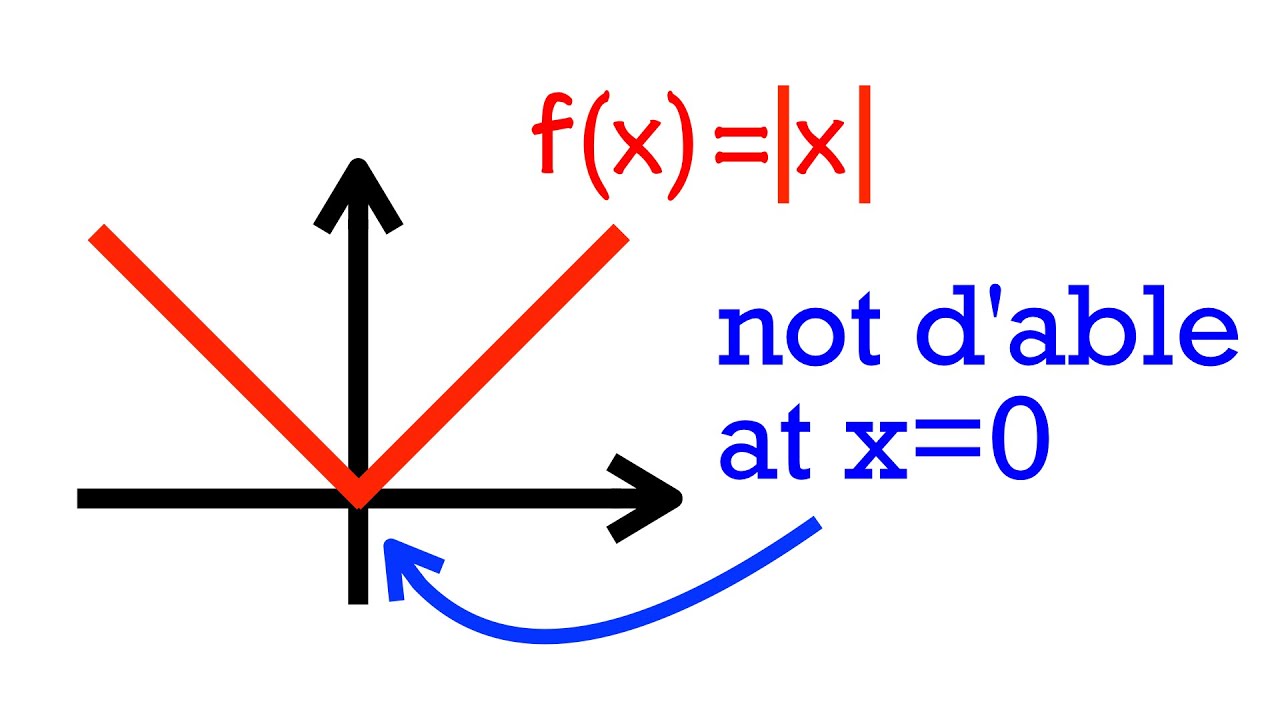
Why isn't abs(x) differentiable at x=0? (definition of derivative)
Показать описание
We will use the definition of derivative to show f(x)=abs(x) is not different x=0.
This is a classic counterexample that continuous functions might not be .
For more calculus tutorials, please see my new "just calculus" channel:
If you find my videos helpful, then consider supporting me on Patreon:
This is a classic counterexample that continuous functions might not be .
For more calculus tutorials, please see my new "just calculus" channel:
If you find my videos helpful, then consider supporting me on Patreon:
Why isn't abs(x) differentiable at x=0? (definition of derivative)
Why is absolute value not differentiable at x=0? Derivatives
This is Why the Absolute Value Function is Not Differentiable At Zero
Show Absolute Value Function Not Differentiable at x = 0
Where a function is not differentiable | Taking derivatives | Differential Calculus | Khan Academy
How to show that y=abs(x) or y=|x| is not differentiable at (0,0)
Derivative of x+abs(x)
Explain why Absolute Function is not differentiable at origin MCV4U
Why is the absolute value function not differentiable? - Week 3 - Lecture 3 - Mooculus
Proof that the Absolute Value Function is Not Differentiable at Zero
Differentiability (2 of 3: Absolute Value of x)
Symmetric derivative of abs(x) at 0
|x| is Continuous but not Differentiable | Calculus 1 Exercises
Continuity and Differentiability
Integral of abs(x) in 44 seconds!
WTH IS A SUBDERIVATIVE?! Calculating the Subdifferential of abs(x) [ |x| ]
show that the derivative of abs(x-6) does not exist at x=6 by using the definition of derivative
modulus derivative !! 😱 How ? can u find why ??
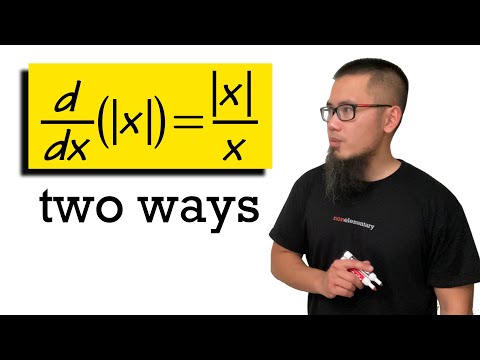
Derivative of abs(x), two ways
abs(x)^3/2 absolute power 3/2 Continuity and Differentiability Calculus
why mod x is not differentiable at x=0
Calculus - Lesson 9 | When does the Derivative Not Exist? | Don't Memorise
IMPORTANT Derivative of product of x and absolute x at zero if it exists
Derivative of abs(x^3 - 1)
Комментарии
 0:09:02
0:09:02
 0:09:51
0:09:51
 0:05:45
0:05:45
 0:06:14
0:06:14
 0:03:35
0:03:35
 0:00:58
0:00:58
 0:04:09
0:04:09
 0:07:39
0:07:39
 0:02:39
0:02:39
 0:03:10
0:03:10
 0:03:30
0:03:30
 0:01:45
0:01:45
 0:09:06
0:09:06
 0:32:48
0:32:48
 0:00:44
0:00:44
 0:06:55
0:06:55
 0:05:37
0:05:37
 0:00:16
0:00:16
 0:01:28
0:01:28
 0:06:33
0:06:33
 0:00:55
0:00:55
 0:08:52
0:08:52
 0:07:18
0:07:18
 0:00:46
0:00:46