filmov
tv
Reciprocals, powers of 10, and Euler's totient function II | Data Structures Math Foundations 203

Показать описание
We introduce the idea of the unit group U(n) of a natural number n. This is an algebraic object that contains important data about how multiplication mod n works, even for a composite number n. There is a natural connection with Euler's totient function, and we will see how to exploit this to figure out how big the repeated cycles are for a fraction such as 1/n, even for a big number n.
Along the way, we will summarize some important number theory / group theory facts relating to the unit groups, including prominently Lagrange's theorem.
You might notice the video looks a bit different--I now have a new camera, and am working on getting my head around using it!
************************
Here are the Insights into Mathematics Playlists:
Along the way, we will summarize some important number theory / group theory facts relating to the unit groups, including prominently Lagrange's theorem.
You might notice the video looks a bit different--I now have a new camera, and am working on getting my head around using it!
************************
Here are the Insights into Mathematics Playlists:
 0:24:27
0:24:27
 0:25:33
0:25:33
 0:03:57
0:03:57
 0:01:11
0:01:11
 0:01:10
0:01:10
 0:03:20
0:03:20
 0:06:45
0:06:45
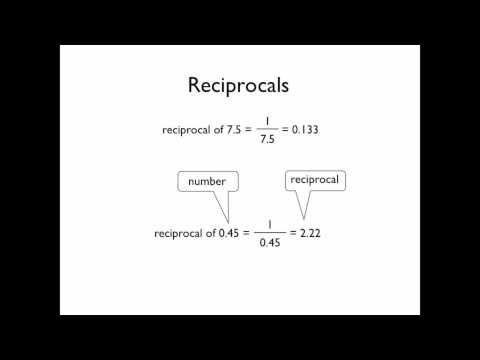 0:02:41
0:02:41
 0:02:57
0:02:57
 0:28:11
0:28:11
 0:03:28
0:03:28
 0:12:38
0:12:38
 0:00:55
0:00:55
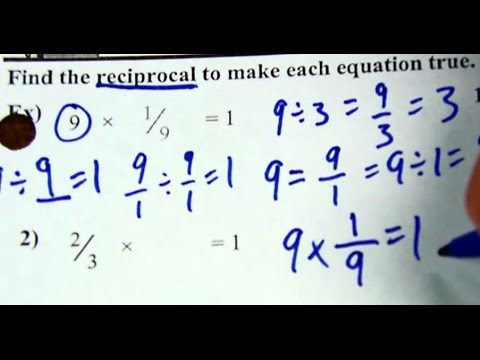 0:04:34
0:04:34
 0:00:24
0:00:24
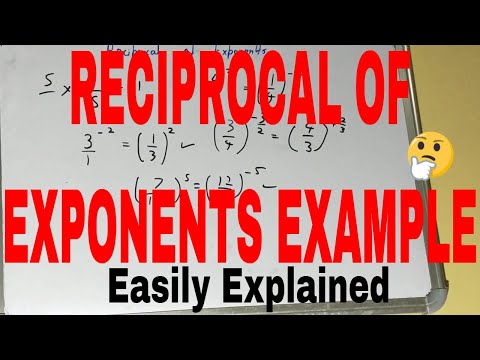 0:03:19
0:03:19
 0:04:45
0:04:45
 0:15:31
0:15:31
 0:02:01
0:02:01
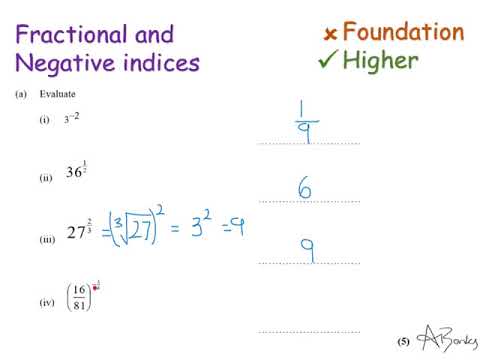 0:05:28
0:05:28
 0:12:30
0:12:30
 0:13:47
0:13:47
 0:00:57
0:00:57
 0:00:09
0:00:09