filmov
tv
Linear Algebra 6f: Linear Dependence Example 2 - Geometric Vectors

Показать описание
Linear Independence
Linear Independence and Linear Dependence, Ex 1
Introduction to linear independence | Vectors and spaces | Linear Algebra | Khan Academy
Linear Algebra 6f: Linear Dependence Example 2 - Geometric Vectors
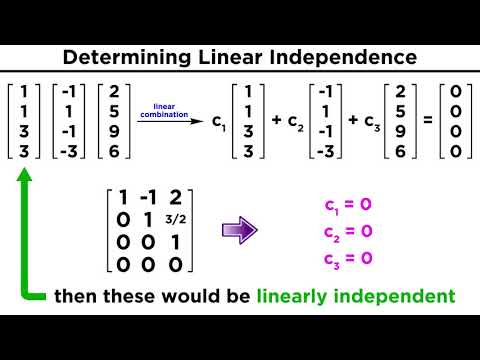
Determining Linear Independence vs Linear Dependence
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
More on linear independence | Vectors and spaces | Linear Algebra | Khan Academy
[Linear Algebra] Linear Independence
Basis and Dimension for csir net #vectorspace #linearalgebra
Linear Independence of Functions & The Wronskian
Linear Algebra Example Problems - Linearly Independent Vectors #1
Linear Independence Problems - Using the Definition
Linear Algebra - Lecture 15 - Linear Independence
Linear Algebra 6c: Second Definition of Linear Dependence Fixed!
Linear Dependence and Independence - Geometrically
Span and linear independence example | Vectors and spaces | Linear Algebra | Khan Academy
How to find out if a set of vectors are linearly independent? An example.
[Linear Algebra] Linear Independence and Bases
Linear Algebra 1.7.1 Linear Independence
Check for Linear Dependence (of 2 functions)
06 Linearly Dependent Vectors Exercise
Linearly Dependent Vectors | Example of Linearly Dependent Vectors
Oxford Linear Algebra: Basis, Spanning and Linear Independence
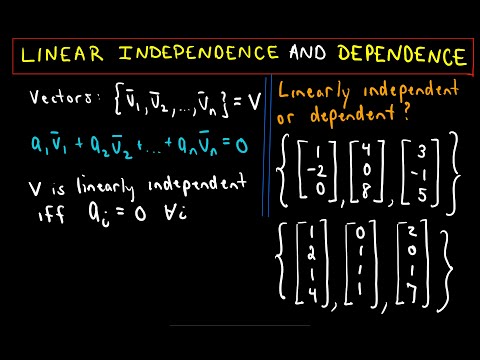
Linear Independence
Комментарии
 0:12:56
0:12:56
 0:08:05
0:08:05
 0:15:46
0:15:46
 0:08:47
0:08:47
 0:06:39
0:06:39
 0:09:59
0:09:59
 0:17:38
0:17:38
![[Linear Algebra] Linear](https://i.ytimg.com/vi/XI2kYIxhe-o/hqdefault.jpg) 0:16:05
0:16:05
 0:01:00
0:01:00
 0:09:59
0:09:59
 0:04:58
0:04:58
 0:09:05
0:09:05
 0:13:46
0:13:46
 0:04:29
0:04:29
 0:08:16
0:08:16
 0:16:53
0:16:53
 0:04:41
0:04:41
![[Linear Algebra] Linear](https://i.ytimg.com/vi/OLqc_rt7abI/hqdefault.jpg) 0:08:40
0:08:40
 0:11:17
0:11:17
 0:07:09
0:07:09
 0:04:04
0:04:04
 0:09:08
0:09:08
 0:41:44
0:41:44
 0:10:54
0:10:54