Factoring an expression with a greater than one and two square variables 8x^2 -6xy -9y^2

Показать описание

👉Learn how to factor quadratics when the coefficient of the term with a squared variable is not 1. To factor an algebraic expression means to break it up into expressions that can be multiplied together to get the original expression.

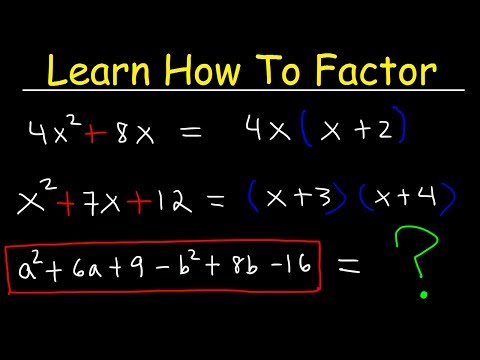
To factor a quadratic trinomial where the coefficient of the term with a squared variable is not 1, we find two expressions which when multiplied together gives the product of the constant term (the term with no variable) and the term with the squared variable and when the two expressions are added gives the term whose variable has no power. These two expressions obtained are used to replace the term whose variable has no power. The result is then factored by grouping them and factoring out the GCD.

There are other methods that can be used to achive this including the AC, Berry, Box, Grouping and mental technique. Each way will have it's benefits but it is also helpful to check your answer by multiplying your binomials to make sure they give the original trinomial

Organized Videos:
✅Factor Quadratic Expressions
✅Factor Quadratic Expressions | Learn About
✅Factor Quadratic Expressions | GCF
✅Factor Quadratic Expressions | x^2+bx+c
✅Factor Quadratic Expressions | x^2+bx+C
✅Factor Quadratic Expressions | Difference of Two Squares
✅Factor Quadratic Expressions | Perfect Square
✅Factor Quadratic Expressions | ax^2+bx+c

Connect with me:

#quadratics #factoring #brianmclogan

Рекомендации по теме

Комментарии

Thanks so much
I’m doing further maths GCSE and my teacher didn’t have time to teach the whole thing so this really helped

halaco

You make it look so easy, I hope you keep making these

shanehooper

There is another solution to this problem: (-2x + 3y)(-4x - 3y). Check it out. Also there is a more general approach to solving all problems of this type. Note that you can write the general solution as (ax + by)(cx + dy). Note that ac will be the coefficient of the first term of the expression you are attempting to factor. Note that bd will be the coefficient of the last term, and that (ad +bc) will be the coefficient of the middle term.

Summarizing our constraints for this problem gives
ac = 8
bd = -9
ad +bc = -6

To solve this problem simply find the ordered pairs of integers that meet the ac and bd criteria.

For ac these are (4, 2), (2, 4) (-4, -2) (-2, 4), (8, 1), (1, 8), (-8, -1), and (-1, 8)

for bd these are ((3, -3), (-3, 3), (-1, 9), (9, -1)

Now all you have to do is find the combinations of pairs that meet the ad + bc criteria. There are 4 x 8 combinations to check.
With this approach you can solve any quadratic of this form.

The really interesting problem is not wasting a lot of time factoring quadratics by arbitrary heuristics, but to come up with a general solution like this and then use it to solve ALL the problems of this type. Once you have this type of solution write a program that will do the checking of the coefficient combinations for you. This is just simple arithmetic, and the most error prone aspect of solving problems like this. That is a job that computers do best. For a slightly bigger challenge write your program to find all of the ordered pairs you will need. Finding general solutions to problems is what real math and computer science is all about.

garretta.hughes