filmov
tv
Discrete and continuous random variables | Probability and Statistics | Khan Academy

Показать описание
Defining discrete and continuous random variables. Working through examples of both discrete and continuous random variables.
Missed the previous lesson?
Probability and statistics on Khan Academy: We dare you to go through a day in which you never consider or use probability. Did you check the weather forecast? Busted! Did you decide to go through the drive through lane vs walk in? Busted again! We are constantly creating hypotheses, making predictions, testing, and analyzing. Our lives are full of probabilities! Statistics is related to probability because much of the data we use when determining probable outcomes comes from our understanding of statistics. In these tutorials, we will cover a range of topics, some which include: independent events, dependent probability, combinatorics, hypothesis testing, descriptive statistics, random variables, probability distributions, regression, and inferential statistics. So buckle up and hop on for a wild ride. We bet you're going to be challenged AND love it!
About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content.
For free. For everyone. Forever. #YouCanLearnAnything
Subscribe to KhanAcademy’s Probability and Statistics channel:
Комментарии
 0:11:56
0:11:56
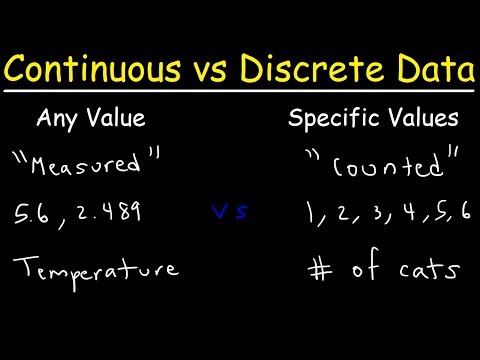 0:09:03
0:09:03
 0:02:17
0:02:17
 0:05:35
0:05:35
 0:10:13
0:10:13
 0:09:09
0:09:09
 0:08:57
0:08:57
 0:14:11
0:14:11
 0:42:15
0:42:15
 0:16:23
0:16:23
 0:08:41
0:08:41
 0:04:39
0:04:39
 0:18:54
0:18:54
 0:05:37
0:05:37
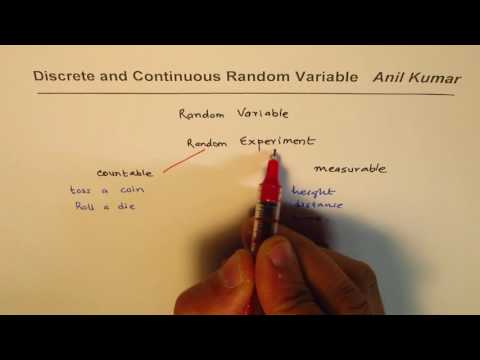 0:06:01
0:06:01
 0:11:29
0:11:29
 0:23:16
0:23:16
 0:10:48
0:10:48
 0:05:11
0:05:11
 0:10:06
0:10:06
 0:29:54
0:29:54
 0:07:10
0:07:10
 0:45:04
0:45:04
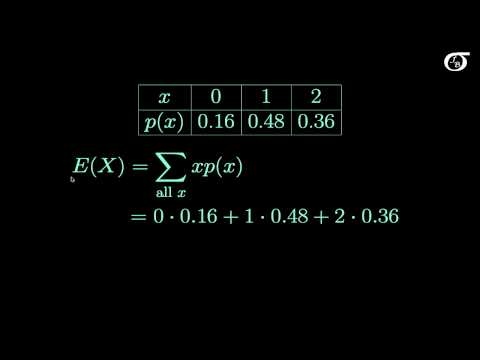 0:07:57
0:07:57