filmov
tv
Probability with discrete random variable example | Random variables | AP Statistics | Khan Academy

Показать описание
Example analyzing discrete probability distribution.
AP Statistics on Khan Academy: Meet one of our writers for AP¨_ Statistics, Jeff. A former high school teacher for 10 years in Kalamazoo, Michigan, Jeff taught Algebra 1, Geometry, Algebra 2, Introductory Statistics, and AP¨_ Statistics. Today he's hard at work creating new exercises and articles for AP¨_ Statistics.
Khan Academy is a nonprofit organization with the mission of providing a free, world-class education for anyone, anywhere. We offer quizzes, questions, instructional videos, and articles on a range of academic subjects, including math, biology, chemistry, physics, history, economics, finance, grammar, preschool learning, and more. We provide teachers with tools and data so they can help their students develop the skills, habits, and mindsets for success in school and beyond. Khan Academy has been translated into dozens of languages, and 15 million people around the globe learn on Khan Academy every month. As a 501(c)(3) nonprofit organization, we would love your help! Donate or volunteer today!
Probability with discrete random variable example | Random variables | AP Statistics | Khan Academy
Discrete and continuous random variables | Probability and Statistics | Khan Academy
Discrete Random Variables
02 - Random Variables and Discrete Probability Distributions
Understanding Discrete Random Variables and Probability Distributions
Discrete Random Variable : How to find probability from a CDF.
An Introduction to Discrete Random Variables and Discrete Probability Distributions
Introduction to Discrete Random Variables and Discrete Probability Distributions
Joint, Marginal and Conditional distributions (Week-1)
Random variables | Probability and Statistics | Khan Academy
Discrete Random Variables The Expected Value of X and VarX
Probability Distributions for Discrete Random Variables - Example
Expected Value and Variance of Discrete Random Variables
Discrete Random Variable and Probability Mass Function || Lesson 44 || Algorithms || Learning Monkey
Lec-17: Discrete And Continuous Random Variable | Probability and Statistics
Discrete Random Variables (1 of 3: Expected value & median)
Finding The Probability of a Binomial Distribution Plus Mean & Standard Deviation
DISCRETE PROBABILITY DISTRIBUTION: FINDING THE UNKNOWN PROBABILITIES.
Discrete random variable problem/ application of discrete random variable
Introductory Statistics: Discrete Random Variables; Probability Distributions (5.1 & 5.2)
L05.3 Probability Mass Functions
Discrete Random Variable : Finding the probability from PDF function.
Constructing a probability distribution for random variable | Khan Academy
L12.2 The Sum of Independent Discrete Random Variables
Комментарии
 0:03:50
0:03:50
 0:11:56
0:11:56
 0:11:29
0:11:29
 0:29:54
0:29:54
 0:08:41
0:08:41
 0:05:36
0:05:36
 0:14:11
0:14:11
 0:11:46
0:11:46
 2:05:15
2:05:15
 0:05:32
0:05:32
 0:05:33
0:05:33
 0:05:57
0:05:57
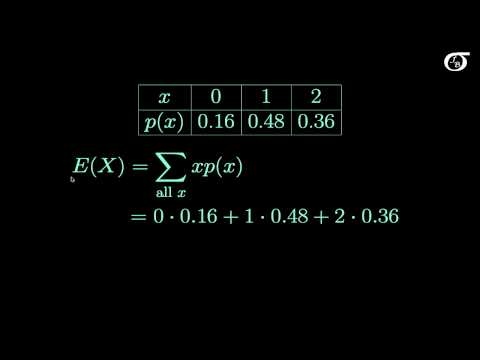 0:07:57
0:07:57
 0:07:36
0:07:36
 0:18:54
0:18:54
 0:09:37
0:09:37
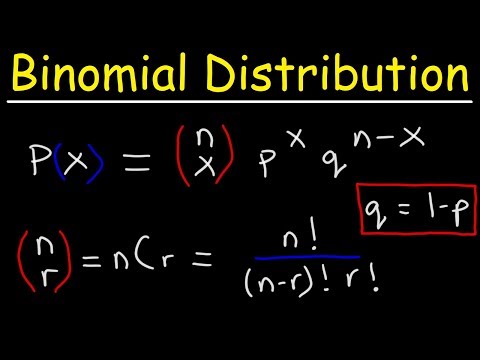 0:20:27
0:20:27
 0:05:29
0:05:29
 0:10:22
0:10:22
 0:39:30
0:39:30
 0:10:21
0:10:21
 0:05:48
0:05:48
 0:06:47
0:06:47
 0:07:52
0:07:52