L12.2 The Sum of Independent Discrete Random Variables

preview_player
Показать описание
MIT RES.6-012 Introduction to Probability, Spring 2018
Instructor: John Tsitsiklis

License: Creative Commons BY-NC-SA
  1. MIT OpenCourseWare
  2. Sum
  3. Independent Discrete Random Variables
Рекомендации по теме
L12.2 The Sum 0:07:52

L12.2 The Sum of Independent Discrete Random Variables

L12.3 The Sum 0:06:45

L12.3 The Sum of Independent Continuous Random Variables

L12.4 The Sum 0:03:10

L12.4 The Sum of Independent Normal Random Variables

Sum of Independent 0:07:24

Sum of Independent Binomial Random Variables

The PDF of 0:05:48

The PDF of the Sum of Two Independent Random Variables

Finding the Distribution 0:01:58

Finding the Distribution of a Sum of Independent Random Variables

L23.2 The Sum 0:04:03

L23.2 The Sum of Independent Poisson Random Variables

Interview Question: Sum 0:06:28

Interview Question: Sum of Two Uniform Random Variables

Sum of two 0:09:53

Sum of two independent random variables: Convolution.

Sum of Independent 0:06:56

Sum of Independent Normal Random Variables Proof

L12.7 The Variance 0:05:36

L12.7 The Variance of the Sum of Random Variables

Prob & Stats, 0:50:14

Prob & Stats, Lec 17A: Distribution of Sum of 2 Independent Random Variables (Exponential & ...

Sum of two 0:04:57

Sum of two independent exponentially distributed random variables

The Sum of 0:04:18

The Sum of Normal Random Variables with Moment Generating Functions

PGF - Sum 0:09:12

PGF - Sum Of Independent Random Variables

Sum of 2 0:02:59

Sum of 2 Binomial Random Variables Distribution (Independent)

[Chapter 6] #7 0:14:36

[Chapter 6] #7 Sum of two independent uniforms

Convolution in Probability: 0:06:10

Convolution in Probability: PDF of a Sum of Independent Random Variables (With Proof) [DSP #13]

Terms: Independent and 0:01:04

Terms: Independent and Identically Distributed (IID)

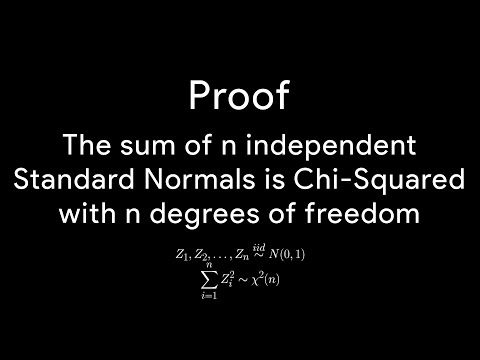
Proof: Sum of 0:10:59

Proof: Sum of Independent Standard Normals is Chi-Square

Sum of two 0:12:52

Sum of two random variables: uniform, exponential, normal distributions.With examples and code in R.

Sum of independent 0:05:35

Sum of independent Poisson random variables

Sum of two 0:27:25

Sum of two independent random variables - 3 MRK

Variance of the 0:03:56

Variance of the Sum of Independent Random Variables

Комментарии
Автор

I agree with Bill. This is the best explanation of the convolution formula I've seen on YouTube.

rakoonberry
Автор

This is a *GREAT* explanation of the Convolution equation and the Convolution calculation process, the best I have seen.
@MIT OpenCourseWare - I suggest you to include the words "Convolution Equation" in your lesson title; I believe many others are searching for this and will find this beneficial. *Thank you!*

billwindsor
Автор

The flipping is a very good and intuitive explanation for convolution. Thanks so much.

drgothmania
Автор

I'm a little confused as to *why* that flip-and-shift scheme works. 🤔 Could someone help clear that up for me?

ChrisOffner
Автор

0:45 You took an example of z=x+y=3. But in this case x and y are not independent. If we take x=1 then we are restricted to take y=2. And so P(x=1 and y=2) will not be equal to P(x=1)*P(y=2).
If I'm wrong let me know.

kmishy
Автор

if we have two dependent random variable how we calculate Z

kaankutlu