General Theory of nth-Order Linear Differential Equations (1 of 4)

This video is an introduction to the idea of differential equations with orders higher than 2. We discuss the form these equations take, and address the similarities between them and their lower-order cousins, including the existence and uniqueness theorem for the general case.

I've tried uploading this twice now, and the annotations I make from about 1:38 to 2:06 just don't show up. They're in the original, and you can see me erasing them at 2:05, but they just don't want to make an appearance, for some reason. For the record, I'm trying to distinguish between the notation y^4 and y^(4). The parentheses indicate the fourth derivative; the "no parentheses" version is the fourth power.

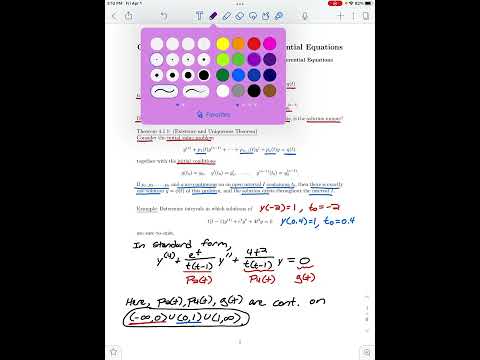
[10:32] In my description of the initial conditions for the nth-order equation, I have inadvertently left in the derivative markers. That line should read:
y(t0)=y0, y'(t0)=y1, ... y^(n-1)(t0)=yn.