filmov
tv
Diagonalize 3x3 matrix

Показать описание
Diagonalizing a 3x3 matrix. Finding eigenvalues and eigenvectors. Featuring the rational roots theorem and long division
Diagonalize a 3 by 3 Matrix (Full Process)
Diagonalize 3x3 matrix
Full Example: Diagonalizing a Matrix
Linear Algebra: Diagonalizing a 3x3 matrix
Diagonalizing a 3x3 matrix using eigenvectors
Linear Algebra: Diagonalize a matrix (3x3)
Diagonalization
Diagonalization of a 3x3 matrix
Diagonalize a 3x3 matrix
Diagonalization of matrices 3x3 | How to diagonalise the matrix with example | Linear algebra
DIAGONALISATION OF 3x3//STEP WISE EXPLANATION//TRICKS AND TIPS//MATHSPEDIA//
🔷15 - Eigenvalues and Eigenvectors of a 3x3 Matrix
Diagonalizing a Matrix
Eigenvalues of a 3x3 matrix | Alternate coordinate systems (bases) | Linear Algebra | Khan Academy
Diagonalizing a matrix with repeated eigenvalues
Orthogonal diagonalisation of symmetric 3x3 matrix using eigenvalues & normalised eigenvectors
Find the Diagonalization of a 3 by3 Matrix Given Eigenvectors and Eigenvalues
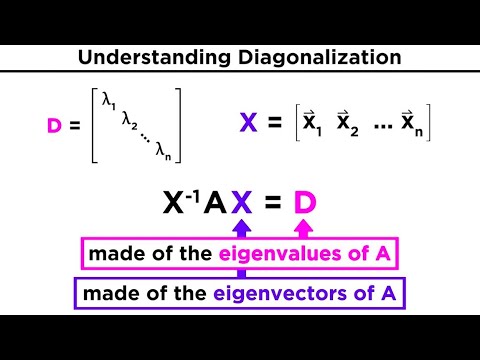
Visualizing Diagonalization
Diagonal Matrix: Diagonalization of Matrix | { 2024 } best method !!!
Diagonalization and power of a matrix
#Easy way ||how to diagonalize matrix ||
Linear Algebra Example Problems - Diagonalizing a Matrix
Diagonalization of a Matrix | Numerical | Matrices | Maths
Linear Algebra - Lecture 36 - Diagonalizing a Matrix
Комментарии
 0:08:48
0:08:48
 0:23:01
0:23:01
 0:10:08
0:10:08
 0:14:44
0:14:44
 0:10:03
0:10:03
 0:13:38
0:13:38
 0:08:43
0:08:43
 0:05:38
0:05:38
 0:03:48
0:03:48
 0:12:42
0:12:42
 0:19:16
0:19:16
 0:31:10
0:31:10
 0:11:37
0:11:37
 0:14:08
0:14:08
 0:08:50
0:08:50
 0:04:53
0:04:53
 0:03:33
0:03:33
 0:05:00
0:05:00
 0:22:39
0:22:39
 0:11:32
0:11:32
 0:00:16
0:00:16
 0:04:41
0:04:41
 0:10:53
0:10:53
 0:09:29
0:09:29