filmov
tv
Linear Algebra - Lecture 36 - Diagonalizing a Matrix
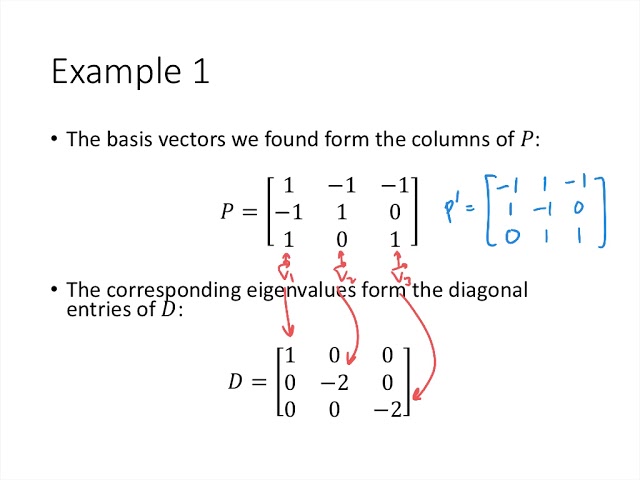
Показать описание
In this lecture, we work through some examples where we attempt to diagonalize a matrix. We also discuss a sufficient (but not necessary) condition for diagonalizability.
Linear Algebra - Lecture 36 - Diagonalizing a Matrix
Linear Algebra Lecture 36 | Properties of Linear Transformation
Linear Algebra 36 | Solving Systems of Linear Equations (Introduction)
Advanced Linear Algebra - Lecture 36: The Fundamental Matrix Subspaces from the SVD
Energy, momentum and linear algebra | Wild Linear Algebra B 36 | NJ Wildberger
Linear Algebra 36 | Solving Systems of Linear Equations (Introduction) [dark version]
Diagonalization of matrices example | Lecture 36 | Matrix Algebra for Engineers
UPSC Mathematics Optional (in Hindi) | Linear Algebra | Lecture 36
Gauss Jordan Method Question 6 Solution #matrices #gaussjordanmethod
Lecture 36: Alan Edelman and Julia Language
The Big Picture of Linear Algebra
Linear Algebra - Lecture 15 - Linear Independence
Algebra 36 - Solving Systems of Equations by Substitution
Linear Algebra: Ch 2 - Determinants (36 of 48) Find Determinant=? by Reducing to Echleon Form: 4
Geometry of Linear Algebra
Linear Algebra: Ch 2 - Determinants (22 of 48) The Cofactor of a Matrix
Linear Algebra Lecture 2: Examples of vector spaces
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
Linear Algebra | Rank of a matrix | Exercises | Lecture-36
Trying transition video for the first time 💙😂 || #transformation #transition #shorts #viralvideo...
Advanced Linear Algebra, Lecture 1.5: Dual vector spaces
Linear Algebra - Lecture 41 - Diagonalization of Symmetric Matrices
Linear Algebra 1: Systems of linear equations - Oxford Mathematics 1st Year Student Lecture
Linear Algebra - Lecture 28: The Span of a Set of Vectors
Комментарии
 0:09:29
0:09:29
 0:15:49
0:15:49
 0:09:28
0:09:28
 0:23:01
0:23:01
 0:46:03
0:46:03
 0:09:28
0:09:28
 0:15:03
0:15:03
 0:49:16
0:49:16
 0:16:43
0:16:43
 0:38:11
0:38:11
 0:15:57
0:15:57
 0:13:46
0:13:46
 0:06:10
0:06:10
 0:01:29
0:01:29
 0:16:36
0:16:36
 0:04:13
0:04:13
 0:36:39
0:36:39
 0:00:15
0:00:15
 0:27:57
0:27:57
 0:00:15
0:00:15
 0:23:36
0:23:36
 0:15:12
0:15:12
 0:51:36
0:51:36
 0:20:16
0:20:16