filmov
tv
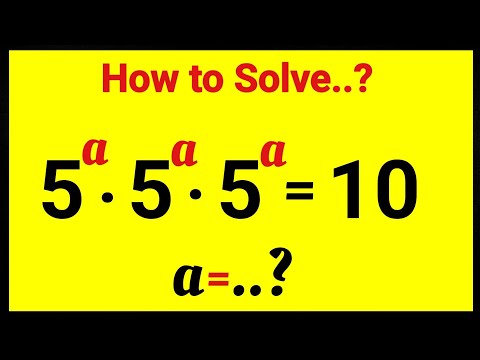
USA Olympiad Exponential Equation: solve for a=?

Показать описание
USA Olympiad Exponential Equation: solve for a=?
Hello My Dear Family😍😍😍
I hope you all are well 🤗🤗🤗
If you like this video about
How to solve this math problem
Please like and subscribe to my channel as it helps me a lot,🙏🙏🙏🙏
Explore the interesting world of exponential equations with varying bases and unlock the power of exponentiation! In this educational video, we delve into the concept of exponential equations where the base is not a constant value. Learn the fundamentals, discover the properties, and master solving equations with different bases, all explained clearly and concisely. Join us on this exponential journey and level up your mathematical prowess!
#exponentialequation #differentbases #exponentiation
#mathematicalproperties #increasingpower #solvingequations #variablebases #algebra #mathematics #mathematicalconcepts
#educationalvideo #exponentialequations
#tetrationequations #solvingequations #mathematics #mathtutorial #exponentialfunctions #complexequations#equationsolvingmethods #mathhelp #stepbystepguide #mathtechniques #mathematicstutorial #algebra #precalculus
#exponentialequations
#solvingexponentialequations
#solvingexponentialequationsusing logarithms
#solvingexponentialequationswithdifferent bases
#solvingexponentialequationswithe #solvingexponentialequationsusingnaturallogarithms properties of logarithms #naturallogarithms #factoringtrinomials #logarithms #examples #math #class #exponentialfunction #logarithm #tutorial #university #exam #free #online #university #test #prep #GRE #onlinecollege #test #solution #solve #ACT #onlineclass #help #tutor #SAT #equation #differentbases #collegealgebra #algebra2 #practice #problems #solveexponential #howtosolveexponentialequations #logs #equations #logssamebase #exponentialequatios
#solveexponentialequations #fractions #brianmclogan #exponentialequation#freemathvideos
#howtosolveexponetialequations #exponents #mathematics #solveexponentialequation
#solveequation #solvinganexponentialequation #solving #naturalexponents
Hello My Dear Family😍😍😍
I hope you all are well 🤗🤗🤗
If you like this video about
How to solve this math problem
Please like and subscribe to my channel as it helps me a lot,🙏🙏🙏🙏
Explore the interesting world of exponential equations with varying bases and unlock the power of exponentiation! In this educational video, we delve into the concept of exponential equations where the base is not a constant value. Learn the fundamentals, discover the properties, and master solving equations with different bases, all explained clearly and concisely. Join us on this exponential journey and level up your mathematical prowess!
#exponentialequation #differentbases #exponentiation
#mathematicalproperties #increasingpower #solvingequations #variablebases #algebra #mathematics #mathematicalconcepts
#educationalvideo #exponentialequations
#tetrationequations #solvingequations #mathematics #mathtutorial #exponentialfunctions #complexequations#equationsolvingmethods #mathhelp #stepbystepguide #mathtechniques #mathematicstutorial #algebra #precalculus
#exponentialequations
#solvingexponentialequations
#solvingexponentialequationsusing logarithms
#solvingexponentialequationswithdifferent bases
#solvingexponentialequationswithe #solvingexponentialequationsusingnaturallogarithms properties of logarithms #naturallogarithms #factoringtrinomials #logarithms #examples #math #class #exponentialfunction #logarithm #tutorial #university #exam #free #online #university #test #prep #GRE #onlinecollege #test #solution #solve #ACT #onlineclass #help #tutor #SAT #equation #differentbases #collegealgebra #algebra2 #practice #problems #solveexponential #howtosolveexponentialequations #logs #equations #logssamebase #exponentialequatios
#solveexponentialequations #fractions #brianmclogan #exponentialequation#freemathvideos
#howtosolveexponetialequations #exponents #mathematics #solveexponentialequation
#solveequation #solvinganexponentialequation #solving #naturalexponents
Комментарии
 0:08:16
0:08:16
 0:08:05
0:08:05
 0:08:45
0:08:45
 0:09:22
0:09:22
 0:08:19
0:08:19
 0:06:53
0:06:53
 0:07:29
0:07:29
 0:08:05
0:08:05
 0:07:03
0:07:03
 0:05:53
0:05:53
 0:07:17
0:07:17
 0:08:03
0:08:03
 0:14:46
0:14:46
 0:02:34
0:02:34
 0:01:34
0:01:34
 0:19:27
0:19:27
 0:09:01
0:09:01
 0:05:49
0:05:49
 0:11:47
0:11:47
 0:08:04
0:08:04
 0:08:20
0:08:20
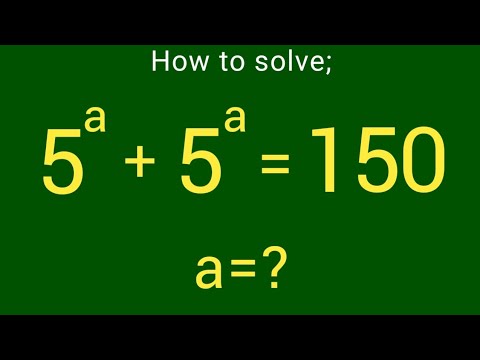 0:08:02
0:08:02
 0:11:38
0:11:38
 0:08:30
0:08:30