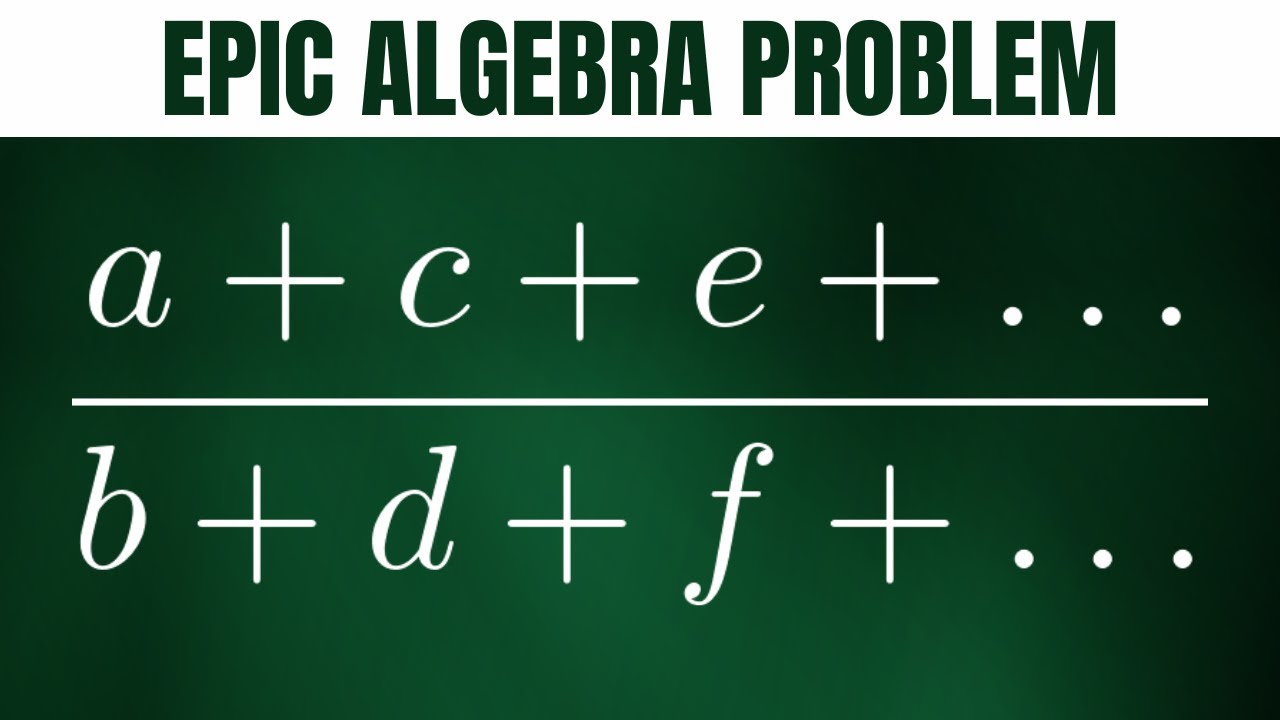
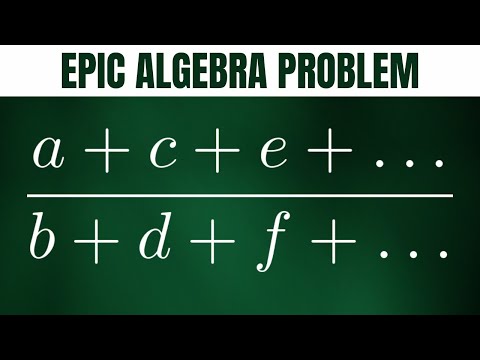
Prove if a/b = c/d = e/f = ... then (a + c + e + ...)/(b + d + f + ...)

Показать описание

Prove if a/b = c/d = e/f = ... then (a + c + e + ...)/(b + d + f + ...)

If you enjoyed this video please consider liking, sharing, and subscribing.

There are several ways that you can help support my channel:)

************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course

Advanced Calculus Course

Calculus 1 Course

Calculus 2 Course

Calculus 3 Course

Calculus 1 Lectures with Assignments and a Final Exam

Calculus Integration Insanity

Differential Equations Course

Differential Equations Lectures Course (Includes Assignments + Final Exam)

College Algebra Course

How to Write Proofs with Sets Course

How to Write Proofs with Functions Course

Trigonometry 1 Course

Trigonometry 2 Course

Statistics with StatCrunch Course

Math Graduate Programs, Applying, Advice, Motivation

Daily Devotionals for Motivation with The Math Sorcerer

Thank you:)

Рекомендации по теме

Комментарии

Here's how I like to think about it (not a proof just a cool intuition):

Suppose you have a line in the xy plane which intersects the origin. Now suppose that the point (b, a) is located on the line - this means that the slope of the line is a/b (rise/run).

Now, if you move from point (b, a) to a new point (b + d, a + c), we know that this new point must lie on the original line since c/d = a/b (for every d units moved right, we moved c units up, thus obeying the slope of the line).

Therefore, since the new point (b + d, a + c) lies on the same original line, the slope of the line, a/b, is also equal to (a + c)/(b + d). The process can be repeated (moving from (b + d, a + c) to (b + d + f + ..., a + c + e + ...)) and as long as the slope of each of those "steps" is equal to the original slope (a/b = c/d = e/f), each consecutive point will lie on the original line and therefore (a + c + e + ...)/(b + d + f + ...) will be equal to the original slope a/b.

jaeheonshim

Thanks, I from "Elementary results in puré mathematics" of G.S Carr

josevasconcelos

Please sir can you make a video about all the math lessons in order for the university because most of us are lost where to star after high school

hamza_yt

Super video, Also I love your thumbails

Iamblindanddeaf

Can you make a review for higher algebra by hall and knight sir please? Thanks in advance

mathpassion

Could guess that it's from Hall and Knight. A similar problem was shown a few days back.
Thank you 🙏

sureshnair

Hi math sorcerer! here is my solution
step 1 - factor a/b from the fraction: (I will do this for numerator and denominator separately)
numerator: a+c+e+... -> a(1 + c/a + e/a +...)
denominator: b+d+f+... -> b(1 + d/b + f/b +...)
step 2: with simple manipulations of the ratios you see that d/b=c/a, that f/b=e/a and this extends to all the other ratios

step 3: notice that (1 + c/a + e/a +...)=(1 + d/b + f/b +...) so dividing them equals 1, leaving only the a/b
QED

themibo

It's a feedback, where you go in reverse

jameyatesmauriat

Prove if a/b = c/d = e/f = ... then (a + c + e + ...)/(b + d + f + ...)

If a/b=c/d then a/b=(a+c)/(b+d) mathematical proof

Prove if a/b = c/d = e/f = ... then (a + c + e + ...)/(b + d + f + ...)

Divisibility Proof with the Sorcerer: a|b and a|c implies a|(b + c)

Number Theory Proof: If a|b and b|a then a = b or a = -b

Direct Proof: If a|b and b|c, then a|c

Prove that if a/b = c/d = e/f then (a^3b + 2c^2e - 3ae^2f)/(b^4 + 2d^2f - 3bf^3) = (ace)/(bdf)

How to Prove Two Sets are Equal: (Prove if A x C = B x C then A = B)

Proof: Divides Relation is Transitive (if a divides b and b divides c then a divides c)

Componendo and Dividendo Proof || (a+b)/(a-b)=(c+d)/(c-d)

Given (a,b)R(c,d) iff ad=bc show R forms an equivalence relation on RxR

If a and b are co-primes then show that gcd of a-b and a+b equals 1 or 2

If a,b,c are in AP. Show thata(b+c)/bc, b(c+a)/ca, c(a+b)/ab are in AP | Arithmetic Progression

If a divides c and b divides c with gcd(a,b)=1 then ab will divide c

Set Theory Proof A is a subset of B if and only if A intersect B = A

Set Theory Proof: A subset of B and C subset of D then A x C is a subset of B x D

Proof: A is a Subset of B iff A Union B Equals B | Set Theory, Subsets

If `(a+b)/(b+c) = (c +d)/(d + a)` , that prove that ` c = a ' or, ' a + b + c + d = 0`.

If a divides b then a divides bx for all Integers x Proof

If a^x=b,b^y=c & c^z=a, prove that xyz=1.| indices | class10

Congruence Modulo n Addition Proof

If a, b, c and d are in G.P. show that (a^2+b^2+c^2)(b^2+c^2+d^2)=(a b+b c+c d)^2 . a, b, c, d → ......

Let a, b, c and d be positive rationals such that a+√b = c+√d, then prove that either a=c and b=d or...

If a, b, c and d are in G.P. show that (a^2 + b^2 + c^2)(b^2 + c^2 + d^2) = (ab + bc + cd)^2.

If a ≡ b (mod m ) , then prove that a^n ≡ b^n (mod m) || Property of Congruence || Number theory....