Given (a,b)R(c,d) iff ad=bc show R forms an equivalence relation on RxR

preview_player
Показать описание

  1. Ms Shaws Math Class
Рекомендации по теме
Given (a,b)R(c,d) iff 0:06:57

Given (a,b)R(c,d) iff ad=bc show R forms an equivalence relation on RxR

Let N denote 0:13:09

Let N denote the set of all natural numbers and R be the relation on n×n defined by (a b)r(c d)

R: N×N - 0:17:09

R: N×N - N×N defined by (a,b)R(c,d) if ad(b+c)=bc(a+d) | Show that R is an equivalence | Izhar Sir |...

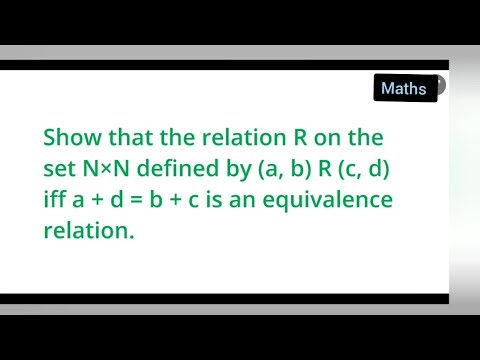
Show that the 0:08:05

Show that the relation R on the set N×N defined by (a, b)R(c, d)iff a+d=b+c is equivalence relation....

In N x 0:09:42

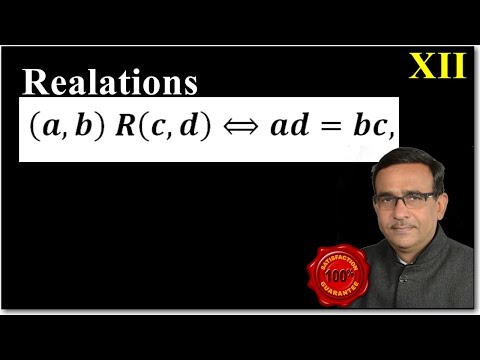
In N x N ,show that the relation defined by (a, b) R (c, d) if and only if ad = bc is an equivalence

Class 12 maths 0:16:26

Class 12 maths (a,b) R (c,d) ⟺ad=bc,for all a,b,c,d∈A×A Show that R is an equivalence relation

Let A={1,2,3,………9} and 0:15:29

Let A={1,2,3,………9} and R be the relation defined on AxA by (a,b)R(c,d) iff a+d=b+c | Prove that R is...

Prove that the 0:05:06

Prove that the relation R on the set `NxN` defined by `(a , b)R(c , d) iff a+d=b+c` for all `(a ...

Show that the 0:07:27

Show that the relation R defined by (a, b) R(c, d) if a+d=b+c on set N*N is an equivalence.

Proof: Divides Relation 0:05:19

Proof: Divides Relation is Transitive (if a divides b and b divides c then a divides c)

Let A = 0:09:37

Let A = {1,2,... 9} and R be the relation in A×A defined by (a,b) R (c,d) if a+d=b+c for (a,b),...

Let A = 0:00:58

Let A = NxN be the set of all ordered pairs of natural numbers (a, b) R ( c, d) iff ad=bc.

Given aRb iff 0:02:37

Given aRb iff ab is greater or equal to 0 explain why R is not an equivalence relation on set N

Statement-1: The relation 0:07:30

Statement-1: The relation R on the set `N xx N` defined by (a, b) R (c, d) `iff` a+d = b+c for all

AD and BC 0:06:07

AD and BC Explained (as well as CE and BCE)

Prove that the 0:40:12

Prove that the relation R on the set NxN defined by (a , b)R(c , d) ⇌ a+d=b+c for all (a , b),(c ,...

In N × 0:09:49

In N × N , Show that the relation defined by (a, b) R (c,d) iff ad= cd is an equivalence relation.

Question Relation R 0:09:30

Question Relation R = (a,b) R (c,d) ad(b+c)=bc(a+d). Prove Equivalence , Vid 24

Equivalence Relation 0:06:29

Equivalence Relation

ABCD Nursery Rhyme 0:07:06

ABCD Nursery Rhyme | English Alphabet Rhyme | Alphabet Songs | Nursery Rhymes | ABC Cartoon Song

Let N denote 0:13:28

Let N denote the set of all natural numbers and R be the relation on NxN | defined by (a,b) R(c,d)

Divisibility Proof with 0:02:57

Divisibility Proof with the Sorcerer: a|b and a|c implies a|(b + c)

Q33. Let N 0:09:19

Q33. Let N be the set of all natural numbers and R be a relation on N × N defined (a,b)R(c,d) Leftri...

If a/b=c/d then 0:03:57

If a/b=c/d then a/b=(a+c)/(b+d) mathematical proof

Комментарии
Автор

Character In the video It's great, I like it a lot $$

dungletrung