Linear Algebra for Computer Scientists. 13. Transformation Matrices

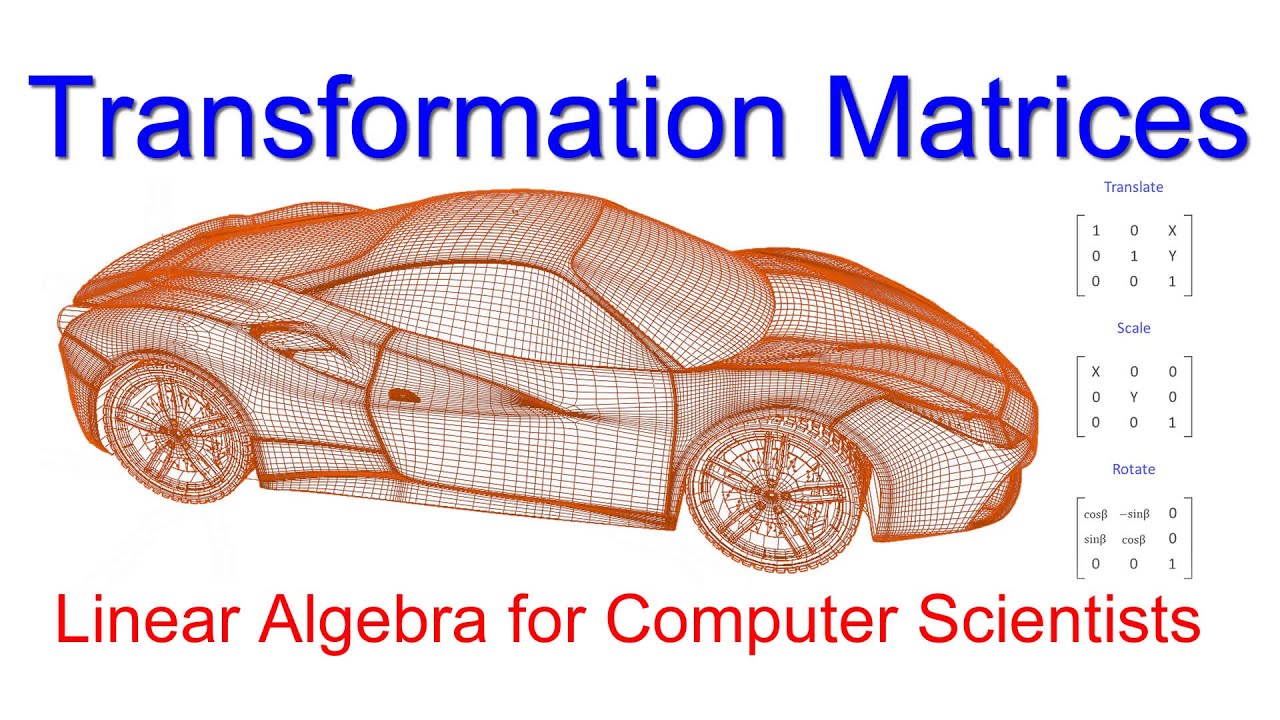
Animated computer graphics are based on models composed of thousands of tiny primitive shapes such as triangles, and each vertex in a model is encoded as a vector. This computer science video demonstrates how matrices are used to transform these vector. In particular you will learn how to derive a two dimensional rotation matrix using trigonometric identities, and how to transform a model in two dimensional space with a scaling matrix, a translation matrix and a rotation matrix. You will also learn how several transformation matrices can be combined using matrix multiplication to create a single transformation matrix that encodes multiple transformations at once.

Chapters:
00:00 Introduction to 3D computer models
00:50 Scale a vector with a vector
02:10 Translate a vector with a vector
02:40 Derive a rotation matrix using trigonometric identities
07:42 Rotate a vector with a matrix
08:48 Scale a vector with a matrix
09:32 Scale and rotate a vector with a single matrix
10:42 2D translation matrices
13:06 Translation, rotation and scaling combined
15:05 The role of a graphics processing unit (GPU)

[andysantosa]

It's a very underated channel, I was lucky to find it, and finally understand about matrices usage. Thank you for sharing 🙏

[roimordechay]

You are a genius. really. There are very few people who can explain so well as you do. Thx.

[CASLOAcademy]

you are the only one who can make apparent complex topics seems simple! you are great

[SuperOnlyP]

the rotation matrix using trigonometry pRT is really realy great ! Finally I can undertand how sin, cosin is applied to the transformation as rotation.

[emreiris]

Very informative and clear sir. Thank you so much

[wccuwoc]

A gem for us computer scientists, thank you so much for the work put in these series!❤

[simaobonvalot]

Thank you sir.

This is gold!

I'am building my ray tracing program and I need to rotate the camera and I didn't know.

Hopefully I will be able to apply this !

[aayushbajaj]

My good sir you blew my mind so many times in this video and even more times throughout the rest of the series. thank you for this 🙏

[Flaystray]

1:51 this is actually multiplying the vectors by a scalar of 2. Mtilkying by a [ 2, 2] vevtor would yield scalars

[marcosgoldin]

Great explanation. According to the example in 13:30, shouldn't the order of the product of matrices be first the translation matrix and then the rotacion matrix?

[dean-orochester]

Great video... at the end you combined the Rotate, Translate and Scale into one matrix. Question, Can I take the coords you have 3, 2 4, 5 2, 5 and then first rotate them 90degrees, then take those coordinate values and translate them the 1, 1. and then take those values and do the scaling of .5, .5 and get the same result as if I calculated the combined matrix from the result of Rotate times Translate and take that product matrix and then that the scale matrix and mulitply it by the product matrix? Thanks in advance for your assistance

[saraswathigantapakagantapa]

Could you please tell the reason for exchanging the positions of sin and cos in the equation of y1 for rotation?

[urimtefiki]

Start with my algorithm in the beginning then tell people about vectors, this is failing the whole picture.

[Remixt]

what software did you use to make your graphics?