The Exponential Distribution and Exponential Random Variables | Probability Theory

What is the exponential distribution? This is one of the most common continuous probability distributions. We'll go over an introduction of the exponential distribution and exponentially distributed random variables in today's probability theory video lesson.

The exponential distribution is often used to model the time until a particular event occurs, or the time between events. We'll see a couple examples of this in the lesson.

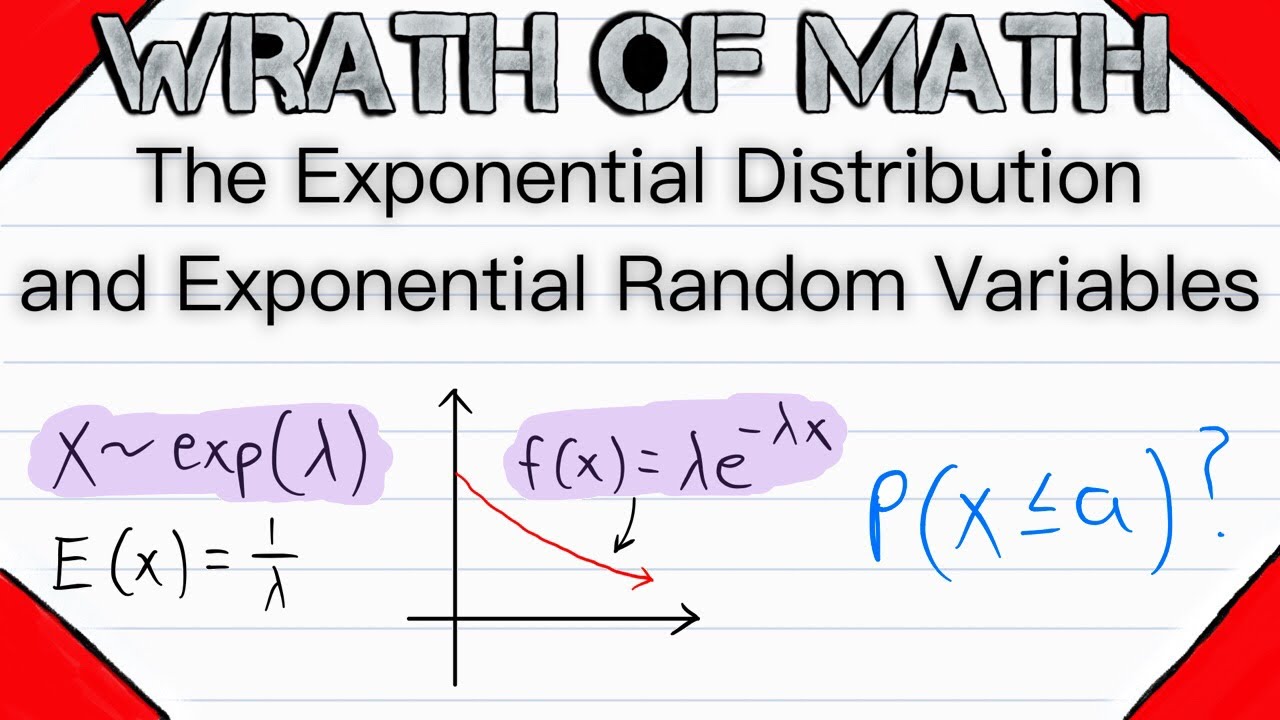
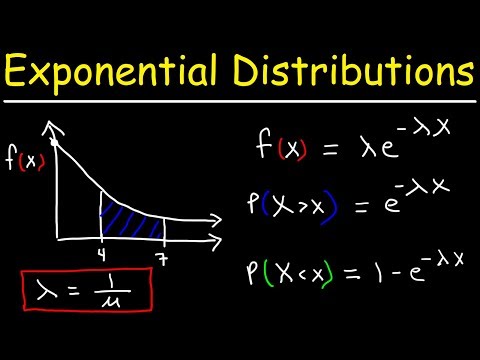
Remember that continuous probability distributions have probability density functions (PDF), which don't give actual probabilities. The PDF of the exponential distribution is f(x) = \lambda*e^{-\lambda*x} when x is greater than or equal to 0. Note that "\lambda" is our way of writing the greek character lambda here in a YouTube description where we cannot actually write lambda. When x is less than 0, f(x) = 0, so there is no probability in an exponential distribution for negative values of x.

More interesting than the PDF of a continuous probability distribution is the cumulative distribution function (CDF), which gives actual probabilities! Let X be an exponential distributed random variable with parameter lambda. The CDF of the exponential distribution, evaluated at a, is 1 - e^{-\lambda*a}. This is the probability that X is less than or equal to a. We'll prove the CDF of the exponential distribution using the definition of CDF in this lesson, and we'll go over some CDF example problems!

We also go over the expected value, or mean, of an exponential distribution, as well as the variance and the standard deviation. 

=============================================================
SOLUTION TO PRACTICE PROBLEM:

Let X be the amount of time a husband spends shopping for an anniversary card. Thus, X is exponential with mean 9 minutes. Hence, it's parameter is the inverse: 1/9. So the pdf is, for x greater than or equal to 0, f(x) = -(1/9)*e^{-(1/9)*x}. 

The CDF is F(a) = 1 - e^{-(1/9)*a}.

The probability that a husband spends between 2 and 4 minutes shopping for an anniversary card is the probability that X is between 2 and 4, which is F(4) - F(2) = ( 1 - e^{-(4/9)} ) - ( 1 - e^{-(2/9)} ) = e^{-2/9} - e^{-4/9} which is roughly 16%. 

=============================================================

The example problem we went over in the video was adapted from "A First Course in Probability Theory" by Sheldon Ross, the first textbook I used to learn probability theory! Check out the book and see if it suits your needs! You can purchase the textbook using the affiliate link below which costs you nothing extra and helps support Wrath of Math!

********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.

********************************************************************

+WRATH OF MATH+

Follow Wrath of Math on...

[James]

Great recap. It really helped me piece a bunch of stuff together

[beefandpotatoes]

after many years I understood why in an exponentially distributed continues random variable probability of x >= a is e ^ (- ax ). Thank you man.

[abhishekroy]

Thanks for making the video.
It really helps me to understand the basics in actuarial field.

[jeffreya.faulkner]

I like to think of lambda as the rate parameter and mu as the "wait" parameter. Lambda = 1/5 would be one call per five minutes; mu = 5/1 would be five minutes per one call.

[cbkjskj]

I know this was published three years ago but could you do a video about poisson to exponential distributions

[Shaan_Suri]

Whats the difference between a probability density function and probability mass function ?

[miracleodion]

Your video on exponential distribution is helpful.i need your help on this particular question

Suppose that the time between emergency calls in a fire station follows the exponential distribution with an average rate of 2 calls per day.what is the probability that the firemen are not called in 3 days?

[nouramohammad]

i have a question, i can't solve


Let X ~ Exponential (lambda), and Y=aX, where a is a positive real number. Show that Y~ Exponential (lambda/a).

[omarsaad]

Hello Sir,
1. A system consists of three processors and four peripheral units. In each of these
cases find the reliability of the system if the processor lifetimes are exponential
random variable with mean 10 and the peripheral lifetimes are exponential random
variable with mean 15.
a) The system is functioning as long as one processor and one peripheral are
functioning.
b) The system is functioning as long as two processors and two peripherals
are functioning.

Any suggestions pls?

[zack_]

5:40 - are you joking or serious: σ=1/λ=E(x), meaning μ=σ (!?) since μ=1/λ.