Exponential Distribution

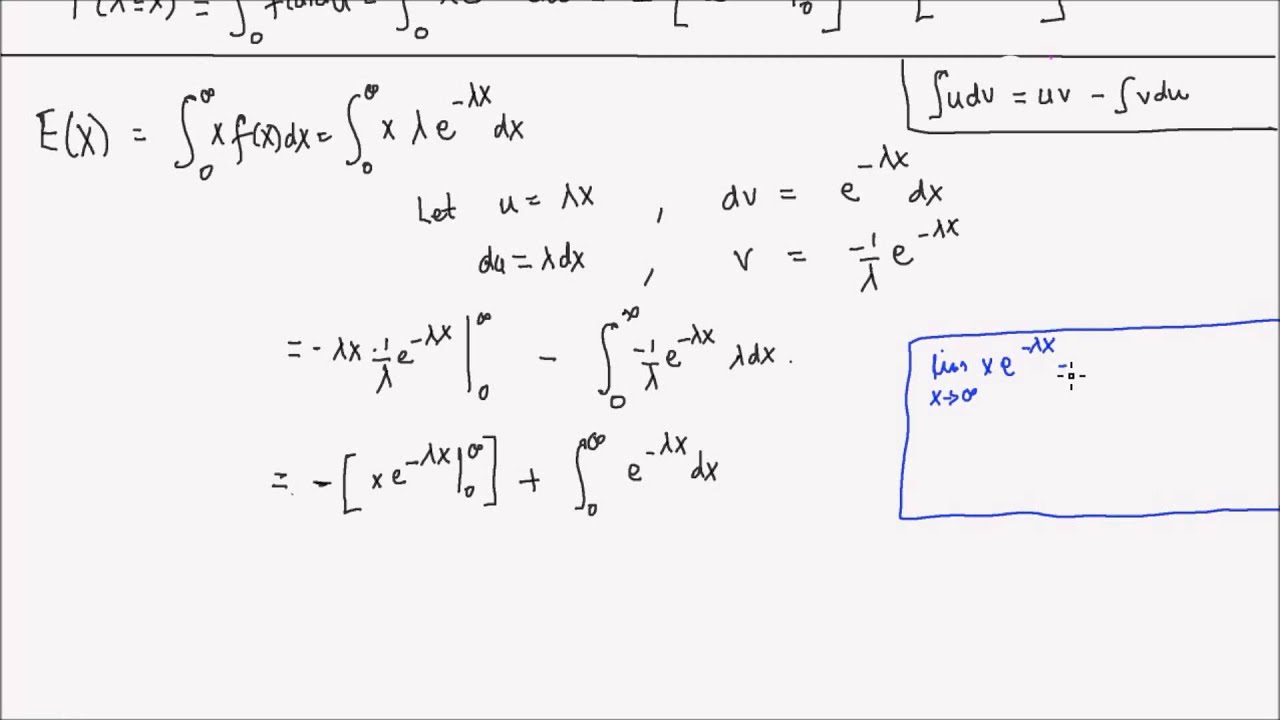
In this lesson we introduce the exponential distribution, derive its expected value, variance, moment generating function, and describe that it has "Meomryless" Property.

[somethingironical]

Thanks so much for posting up these videos. They are an incredibly helpful supplement, and you have a really clear way of teaching things.... I am really looking forward to some new videos soon!

[brianmccullough]

great video helped me a lot looking back on my lecture notes while doing my homework problem set

[ConnerDKnox]

Sure! If you don't do the trick that he does, the integral is 1/lambda*e^(-lambda*x) evaluated from 0 to infinity. The limit of that function as x approaches infinity is 0, which we see from it approaching 1/infinity. We must then evaluate the lower limit and *subtract* it (I think this may be where you made a mistake) and we end up subtracting -1/lambda(1/e^0) and since we are subtracting a negative it becomes just 1/lamda*1 = 1/lambda. Hope that helps!

[ajwilhelm]

I am curious to know if you are planning on putting any more of these videos out any time in the near future.

[akothjackline]

kudozz am very greatful hope for more under distibutions

[StatCourses]

The integral at 10:49 is the same as the one solved between 1:48 and 2:56 .

[leobrook]

can you further show how exponential distribution can be written as a member of exponential family

[LycoanOfSeven]

O.o Yeah I think I forgot to bring in the negative sign or I reversed the terms and took 1/lambda[(-1) - 0]. Everything makes sense now. Thanks~! :D

[grimlazy]

at 9:00, what will be the result for [X/e^(lambda*X)] when limits are from negative infinity to zero??

[yogeshagnihotri]

Sir make video on hypergeometric distribution

[mashhoodahmad]

Dear Sir Please tell how can integrate of Likelihood function of exponential dist.

[LycoanOfSeven]

sorry my math is a little bit rusty but could someone tell me why at 10:49 isn't the result negative one over lambda? I thought when you integrate (e to the power of a negative constant times x) the result should be (e to the power of a negative constant times x over the negative constant)? Sorry for the messy question but it's kinda hard asking a math question in comment. :)

[ajwilhelm]

Ahh the mathematician in me really hates you skipping the limits in your improper integral...