filmov
tv
Arc Length (formula explained)

Показать описание
Arc length integral formula,
blackpenredpen,
math for fun
blackpenredpen,
math for fun
Arc Length (formula explained)
Arc length intro | Applications of definite integrals | AP Calculus BC | Khan Academy
I want to show you how to prove the arc length formula
Arc Length Calculus Problems,
Arclength Formula | Derivation & Ex: Circumference of a Circle
How long is a curve?? The Arclength Formula in 3D
Arc Length (Formula)
Arc Length Derivation and First Example
How do we Find the Length of an Arc? | Circles | Don't Memorise
Why Students Struggle With Arc Length and How to Help
Arc Length of a Circle Formula - Sector Area, Examples, Radians, In Terms of Pi, Trigonometry
Arc Length Formula and Sector Area Formula Explained!
A Derivation of the Arc Length Formula
Find the Arc Length of a Function with Calculus
Deriving the Arc Length Formula in Calculus
Arc Length of Parametric Curves
Curves, Parameterizations, and the Arclength Parameterization
Arc Length Formula Explained
7. Deriving a formula for finding an arc length of a circle.
Radians as ratio of arc length to radius | Circles | High school geometry | Khan Academy
Multivariable Calculus | ArcLength Example
How to Calculate Arc Length? | Arc Length Formula | Basic Formulas in Geometry | Mathemetics
Deriving the Arc Length in Cartesian and Polar Coordinates
Arc Length & Surface Area examples
Комментарии
 0:07:57
0:07:57
 0:06:21
0:06:21
 0:01:25
0:01:25
 0:30:47
0:30:47
 0:10:13
0:10:13
 0:07:10
0:07:10
 0:01:11
0:01:11
 0:03:08
0:03:08
 0:03:35
0:03:35
 0:09:57
0:09:57
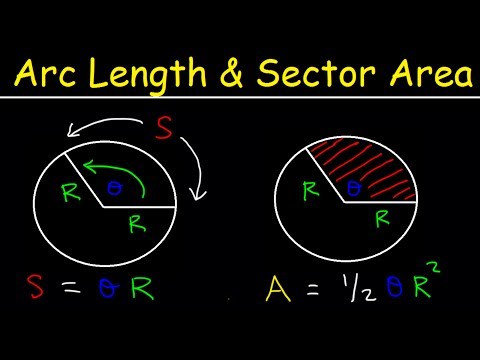 0:15:57
0:15:57
 0:05:35
0:05:35
 0:06:53
0:06:53
 0:16:21
0:16:21
 0:16:37
0:16:37
 0:12:34
0:12:34
 0:10:04
0:10:04
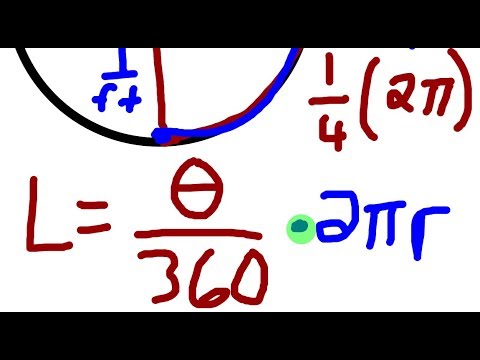 0:05:22
0:05:22
 0:01:13
0:01:13
 0:06:40
0:06:40
 0:04:10
0:04:10
 0:00:45
0:00:45
 0:13:48
0:13:48
 0:17:35
0:17:35