filmov
tv
This simple Hack will help you solve this problem in Seconds? | NO Calculators Allowed
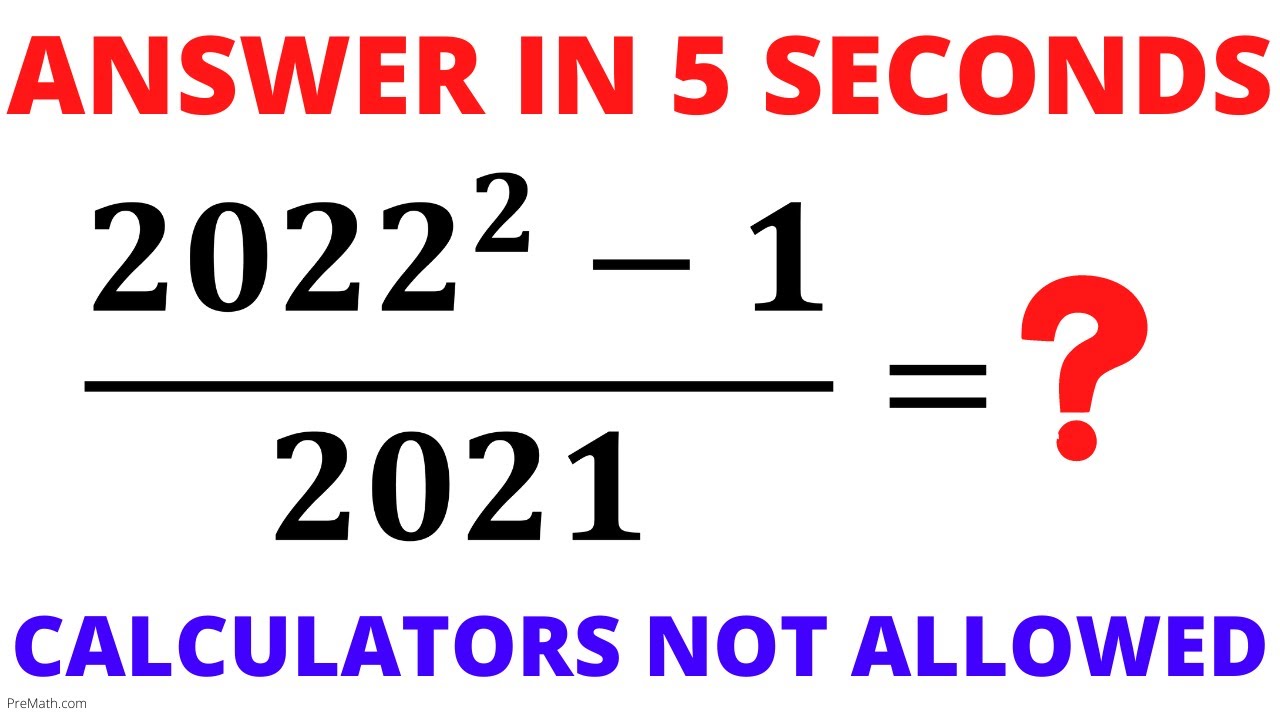
Показать описание
This simple hack will make every trip 𝗦𝗠𝗢𝗢𝗧𝗛𝗘𝗥 ✈️🔑
Have you ever tried this?? Nutella Food Hack
Simple HACK for More #Shorts Views
This simple hack will make your plants look unreal 😳
This simple hack will keep pesky birds away from your home
I Ruined My Skin with this Beauty Hack... #shorts #skincare
Kitchen hack is easy and clean! 🍅
Easiest messy bun hack EVER 🙌
HAIR WASHING HACK THAT WILL SAVE YOUR HAIR #shorts #YouTubePartner #haircareroutine
Quick and Easy Can Opening Hack! 🥫😂
Cleaning Hack!?!
Can A Flipper Zero Hack A PHONE? #Shorts
EASY HACK FOR BIGGER FOREARMS
Life hack for a flexible back (may require a friend) #shorts
Chocolate milk hack #shorts
How to be more LIKEABLE at work (One Simple Hack)
French tip hack✨
This Art Hack Will Save You HUNDREDS💸#shorts
PERFECT Slices Made EASY with This Genius Candy Hack!
How to hack WebCams
Pencil Grasp Hack For Better Handwriting | Credits: @7DaysofPlay
Stop Losing Your Tools! Try This Easy Paracord Wrench Hack! #shorts
Can The Flipper Zero HACK A TESLA? #shorts
Learning to Hack as a Kid
Комментарии
 0:00:22
0:00:22
 0:00:16
0:00:16
 0:00:29
0:00:29
 0:00:12
0:00:12
 0:01:14
0:01:14
 0:00:26
0:00:26
 0:01:00
0:01:00
 0:00:21
0:00:21
 0:00:35
0:00:35
 0:00:23
0:00:23
 0:00:53
0:00:53
 0:00:44
0:00:44
 0:00:25
0:00:25
 0:00:11
0:00:11
 0:00:10
0:00:10
 0:00:59
0:00:59
 0:00:51
0:00:51
 0:00:37
0:00:37
 0:00:21
0:00:21
 0:00:37
0:00:37
 0:00:16
0:00:16
 0:00:28
0:00:28
 0:00:34
0:00:34
 0:05:03
0:05:03