Bernoulli's Water Tank | Calculate Discharge Velocity

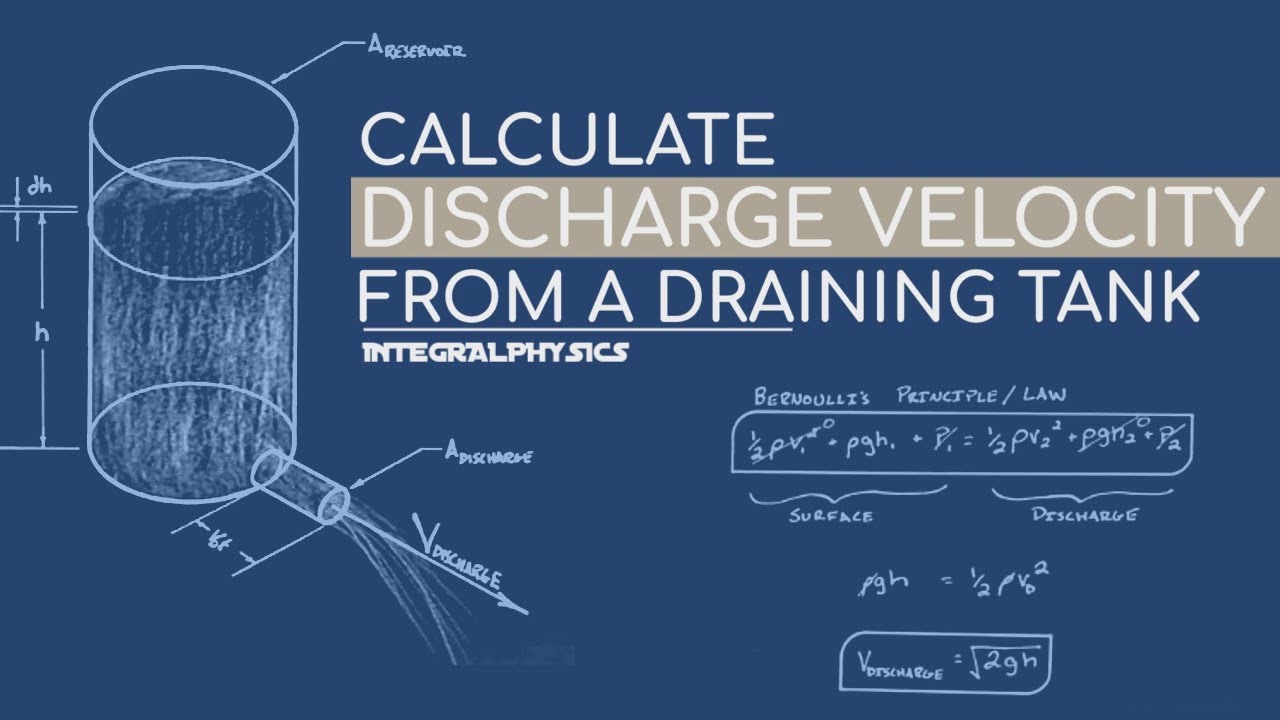
Use Bernoulli's Law to solve for the discharge velocity of a frictionless (inviscid) fluid as it exits a reservoir which is some height h deep.

The reservoir is commonly presented as a tank, or a bucket with some sort of hole or spigot for the fluid (typically water) to drain from. We use Bernoullis Equation to solve for the fluid speed as it exits the hole in the tank, bucket or reservoir.

Stick around for Part 2 of this video where we will take a look at the total time it will take this tank to drain.

This problem typically shows up in college physics, engineering, and fluid mechanics courses. It appears in Project Lead the Way, Principles of Engineering, AP Physics and some high school physics curriculums.

[mariamgirgis]

What if there are two holes? Is it correct to use Bernoulli's principle on each hole separately, or do we say that energies at the surface will equal sum of energies at both holes?

[Anonymous-qqyy]

Sir,

According to Bernoulli equation the discharge velocity is irrespective of the area of outlet. But practically, when we put a finger in front of a hose, the velocity increases


Please answer fast 🙏

[dawsonbrown]

Do the same rules apply with a slanted bottom?

[oliverfebruary]

Does the diameter of the exit hole/pipe matter?

[nirmalrajoshi]

What is the max velocity possible from that hole if we keep on increasing the tank height. Say 500 meters height. Will velocity reach 99 m/s?