filmov
tv
Why Does Fluid Pressure Decrease and Velocity Increase in a Tapering Pipe?
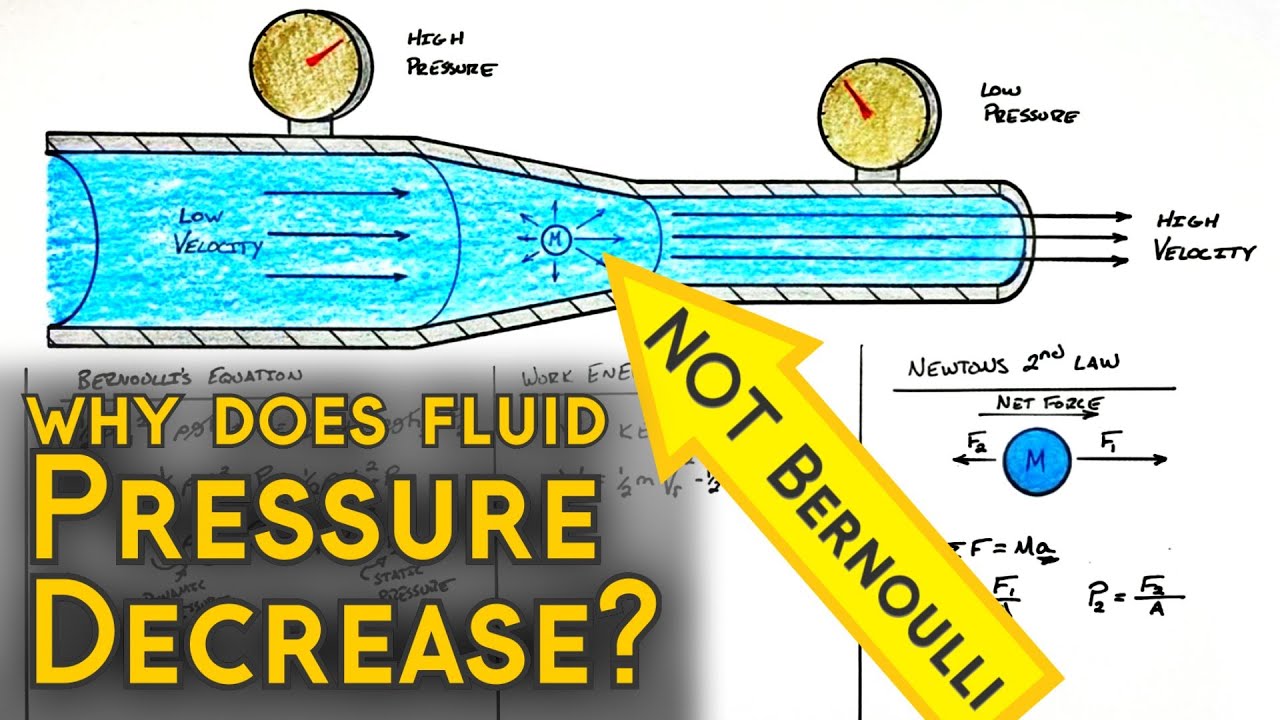
Показать описание
Bernoulli's Equation vs Newton's Laws in a Venturi
Often people (incorrectly) think that the decreasing diameter of a pipe correlates to a smaller volume and thus higher pressure. But the ideal gas law has no place in INCOMPRESSIBLE fluid flow.
Others try to explain the decrease in static pressure using Bernoulli's Principle, however, unless you have a VERY good handle on Bernoulli's, that is mostly a self supporting argument.
Back all the way up to Newton's 2nd Law to understand why there is a decrease in pressure in a pipe taper.
Often people (incorrectly) think that the decreasing diameter of a pipe correlates to a smaller volume and thus higher pressure. But the ideal gas law has no place in INCOMPRESSIBLE fluid flow.
Others try to explain the decrease in static pressure using Bernoulli's Principle, however, unless you have a VERY good handle on Bernoulli's, that is mostly a self supporting argument.
Back all the way up to Newton's 2nd Law to understand why there is a decrease in pressure in a pipe taper.
Why Does Fluid Pressure Decrease and Velocity Increase in a Tapering Pipe?
What is Head Loss? Pressure Drop? Pressure Loss? ( Fluid Animation)
Flow and Pressure in Pipes Explained
Does fluid always flow from high pressure to low pressure? | Quick Concepts
Siphon Theory - The fluid flows from high pressure to low pressure
Fluid Mechanics: Topic 8.3 - Pressure drop and head loss in pipe flow
The Four Influences of Pressure Drop in Fluid Systems
Calculate Change in Fluid Velocity & Pressure in a Tapering Pipe | Bernoulli's Law
HIGH PRESSURE RTR RTP LESSON LEARNT ?? TOP IMPORTANT FOR THE NEWLY IMPLEMENTED TECHNOLOGY IN GULF.
Pressure drop attributed to valves é fittings - Hydraulic calculation & fluid mechanics WR Train...
Pressure Confusion? Hydrostatic vs Static vs Dynamic vs Stagnation Pressure? on Fluid Dynamics.
Why Does Fluid Speed Up as it Moves Into a Narrower Pipe? | Continuity | Fluid Mechanics
Why PRESSURE is inversely proportional to the VELOCITY of a dynamic fluid? | Physics | zorthus-2020
Pressure in Gases | Matter | Physics | FuseSchool
Cerebrospinal Fluid CSF Leaks Symptoms | Cedars-Sinai
Boiling Water Without Heat | Earth Science
Diuretics for Hypertension (high blood pressure), Heart Failure, or Fluid Overload
Plotting the pressure distribution in a pipe with bends (Fluid Dynamics with Olivier Cleynen)
Poiseuille's Law - Pressure Difference, Volume Flow Rate, Fluid Power Physics Problems
Introductory Fluid Mechanics L18 p1 - Example: Pressure Drop Laminar Pipe Flow
What is Water Hammer?
Fluid Power: Pneumatic Air Pressure Losses in Pipes and Fittings
How to Test Your Water Heater's Temperature & Pressure Valves | Roto-Rooter
Frictional pressure drop in fluidized bed- fluid-solid system
Комментарии
 0:05:45
0:05:45
 0:05:16
0:05:16
 0:12:42
0:12:42
 0:02:46
0:02:46
 0:00:31
0:00:31
 0:09:04
0:09:04
 0:01:32
0:01:32
 0:06:43
0:06:43
 0:18:02
0:18:02
 0:02:56
0:02:56
 0:06:56
0:06:56
 0:07:27
0:07:27
 0:01:43
0:01:43
 0:03:05
0:03:05
 0:02:30
0:02:30
 0:02:58
0:02:58
 0:03:31
0:03:31
 0:05:03
0:05:03
 0:17:21
0:17:21
 0:10:01
0:10:01
 0:07:40
0:07:40
 0:04:27
0:04:27
 0:03:01
0:03:01
 1:00:52
1:00:52