filmov
tv
Cosets -- Abstract Algebra video 9

Показать описание
⭐Support the channel⭐
⭐my other channels⭐
⭐My Links⭐
⭐my other channels⭐
⭐My Links⭐
Cosets in Group Theory | Abstract Algebra
(Abstract Algebra 1) Definition of Cosets
Abstract Algebra - 7.1 Cosets and Their Properties
Abstract Algebra 36: Cosets
Cosets -- Abstract Algebra video 9
Cosets - 12 - A First Course in Abstract Algebra
Abstract Algebra | Coset equality.
Coset example
Proof: Cosets Partition the Group | Abstract Algebra
Abstract Algebra. Cosets and their properties
Cosets (Abstract Algebra)
Abstract Algebra 38: Properties of cosets
Abstract Algebra 37: Cosets drawn via a picture
Two Properties of Cosets | Abstract Algebra
Normal Subgroups and Quotient Groups (aka Factor Groups) - Abstract Algebra
Abstract Algebra Lectures Part 4: Cosets
Abstract Algebra. Lagrange's Theorem and cosets
Abstract Algebra 7.1: Coset Definitions
Proof: Cosets are Disjoint and Equal Size
Group Theory | Cosets | Cosets Examples | Abstract Algebra
Abstract Algebra | Cosets of a subgroup.
Cosets | Left-Right Cosets|Algebraic Structures | Module 5 (Part 11) MAT 203-DMS S3(2019) KTU BTech
Chapter 3: Lagrange's theorem, Subgroups and Cosets | Essence of Group Theory
Group Theory | Cosets | Lagrange Theorem Group Theory | Abstract Algebra
Комментарии
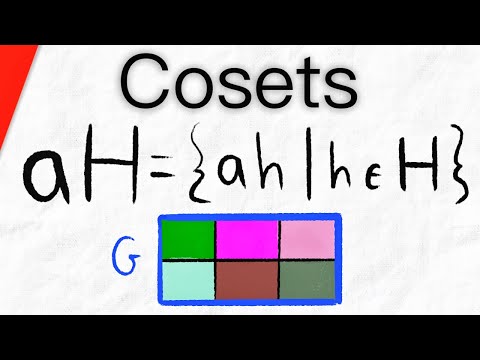 0:15:26
0:15:26
 0:16:56
0:16:56
 0:15:21
0:15:21
 0:05:25
0:05:25
 0:37:24
0:37:24
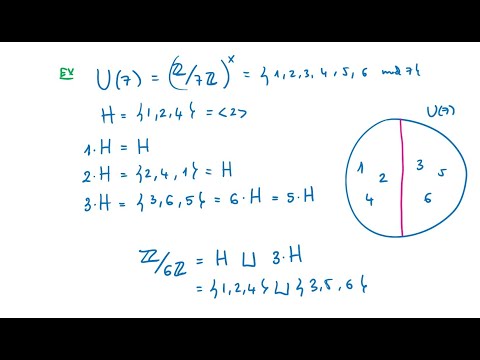 0:40:44
0:40:44
 0:10:50
0:10:50
 0:04:54
0:04:54
 0:06:31
0:06:31
 0:22:03
0:22:03
 0:11:18
0:11:18
 0:07:20
0:07:20
 0:04:25
0:04:25
 0:05:18
0:05:18
 0:11:24
0:11:24
 0:25:36
0:25:36
 0:21:41
0:21:41
 0:08:06
0:08:06
 0:04:46
0:04:46
 0:21:29
0:21:29
 0:18:30
0:18:30
 0:15:55
0:15:55
 0:13:40
0:13:40
 0:18:41
0:18:41