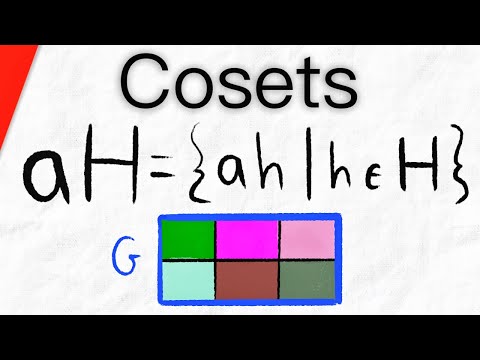
Cosets in Group Theory | Abstract Algebra

We introduce cosets of subgroups in groups, these are wonderful little discrete math structures, and we'll see coset examples and several coset theorems in this video. If H is a subgroup of a group G, and a is an element of G, then Ha is the set of all products ha where a is fixed and h ranges over H. Ha is called a left coset of H in G, and the right coset aH is defined similarly. We will see finite cosets and infinite cosets. #abstractalgebra #grouptheory

Join Wrath of Math to get exclusive videos, lecture notes, and more:

★DONATE★

Outro music by Ben Watts and is available for channel members.

Follow Wrath of Math on...

Cosets of a subgroup partition the whole group, and cosets with common elements are equal, we discuss both of these facts in this video, and we prove the latter fact. Our discussions of cosets will eventually lead to a proof of a famous result: Lagrange's Theorem.

[DrUBashir]

Refreshing to see a lecture the length of the intro to most profs' 95 minute long rants on youtube. You conmunicated your message in less than what most people spend writing things out on a board. bravo

[nataliarobinson]

This was clear, easy to understand and concise without leaving things out. It was perfect. Thank you!

[sanguiniusthegreat]

Intro to Abstract was probably my favourite class in my undergrad studies.

[ThefamousMrcroissant]

Very coherent. This is a great format for going over definitions. Since you clearly scripted it as well the video was pleasant to listen to (a lot of similar videos tend to "improvise" on the fly, but that doesn't work as neatly from my experience and is fairly error prone).

Excellent video!

[lesorogolfrancisco]

Greatly appreciate your work and quite comprehensive explanations. Very brief and straightforward thank you!

[MyOneFiftiethOfADollar]

Glad to have you back in Richard Rusczyk Hoodie form!

I liked your morning coffee presentation style as well.

The result from abstract algebra that is most memorable to me is the shockingly easy proof that every group is isomorphic to a permutation group(operation composition)

[slowsatsuma]

Amazing video. I was being thought about this using equivalence relations but this is far far more intuitive and now I actually understand what’s going on instead of just regurgitating formulas!

[MrCoreyTexas]

Thanks for this video, I was watching another series and got stuck on cosets. The examples helped big time!

[dananifadov]

thank you so much for everything that you do!
I've been watching your videos since the beginning of my studies with calculus 1, and I always find myself so relieved when a course I'm doing has a relevant playlist/video in your channel (:
your channel is a place to find sense and great explanations for me when everything is spiraling. so thanks a lot, and hopefully for many more encounters here in my next courses! (:

[aashsyed]

Not only is this video really great, it is coset great .

[chimetimepaprika]

Thank you. The motivation was enigmatic and confusing to me in the beginning.

[tanajkamheangpatiyooth]

Great introduction to group theory. Please keep on good work !

[bangvu]

Thanks for the short but sharp explanation. Was struggling to understand cosets while reading the textbooks.

[athenaheke]

Bless your heart, I finally understood, just in time for exams. I hope I make it this time 😤

[expert-wal]

wow wow wow!!! great job sir. very easy and simple to understand.

[Sarah-puun]

Thank you so much! Greetings from Germany

[MiM.]

Can you please prove that Ha = {x€G: x is congruent to b mod H}

[utsavkumar]

your eyes are too good and your explanation too. And hello, I am from India.

[suneptoshiozukum]

Thanks for your help man
Been struggling with it ❤

[Amedzz]

In this example 2:50, shouldn't you write the classes of the elements? i.e. Z4 = { [0], [1], ... }