filmov
tv
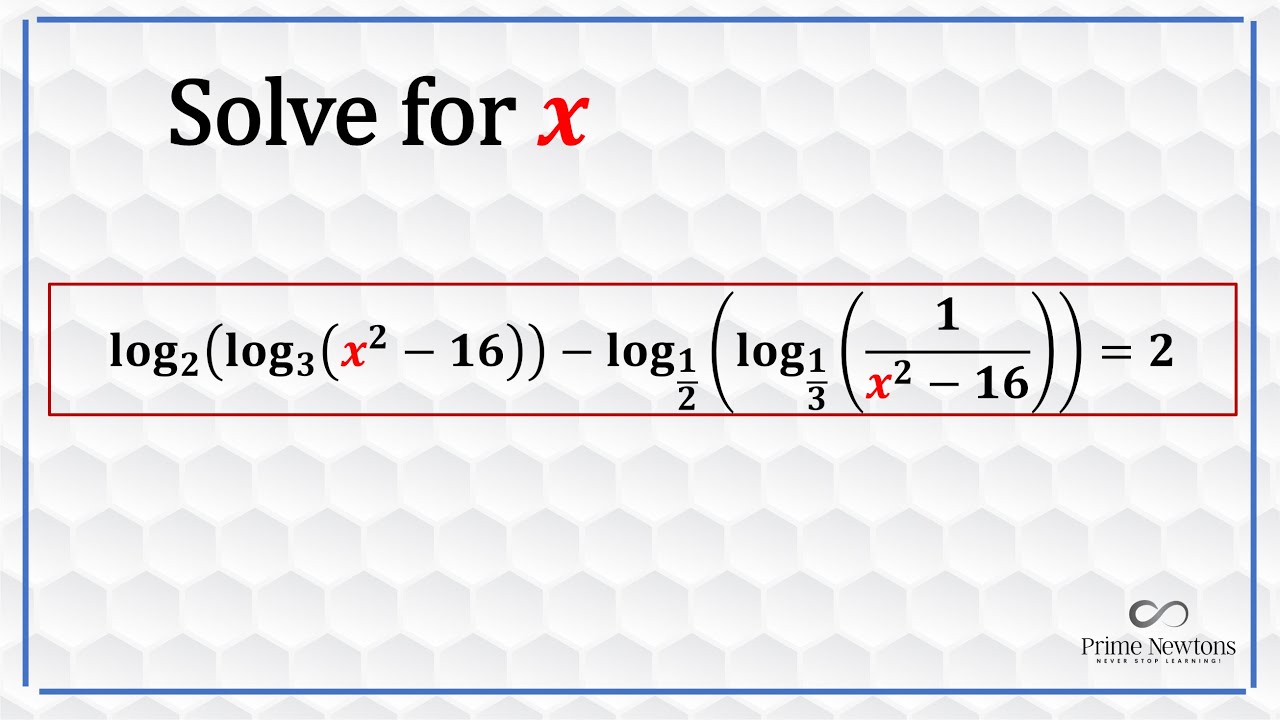
Solving a complicated logarithmic equation
Показать описание
In this video, I showed how to solve a complicated logarithmic equation using basic rules of logarithm
Solving Logarithmic Equations
Solving (Challenging) Log Equations Different Bases
Solving Complex Logarithmic Equations
solving a logarithmic equation with different bases
Solving Exponential and Logarithmic Equations
Solving Complex Logarithmic Equations
solving a logarithmic equation with different bases
Complex Logarithmic Equations
China Math Olympiad Problem. Find x!!
Solving logarithmic equations | Exponential and logarithmic functions | Algebra II | Khan Academy
Solving Log Equation
Solving a logarithmic equation
Solving a hard logarithmic equation with different bases
How to Solve Advanced Logarithmic Equations: Step-by-Step Tutorial
Solving A Quick and Easy Logarithmic Equation
Solving An Interesting Log Equation | Math Olympiads
Simple Logarithmic Equation
SOLVING LOGARITHMIC EQUATIONS || FINDING THE VALUE OF X
Logarithmic Equations
Solving a logarithmic equation when the bases are different. An algebraic challenge.
How to Solve Challenging Logarithmic Equations: Step-by-Step Explanation
Does this logarithmic equation have a solution? #algebra2 #algebra #mathproblems #mathematics
Solving a Logarithmic Exponential Equation
Solve a Logarithmic Equation
Комментарии
 0:25:27
0:25:27
 0:02:50
0:02:50
 0:10:42
0:10:42
 0:06:02
0:06:02
 0:07:08
0:07:08
 0:04:59
0:04:59
 0:04:10
0:04:10
 0:23:54
0:23:54
 0:03:22
0:03:22
 0:04:13
0:04:13
 0:00:55
0:00:55
 0:05:13
0:05:13
 0:02:19
0:02:19
 0:06:05
0:06:05
 0:09:26
0:09:26
 0:09:47
0:09:47
 0:00:33
0:00:33
 0:14:27
0:14:27
 0:12:59
0:12:59
 0:05:06
0:05:06
 0:09:51
0:09:51
 0:00:47
0:00:47
 0:04:54
0:04:54
 0:00:58
0:00:58