filmov
tv
What is convolution?

Показать описание
What is convolution?
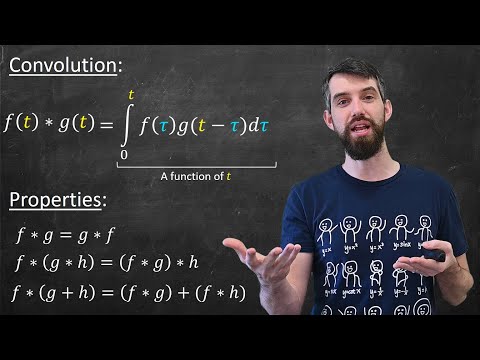
Here is the concept of convolution, which is the correct way of multiplying two functions. Not in an algebra way but in an analysis way. We also state the convolution theorem, which says that the laplace transform of f * g is the product of laplace transforms. This is useful to find inverse Laplace transforms as well. I also show a cool demo illustrating why there is a shift and an integral in the definition.
Here is the concept of convolution, which is the correct way of multiplying two functions. Not in an algebra way but in an analysis way. We also state the convolution theorem, which says that the laplace transform of f * g is the product of laplace transforms. This is useful to find inverse Laplace transforms as well. I also show a cool demo illustrating why there is a shift and an integral in the definition.
But what is a convolution?
What is convolution? This is the easiest way to understand
How to Understand Convolution ('This is an incredible explanation')
What is Convolution and Why it Matters
What is Convolution
The Convolution of Two Functions | Definition & Properties
What are Convolutional Neural Networks (CNNs)?
What is Convolution? And Two Examples where it arises
Convolution and the Fourier Transform explained visually
Tutorial 21- What is Convolution operation in CNN?
Convolutions are not Convoluted
A simple image convolution
Convolution and Unit Impulse Response
2D Convolution Explained: Fundamental Operation in Computer Vision
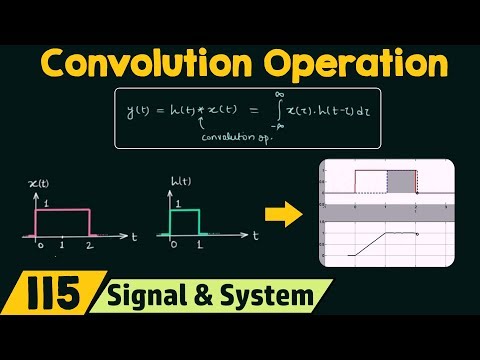
Introduction to Convolution Operation
Convolutions | Why X+Y in probability is a beautiful mess
Convolution in 5 Easy Steps
What is...convolution?
What is convolution?
Convolutional Neural Networks Explained (CNN Visualized)
🤖Convolutional Neural Networks (CNNs) by #andrewtate and #donaldtrump
Convolutional Neural Networks (CNNs) explained
Simple explanation of convolutional neural network | Deep Learning Tutorial 23 (Tensorflow & Pyt...
CNN: Convolutional Neural Networks Explained - Computerphile
Комментарии
 0:23:01
0:23:01
 0:05:36
0:05:36
 0:05:23
0:05:23
 0:09:59
0:09:59
 0:00:55
0:00:55
 0:10:33
0:10:33
 0:06:21
0:06:21
 0:15:48
0:15:48
 0:07:55
0:07:55
 0:10:58
0:10:58
 0:10:28
0:10:28
 0:00:59
0:00:59
 0:09:22
0:09:22
 0:05:06
0:05:06
 0:30:42
0:30:42
 0:27:25
0:27:25
 0:14:02
0:14:02
 0:09:30
0:09:30
 0:12:21
0:12:21
 0:10:47
0:10:47
 0:00:36
0:00:36
 0:08:37
0:08:37
 0:23:54
0:23:54
 0:14:17
0:14:17