filmov
tv
Proof that 0 = 1 using Infinite Series - Can you Find the Mistake?

Показать описание
Proof that 0 = 1 using Infinite Series - Can you Find the Mistake?
Proof that 0 = 1
Why is 0! = 1?
Prove that Zero Factorial is Equal to One
Why 0! = 1 ? | Why 0 factorial is equal to 1? #shorts
0.999999… = 1
why 0!=1 in 30 Seconds. (Shortest 0 factorial proof) #shorts
Prove that 0=1 || How To Proof 0=1
Why is 0! = 1 (Proof)
The Most Controversial Number in Math
A Proof That 0=1 ( Can You Find The Mistake )!
Why does 'x to the zero power' equal 1?
'PROVE' 0 = 1 Using Integral Calculus - Where Is The Mistake?
Why is x^0 = 1 (Quick Proof)
0!=1 PROOF | Zero Factorial is Equal to One
But Why Does 0 Factorial Equal 1
Why is any number multiplied by zero 0 ❓ A mathematical proof using the properties of real numbers....
🧠 Can You Spot the Mistake? Fun Math Puzzle: Does 0 = 1? 🎉
Super Simple Maths Trick to Prove 1 = 0
Zero Factorial - Numberphile
Proof that 1 = 2.
Why do numbers to the power of 0 equal 1?
What is 0 to the power of 0?
I Finally Found Out What 0/0 Should Be
Every PROOF you've seen that .999... = 1 is WRONG
Комментарии
 0:02:59
0:02:59
 0:06:05
0:06:05
 0:01:58
0:01:58
 0:01:00
0:01:00
 0:01:00
0:01:00
 0:00:30
0:00:30
 0:02:48
0:02:48
 0:02:25
0:02:25
 0:06:46
0:06:46
 0:02:53
0:02:53
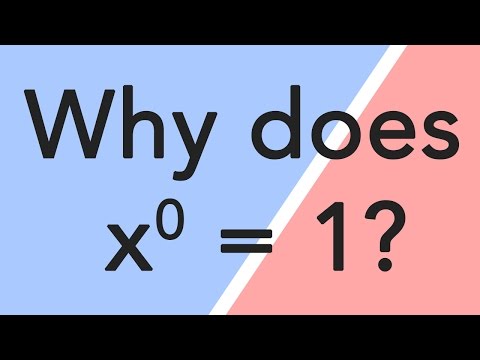 0:02:15
0:02:15
 0:04:58
0:04:58
 0:01:09
0:01:09
 0:03:30
0:03:30
 0:04:49
0:04:49
 0:03:06
0:03:06
 0:03:33
0:03:33
 0:09:40
0:09:40
 0:07:36
0:07:36
 0:02:59
0:02:59
 0:05:58
0:05:58
 0:03:25
0:03:25
 0:04:40
0:04:40
 0:09:25
0:09:25