filmov
tv
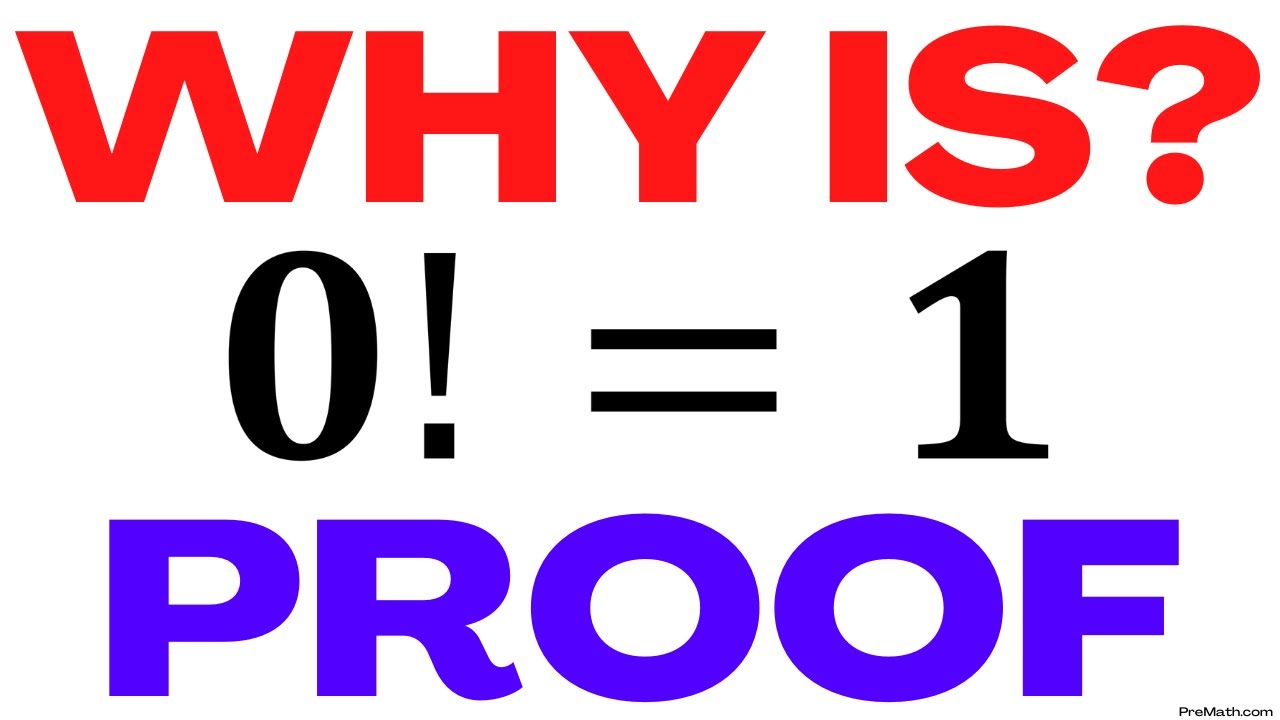
0!=1 PROOF | Zero Factorial is Equal to One
Показать описание
0!=1 PROOF | Zero Factorial is Equal to One
Proof that zero factorial equals one (0!=1)
Prove that Zero Factorial is Equal to One
Why is 0! = 1?
Why is 0! = 1? Zero Factorial
Zero Factorial - Numberphile
zero factorial, why 0! should be 1, 4 reasons
How Zero factorial equals one (0! = 1) Proof
MATHS LAGERAHO || BINOMIAL THEOREM || 5 MARKS || CLASS 11TH
But Why Does 0 Factorial Equal 1
Catalyst University Math: Simple Proof That Zero Factorial (0!) Equals 1
Why is 0! = 1 (Proof)
Zero Factorial: Why is 0! = 1?
why 0!=1 in 30 Seconds. (Shortest 0 factorial proof) #shorts
Proof: Zero Factorial Equals One
Why 0! = 1 ? | Why 0 factorial is equal to 1? #shorts
0! = 1 | Proof of factorial zero
Math - What is Zero Factorial?
why 0! =1 | proof why zero factorial equals to 1
0 ! = 1 Proof | Why is 0 factorial equal to 1 proof | Zero factorial proof | 0 a | 0 1 | Purdue Math
Hence Proved #1 - How is zero factorial equal to one (0! = 1) ?
Why 0 factorial is equal to 1
Why is zero factorial equals one with Paul Proof, the Math Wizard. Why 0! =1.
Proof that 0 = 1
Комментарии
 0:03:30
0:03:30
 0:01:01
0:01:01
 0:01:58
0:01:58
 0:06:05
0:06:05
 0:05:14
0:05:14
 0:07:36
0:07:36
 0:12:58
0:12:58
 0:00:58
0:00:58
 0:37:09
0:37:09
 0:04:49
0:04:49
 0:03:19
0:03:19
 0:02:25
0:02:25
 0:04:07
0:04:07
 0:00:30
0:00:30
 0:00:42
0:00:42
 0:01:00
0:01:00
 0:00:31
0:00:31
 0:05:24
0:05:24
 0:00:14
0:00:14
 0:02:50
0:02:50
 0:04:44
0:04:44
 0:04:51
0:04:51
 0:05:54
0:05:54
 0:02:59
0:02:59