0.999999… = 1

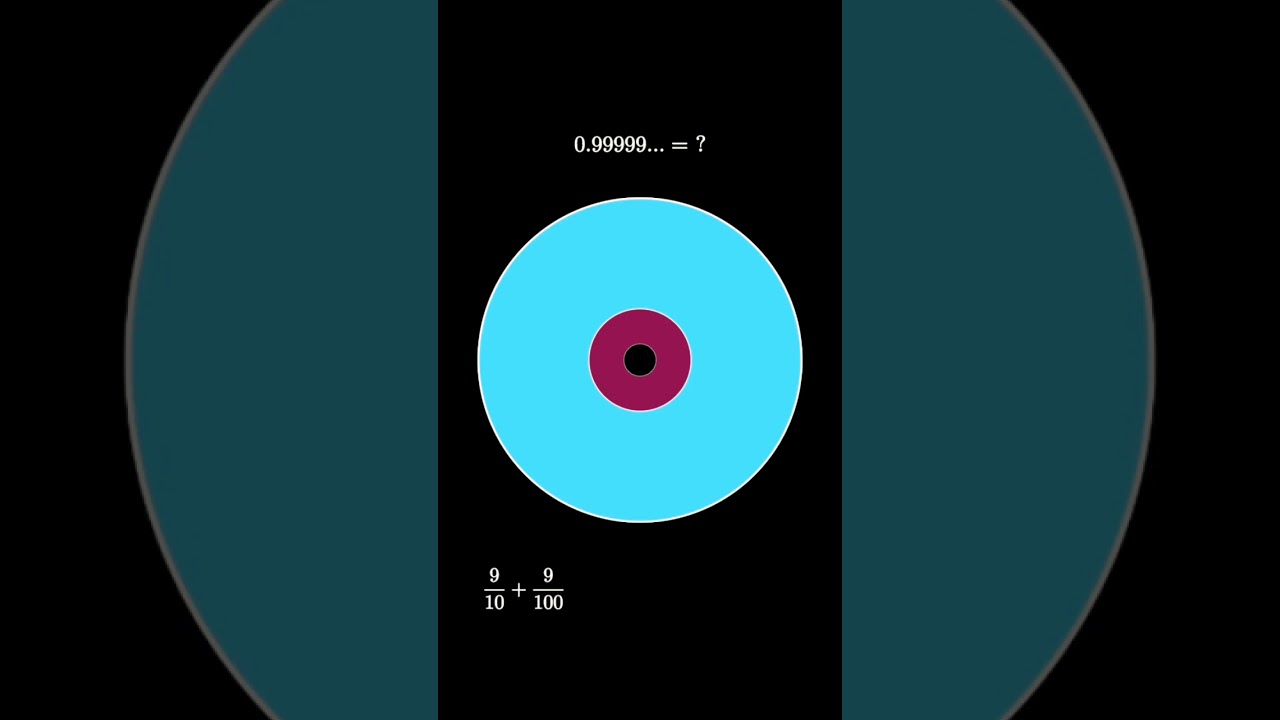
In this short, we use a geometric representation and the Archimedean principle to show why 0.99999... equals 1. This fact tends to be a bit controversial in the mathematics classroom, but the only way to make sense of an infinite sum is to treat it as a limit. Thus, we can use the term equality when discussing infinite sums like 0.999... and there is no number this sum could equal besides 1.

If you like this video, please click "like" and consider subscribing and checking out my other videos.

Thanks!

To see a longer video with a similar fact (and perhaps more justification at the end), see

Here is a playlist with other geometric sums dissection proofs:

#infiniteseries #paradox #manim #math #mathshorts #visualproof #proofwithoutwords

To learn more about animating with manim, check out:

[gabrielbarrantes]

To properly explain this you need to say that 0.9999... represents a limit.

[Astrobay]

Let's be real. People that understood this explanation didn't doubt the fact in the first place.

[sergioelsergio]

Here's another explanation, there's no number between 0.999... and 1, so they are the same number.

[upsidedown-pug]

this also connects to those visual proofs you did a little bit ago with things like
1/4 + 1/4^2 + 1/4^3... = 1/3
this setup basically does:
9/10 + 9/10^2 + 9/10^3... = 9/9 = 1

[MavikBow]

If I remember correctly, the way our calculus teacher explained this was that if two numbers are different, you can squeeze at least one more number in between them. He then proved that you cannot put any number between 1 and 0.(9), thus concluding they were the same.

[ny-ro]

The one I liked the most was
Let 0.999… = x
So 9.999… = 10x
Subtract x so 9 = 9x
And divide to find that 1 = x = 0.999…

[joshyoung]

This could still be contentious; I think the core problem is that infinity is really impossible for the human brain to work intuitively with, so it can be hard to realize that's what infinity does there. I still don't know why it's "controversial, " though. It's definitively true, a feature of the way numbers are written.

[paull]

If 0.9 repeating wasn’t 1, then 0.3 repeating wouldn’t be 1/3.

[MinhBui-hx]

The visualization is nice, but it doesn’t provide a compelling explanation I would way. It’s like trying to use one limit to elaborate another limit which is as controversial as the original one. I appreciate your effort in doing these videos, keep up the good work.

[cheesebusiness]

Kids, remember: involving infinity is a grey zone of math where cheating is possible

[shahanshahpolonium]

When you got the 0.9+0.09+0.009... you could have used the geometrical progression formula for the sum of infinite terms

a/(1-r) where a is the first term and r is the common ratio.
Clearly 0.9 is the first term and 0.1 is the common ratio.

Substituting, we get sum = 0.9/(1-0.1) = 0.9/0.9 = 1.

[celetial]

another way to think about it is that 1/9 =

multiple that by nine, and you get 9/9 = and 9/9 = 1, so 1 = .9999…

[ikwenmusic]

I like to think of how 1/9 is 0.111… and 2/9 is 0.222… and so on. 9/9 is 0.999… but a fraction with the same numerator and denominator is 1

[JJHJKM]

I love your method of explaining summations

[hyronvalkinson]

Exactly. The empty space is equal to the area of a point. That area is zero. Therefore 1 - 0 = 0.9999... and 1 is exactly equal to 0.9999...

[Sebastian-sltk]

My English teacher denied the fact that 38 divided by 4 is 9.5, thus being able to be rounded to 10. She stated that the result is and so on. She said that when putting it into the calculator, it would give that number. So, out of rage I divided by 4 and what do you know, it was 9.5. But in any case I "had" to get another grade in order to have an average of 10, while it was clear that I already had it.

[The_Human_Tripod]

My explanation was: if you were to represent 1/9 in decimal form, you get 0.11… multiply that by 9. The decimal representation would be 0.99… The actual fraction would be 9/9, or other words 1.

[sheshathri]

😮 what an explanation, i like your channel bro

[purplenanite]

1/2 +1/4 +1/8 +1/16 +...=1
people sleep

1/3 +1/9 +1/27 +1/81 +... =1/2
people sleep

1/4 +1/16 +1/64+... =1/3
people sleep


people sleep

9/10+9/100+9/1000+...=1
*sounds of angry crowds*

[enmingzhang]

We can also explain this using algebra.
if x =
then 10x =
and so 10x - x = -
therefore 9x = 9
which means x = 1