filmov
tv
Physics CH 0: General Introduction (9 of 20) Multiplying with Uncertainties in Measurements
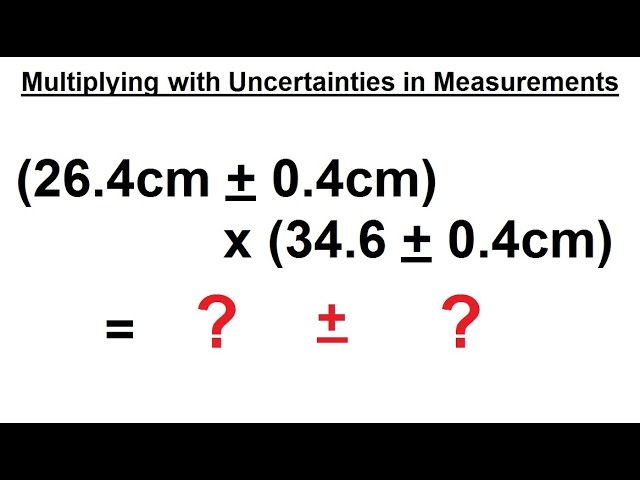
Показать описание
In this video I will multiply 2 lengths when there are uncertainties in the measured length.
Next video in the series can be seen at:
Physics CH 0: General Introduction (1 of 20) Standard SI for Length, Mass, and Time
Physics CH 0: General Introduction (5 of 20) Introduction to Uncertainty in Measurements
Physics CH 0: General Introduction (16 of 20) Understanding Coordinate Systems and Directions
Physics CH 0: General Introduction (18 of 20) Basic Trig for Physics - The Triangle (Sine)
Physics CH 0: General Introduction (6 of 20) Finding Area with Uncertainty in Measurements
Physics CH 0: General Introduction (9 of 20) Multiplying with Uncertainties in Measurements
Physics CH 0: General Introduction (13 of 20) How to Convert 1 Unit to Another Unit
Physics CH 0: General Introduction (10 of 20) Dividing with Uncertainties in Measurements
Condensed Matter Physics - Band Theory of Solids : An Introduction
Physics CH 0: General Introduction (17 of 20) Basic Trigonometry for Physics - The Triangle
Physics CH 0: General Introduction (20 of 20) Basic Trig for Physics - The Triangle (Tangent)
Physics CH 0: General Introduction (4 of 20) How to Determine Significant Figures in Operations
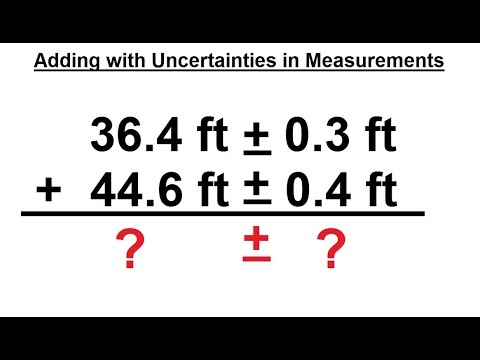
Physics CH 0: General Introduction (7 of 20) Adding with Uncertainties in Measurements
Physics CH 0: General Introduction (3 of 20) How to Determine Significant Figures
Physics CH 0: General Introduction (11 of 20) Uncertainties in Measurements - Squares and Roots
Physics CH 0: General Introduction (15 of 20) Estimate Number of Blades of Grass on Soccer Field
Physics CH 0: General Introduction (8 of 20) Subtracting with Uncertainties in Measurements
Physics CH 0: General Introduction (14 of 20) How to Estimate Number of Teachers in US
Physics CH 0: General Introduction (2 of 20) Dimensional Analysis (Unit Analysis)
Physics - Basic Introduction
Physics CH 0: General Introduction (12 of 20) How to Convert 'Feet' to 'Meters'
Physics CH 0: General Introduction (19 of 20) Basic Trig for Physics - The Triangle (Cosine)
Physics 101 - Chapter 1 - Physics and Measurements
Class 9 - Physics - Chapter 01 - Lecture 1 Introduction to Physics - Allied Schools
Комментарии
 0:04:56
0:04:56
 0:06:51
0:06:51
 0:02:23
0:02:23
 0:03:55
0:03:55
 0:05:44
0:05:44
 0:04:39
0:04:39
 0:07:11
0:07:11
 0:03:30
0:03:30
 0:32:48
0:32:48
 0:05:49
0:05:49
 0:03:02
0:03:02
 0:08:35
0:08:35
 0:00:58
0:00:58
 0:05:55
0:05:55
 0:04:24
0:04:24
 0:02:47
0:02:47
 0:02:26
0:02:26
 0:03:15
0:03:15
 0:07:57
0:07:57
 0:53:37
0:53:37
 0:04:26
0:04:26
 0:02:07
0:02:07
 0:38:05
0:38:05
 0:13:41
0:13:41