How to find arc length and sector area in radians

Geometry Teachers Never Spend Time Trying to Find Materials for Your Lessons Again!

Join Our Geometry Teacher Community Today!

Finding arc length and sector area using radians
0:00 Review of converting degrees to radians and radians to degrees
0:43 200 degrees is how many radians
0:55 315 degrees is how many radians?
2:05 Radian measure of 3Pi/2 is equal to how many degrees?
2:25 Radian measure 2Pi/15 is how many degrees?
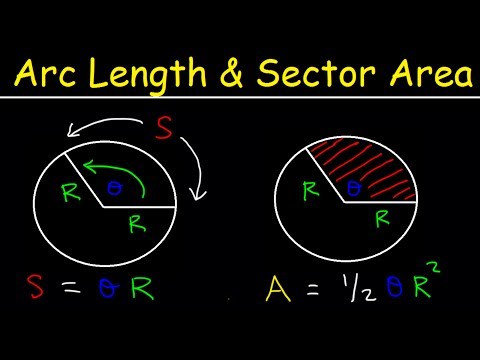
3:00 Review of the arc length formulas
3:32 360 degrees = _____ radians
4:12 Review of formula for finding arc length
4:28 Problem 1 Find the length of the intercepted arc of a circle with radius 12 and arc length in radians of 2Pi/3.
5:00 Problem 2 Find the length of the intercepted arc of a circle with radius 9 and arc length in radians of 11Pi/12.
5:55 Find the central angle in radians
6:32 Find central angle of a circle with radius 100 and arc length is 310.
7:06 Finding sector area in degrees
8:00 Find sector area of a circle with radius of 12 and central angle measure of 2pi/3.
8:20 Find sector area of a circle with a radius of 9inches and central angle of 11pi/12
10:40 Find the radius of a circle.
11:10 Find the radius of a circle with a arc length of 150m^2 and a central angle measure of 3pi/4
13:10 A circle has a sector area of 175 inches^2 and a theta of 5pi/6. What is the radius?

* Subscribe

Let’s connect
MooMooMath
* Google+
* Pinterest
Twitter

-~-~~-~~~-~~-~-
Please watch: "Study Skills Teacher's Secret Guide to your Best Grades"
-~-~~-~~~-~~-~-

[ArturoGarzaID]

Why didn't you use pi in the second examples that you did? You said you don't always have to use a pi. Can you explain? Could you have used one anyways? That kind of threw me off.

[rahulparmar]

Q: find area of segment if r=5 arc length =3.5,
Book says in solution ans is (r*s) 1/2. I don't understand how did they got this expression?? Plz help madam

[mikeysuperboom]

315/5 its not 60, your calculator was right but you wrote down wrong value. Thats why people may get confused. The answer for the second warmup question is wrong. :D