How to find the arc length of a circle using the formula

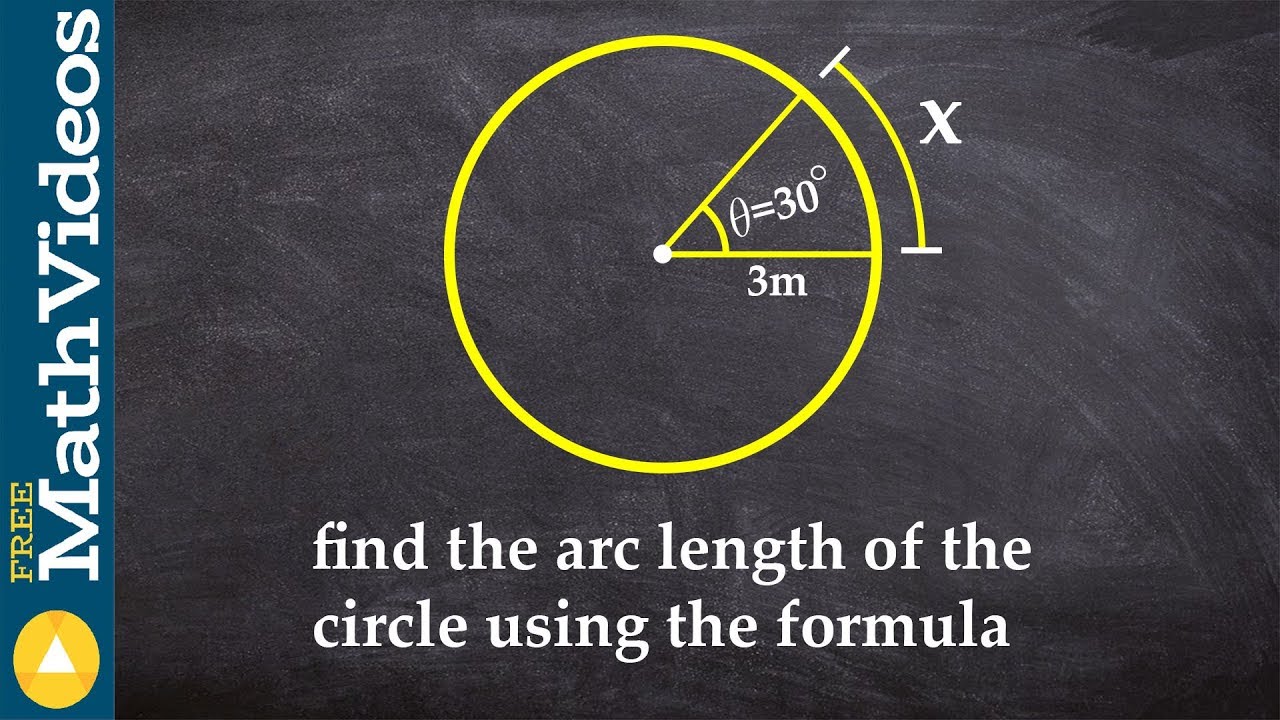
👉 Learn how to solve problems with arc lengths. You will learn how to find the arc length of a sector, the angle of a sector, or the radius of a circle. An arc of a circle is the curve between a pair of points on the circumference of the circle. The angle of an arc is the angle subtended by the arc with a pair of radii of the circle at the center of the circle. The portion enclosed by an arc of a circle and a pair of radii of the circle is called a sector.

The relationship between the arc length (S), the radius (r), and the angle subtended by the arc at the center (theta), is given by the formula S = r(theta), when theta is in radians. And is given by ((theta)/360) x 2pi r, when theta is in degrees.

Organized Videos:
✅ Angles in Trigonometry
✅ Solve Problems with Arc Length
✅ Angles in Trigonometry | Learn about
✅ Sketch Angles in Standard Position
✅ Find the Quadrant of the Angle
✅ Find the Reference Angle
✅ Complement and Supplement of an Angle
✅ Convert Radians to Degrees
✅ Convert Degrees to Radians
✅ Convert Degrees to Degree Minute Seconds
✅ Convert Degree Minute Seconds to Degrees
✅ Coterminal Angles | Learn About
✅ Find Coterminal Angles | 0 and 2pi
✅ Find Coterminal Angles | 0 and 360

Connect with me:

#trigonometry #brianmclogan

[literallykawaii]

Hes helping all of us on quarantine, thank u kind sir

[JuanTonSoupXP]

There’s a special place in hell for math










The day I posted this comment, the end of my senior year was approaching and I was struggling in calculus majorly. I was stuck doing a homework assignment, when I posted that comment I was crying from the stress. 😭😂

[emmalorraine]

He does so well at explaining things and breaking things down for an easier understanding so everyone can understand it. I love the way he teaches, truly a one of a kind teacher !!

[elijahbrents]

Defiantly the best math teacher on YouTube!

[faysal]

Late night grind session made much easier thanks to you

[GIANNIE.]

bro is really out here singlehandedly saving my gpa 🙏🙏

[betrey]

Why do I see him everytime a day before the test?

[michaelbadillo]

I JUST emailed my professor a question about this. Now it looks so easy!!!

[Alidotty]

1:19 every class someone have to say : Wait .. what " at EVERY SINGLE POINT

[candycastle]

Thank you thank you thank you! I couldn't solve these questions to save my life. This helped a whole lot

[stephanyflores]

2πr(m°/360°) you Can also use that formula and find the arc of a circle. Try it and it will give you the same.

[bruhduh]

You made this a lot easier for me! Thanks!

[kroebb]

took a test today and failed. wish i found you before that.
thank you kind sir

[gyubear]

hiii i just wanna thank u sir for making me understand better our lessons in mathematics, may it be calculus or algebra. thank u 3000 <3

[gabbyhuberty]

Im in the middle of my math final rn I needed this 😭

[kimmyranged]

When do we round to 1 decimal place? When they ask us to or the answer should always be rounded to one decimal place?

[maulmemes]

This is fantastic to have for when I have no idea what I'm doing. I must ask though, how do you do this backward, where instead of looking for S, you have S and r but you're looking for theta?

[JoséAntonioBottino]

Many people wonder why radians do not appear when we have radians*meters (rad • m) . Here is an attempt at an explanation:

Let s denote the length of an arc of a circle whose radius measures r.

If the arc subtends an angle measuring β = n°, we can pose a rule of three:
360° 2 • 𝜋 • r
n° s

Then
s = (n° / 360°) • 2 • 𝜋 • r

If β = 180° (which means that n = 180, the number of degrees), then
s = (180° / 360°) • 2 • 𝜋 • r

The units "degrees" cancel out and the result is
s = (1 / 2) • 2 • 𝜋 • r

that is, half of the circumference 2 • 𝜋 • r
s = 𝜋 • r

If the arc subtends an angle measuring β = θ rad, we can pose a rule of three:
2 • 𝜋 rad 2 • 𝜋 • r
θ rad s

Then
s = (θ rad / 2 • 𝜋 rad) • 2 • 𝜋 • r

If β = 𝜋 rad (which means that θ = 𝜋, the number of radians), then
s = (𝜋 rad / 2 • 𝜋 rad) • 2 • 𝜋 • r

The units "radians" cancel out and the result is
s = (1 / 2) • 2 • 𝜋 • r

that is, half of the circumference 2 • 𝜋 • r
s = 𝜋 • r

If we take the formula with the angles measured in radians, we can simplify
s = (θ rad / 2 • 𝜋 rad) • 2 • 𝜋 • r
s = θ • r

where θ denotes the number of radians (it does not have the unit "rad").
θ = β / (1 rad)

and θ is a dimensionless variable [rad/rad = 1].

However, many consider θ to denote the measure of the angle and for the example believe that
θ = 𝜋 rad

and radians*meter results in meters
rad • m = m

Mathematics and Physics textbooks state that
s = θ • r

and then
θ = s / r

It seems that this formula led to the error of believing that
1 rad = 1 m/m = 1

and that the radian is a dimensionless derived unit as it appears in the International System of Units (SI), when in reality
θ = 1 m/m = 1

and knowing θ the angle measures β = 1 rad.

In the formula
s = θ • r

the variable θ is a dimensionless variable, it is a number without units, it is the number of radians.

When confusing what θ represents in the formula, some mistakes are made in Physics in the units of certain quantities, such as angular speed.
My guess is that actually the angular speed ω is not measured in rad/s but in
(rad/rad)/s = 1/s = s^(-1).

[JoseRios-yfih]

Sup Brian! Me and niece marina are back at it studying for her final exam. Thanks for the vid!

[ricardodixon]

Good day professor. Does the 30 represent And inscribe angle from the Arc or The central angle of the Arc?